1998 AHSME Problem 3

Number Theory Level 1

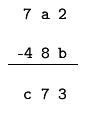If a, b, and c are digits for which the above is true, then a+b+c =

14 16 15 17

Daniel Hirschberg by Daniel Hirschberg , 39, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

3 solutions

Flip the order

alt text alt text

Then we easily see that B = 9, the one carries over so A = 6, the one carries over again so C= 2.

Therefore, we have a + b + c = 17 a + b + c = \boxed{17}

11 Helpful 0 Interesting 0 Brilliant 0 Confused

762-489=273 SO A+B+C=17

Mudassar Ijaz - 7 years, 2 months ago
Deepthi Prakash
Apr 22, 2014

JUST GUESSED

1 Helpful 0 Interesting 0 Brilliant 0 Confused
Shreyas Shastry
Mar 22, 2014

trial and error method

762-489=273 so a=6,b=9 and c=2

so a+b+c=17

1 Helpful 0 Interesting 0 Brilliant 0 Confused

17

Vicky Jangid - 7 years, 1 month ago

I'm getting dumber

Jade Sy - 4 years, 5 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...