200 Followers Problem!
1 2 + ( 1 2 + 2 2 ) + ( 1 2 + 2 2 + 3 2 ) + … + ( 1 2 + … + 1 9 9 2 + 2 0 0 2 ) = ?
The answer is 136016700.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
are you a sigma lover?
Two equivalent ways to obtain the result:
E x p r e s s i o n = 2 0 0 ∗ 1 2 + 1 9 9 ∗ 2 2 + . . . + 1 ∗ 2 0 0 2 , = i = 1 ∑ n ( 2 0 1 − i ) ∗ i 2 = i = 1 ∑ n ( 2 1 0 ∗ i 2 − i 3 ) = 2 0 1 ∗ 6 n ∗ ( n + 1 ) ∗ ( 2 n + 1 ) − ( 2 n ∗ ( n + 1 ) ) 2 = 2 n ∗ ( n + 1 ) ∗ { 2 0 1 ∗ 3 2 n + 1 − 2 n ∗ ( n + 1 ) } B u t n = 2 0 0 , ∴ E x p r e s s i o n = 2 2 0 0 ∗ 2 0 1 ∗ { 3 4 0 1 ∗ 2 0 1 − 2 2 0 0 ∗ 2 0 1 } = 1 3 6 0 1 6 7 0 0 .
The basics
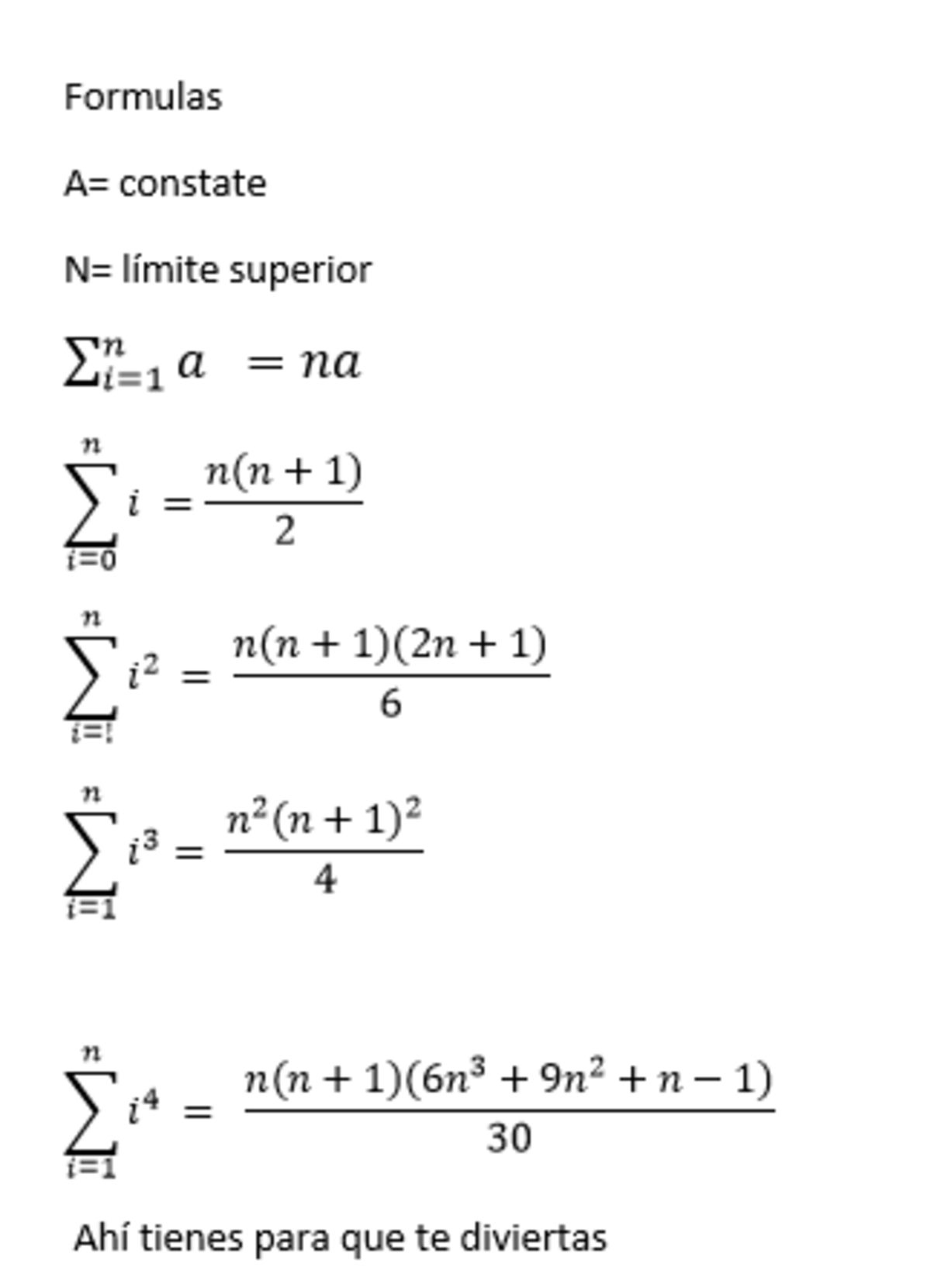
Then figure it out.
Sorry for the handmade
The above expression can be represented by n = 1 ∑ 2 0 0 k = 1 ∑ n k 2 .
And k = 1 ∑ n k 2 = 6 n ( n + 1 ) ( 2 n + 1 ) .
Thus,
n = 1 ∑ 2 0 0 k = 1 ∑ n k 2 = = = = = = = n = 1 ∑ 2 0 0 6 n ( n + 1 ) ( 2 n + 1 ) n = 1 ∑ 2 0 0 6 2 n 3 + 3 n 2 + n n = 1 ∑ 2 0 0 ( 3 1 n 3 + 2 1 n 2 + 6 1 n ) 3 1 n = 1 ∑ 2 0 0 n 3 + 2 1 n = 1 ∑ 2 0 0 n 2 + 6 1 n = 1 ∑ 2 0 0 n 3 1 ( 2 2 0 0 ( 2 0 1 ) ) 2 + 2 1 ( 6 2 0 0 ( 2 0 1 ) ( 4 0 1 ) ) + 6 1 ( 2 2 0 0 ( 2 0 1 ) ) 3 1 ( 4 0 4 0 1 0 0 0 0 ) + 2 1 ( 2 6 8 6 7 0 0 ) + 6 1 ( 2 0 1 0 0 ) 1 3 4 6 7 0 0 0 0 + 1 3 4 3 3 5 0 + 3 3 5 0 = 1 3 6 0 1 6 7 0 0