800 Followers problem!
1 0 0 2 + 9 9 2 − 9 8 2 − 9 7 2 + 9 6 2 + ⋯ + 4 2 + 3 2 − 2 2 − 1 2
Let N denote the number above. Find the remainder when N is divided by 1 0 0 0 .
The answer is 100.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
5 solutions
If we arrange all the numbers in a group of two as
(
1
0
0
2
-
9
8
2
)+(
9
9
2
-
9
7
2
)+..........(
4
2
-
2
2
)+(
3
2
-
1
2
)
=(100+98)(100-98)+(99+97)(99-97)+.............(4+2)(4-2)+(3+1)(3-1)
=2(100+98)+2(99+97)+...............2(4+2)+2(3+1)
=2(100+98+99+97+.............4+2+3+1)
=2(100+99+98+97+96+...........+5+4+3+2+1) [arranging them]
=2(5050)
=10100
Therefore remainder is 100 when divided by 1000
Consider 1st and 3rd term. 1 0 0 2 − 9 8 2 = 2 ∗ 1 9 8 , s o w e h a v e a n 2 − a n + 2 2 = 2 ∗ ( a n + a n + 2 ) S o i n a g r o u p o f f o u r w e h a v e a n 2 − a n + 2 2 + a n + 1 2 − a n + 3 2 = 2 ∗ { a n + a n + 2 + a n + 1 + a n + 3 } ∴ N = k = 1 ∑ 1 0 0 2 ∗ n k = 1 0 1 0 0 1 0 1 0 0 ≡ 1 0 0 ( m o d 1 0 0 0 )
Consider the first 4 terms
Let a = 100 , b = 99
Then , a - 2 = 98 b - 2 = 97
Therefore, the first four terms are in the form:
a^2 + b^2 - [ ( a - 2 )^2 + ( b - 2 )^2 ] = 4( a+b-2 )
Therefore, sum of first 4 terms is 4(100 + 99 - 2 ) = 788
Similarly, sum of next 4 terms is 756
Similarly, sum of last 4 terms is 20.
If we consider the sum of 4 consecutive terms as a single term, then we get a new AP, i.e.,
788, 756, .................,20
Here a =788, n = 100/4 =25 , l = 20
( where a = first term of AP , n = no. of terms and l = last term of AP )
Therefore, S = n/2 ( a+ l )
= 25/2 ( 788 + 20 )
S = 10100
Therefore, when S is divided by 1000, the remainder is 100.
Hence answer is 100
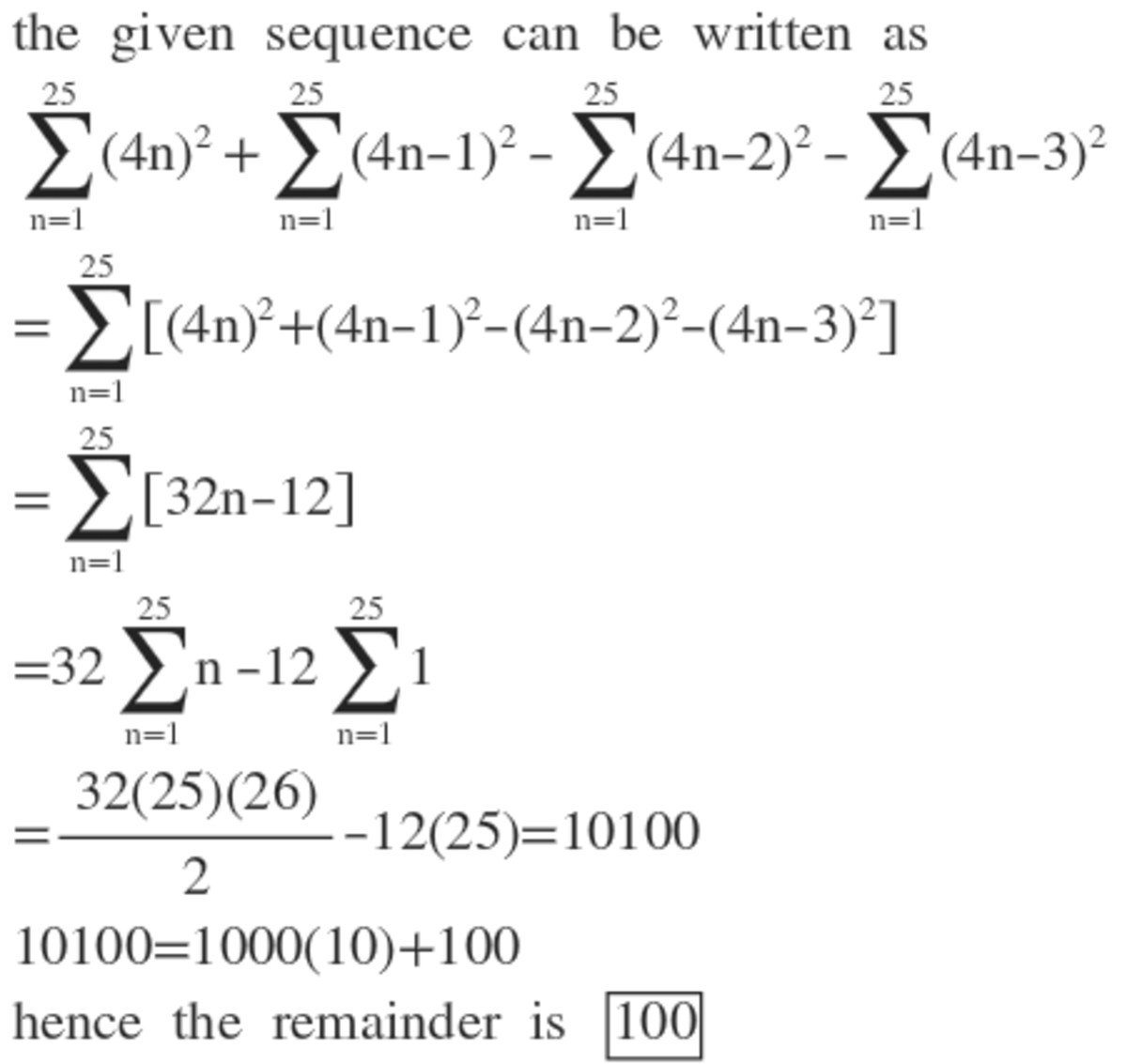
Lets do pairing as follows:
100²-1²=101x99
99²-2²=101x97
-98²+3²= -101x95
......& so on to get 50 pairs
N=101x(99+97-95-93+91+89.......+3+1)
First alternate + & - cancels to give a result 4. This goes on till 48 terms so we get 24x4=96
We add the last +3+1 to get 100
So N=101x100=10100 & hence remainder 100 when divided by 1000