A 5 × 5 pattern grid
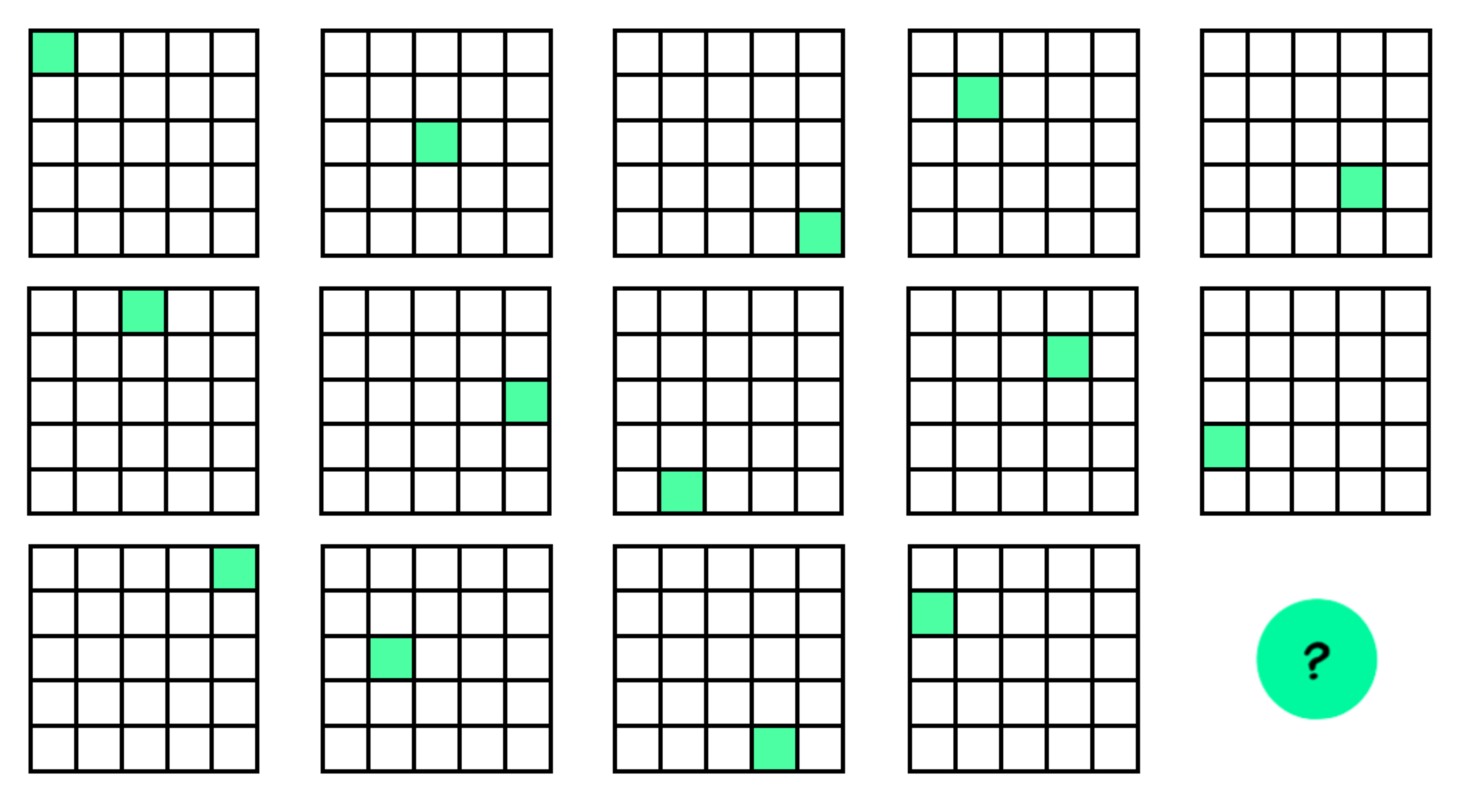
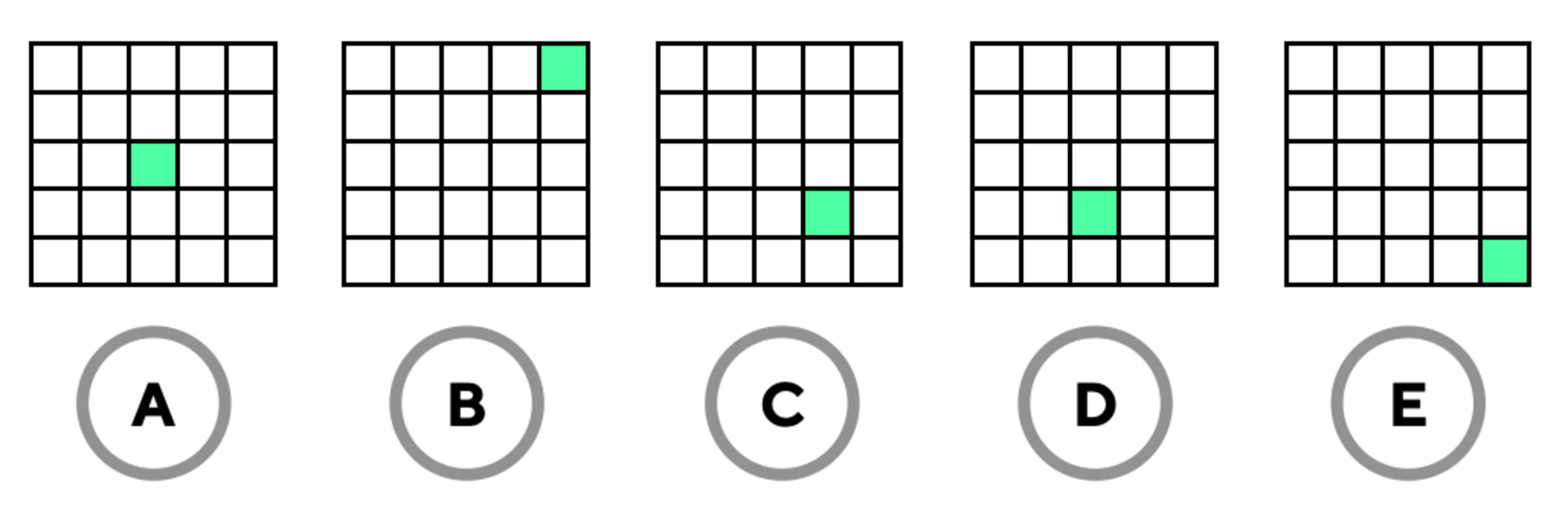
Which of these is the best fit to complete the pattern shown here?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
9 solutions
Such a mesmerizing and beautiful animation. Seems as if you have great skill at KeyNote. Keep it up and be BRILLIANT as always!
Log in to reply
Thank you for the kind comment @Hamza Anushath , glad you like the solutions!!
Fun problem to think about! Thanks!
This is exactly like sudoku, so much fun.
Much easy to solve if you see positions as coordinates Every time 2 is added to x and y coordinates.
Your solutions are great! Animated and can be understood!
How can this be "new"? BTW, I forgot to comment here at that time, nice problem!
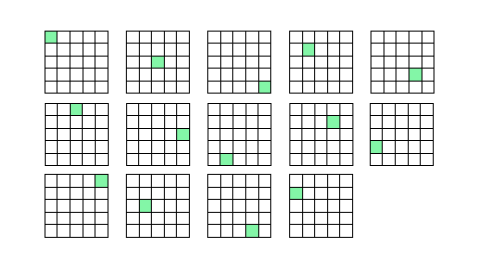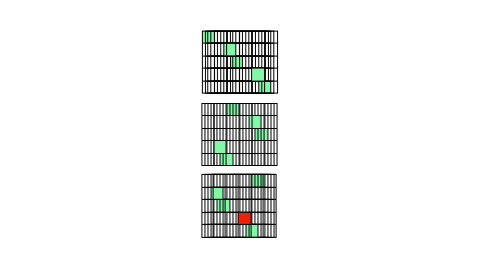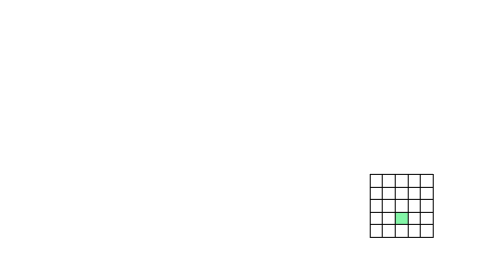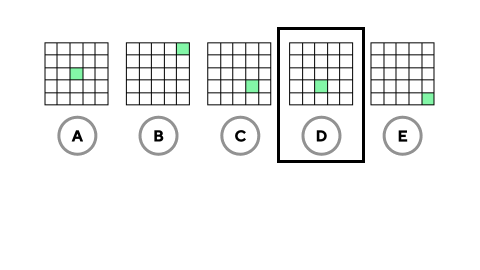
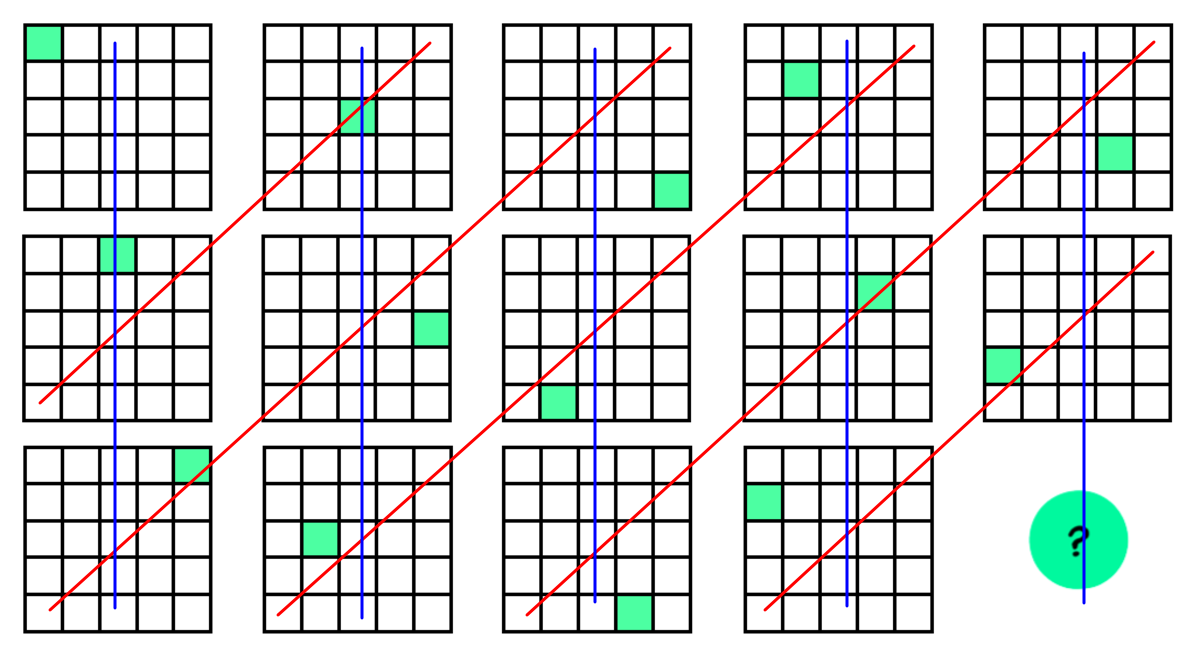 Every square connected with red lines has solution in the same column, with each square to the right having solution two rows lower than the last
Every square connected with red lines has solution in the same column, with each square to the right having solution two rows lower than the last
Every square connected with blue lines has solution in the same row, with each lower square having solution two columns to the right than the last.
You are really a brilliant sir
Of the solutions given, D was the only option that wasn't already included in the grid.
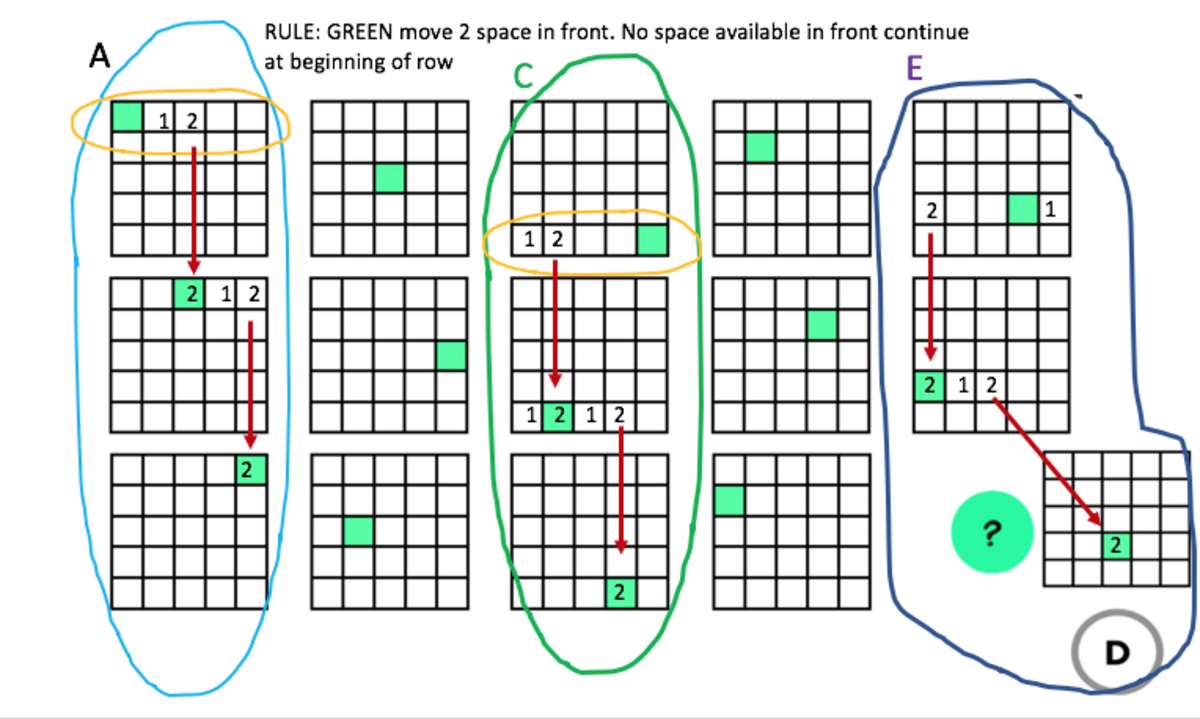
NOTE: Two columns are not done so it does not look too crowded.
It applies the same law as the exercise before. Each vertical column of 3 boxes is an independent unit but all of them obey the same law. The law is to move 2 spaces in front within your row, if there are no more boxes available in front then continue the counting from the first one at the beginning. In this case, there are 5 V.columns (Units) A, B, C, D, and E. Each unit has 3 boxes and all 3 work/play with the same row position. So when you go to the last Unit E, you apply the rule and move 2 spaces in front and land in the middlebox, on the second row from the bottom
Using the quinary operation, the difference in coordinates between adjacent points on the same row is the same.
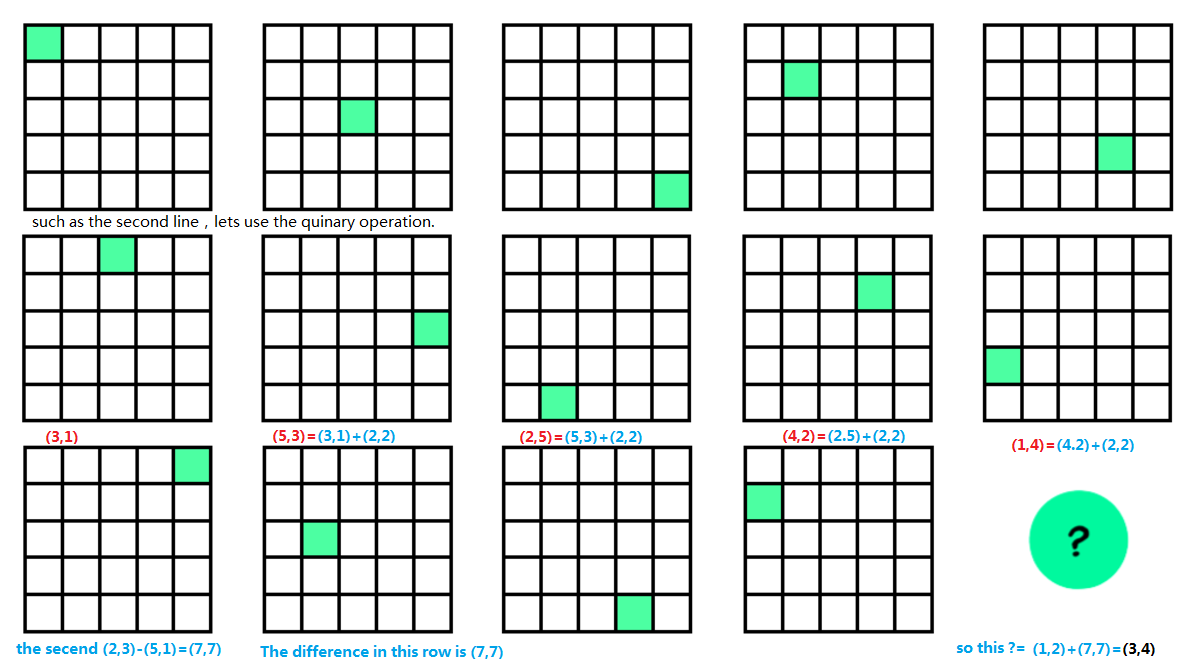
Looks interesting, can you elaborate what quinary operation is. Sorry I don't know about it. Thanks!
Log in to reply
I add a picture .I don't know if that is clear
This was pretty easy. you can see that all of the patterns happen on the same line if you look at the patterns vertically.
You can also see that none of the patterns repeat, so there you have the answer.
Honestly, I just draw it out and shade in the ones that are green then I see which one is the one that is missing a dot to complete the vertical line.
This one is harder than yesterday’s challenge but both are good problems, just saying.