Couldn't get any Fresher

From Euler Formula, we have $e^{-i x^{2}} = cos(x^{2}) - i sin(x^{2})$ So, $sin(x^{2}) = - Im(e^{-i x^{2}})$

Let, $I = \int_{0}^{\infty}\: e^{-i x^{2}} \:dx$ Now, $4I^{2} = \int_{-\infty}^{\infty}\:\int_{-\infty}^{\infty}\: e^{-i (x^{2}+y^{2})} \:dxdy = \int:\int\:e^{-i (x^{2}+y^{2})}:dA$ Convert into polar coordinate, $4I^{2} = \int_{0}^{\infty}\:\int_{0}^{2\pi}\:e^{-ir^{2}}\:r\:drd\theta = \frac{\pi}{i}$ Hence, we have, $I = \frac{1}{2}\sqrt{\frac{\pi}{i}}$ Since $\sqrt(i) = e^{i\pi/4}$ , then $I = \frac{1}{2}\sqrt{\frac{\pi}{2}}(1-i)$ So, we have $\int_{0}^{\infty}\:sin(x^{2})\: dx = -Im(I) = \frac{1}{2}\sqrt{\frac{\pi}{2}} = 0.627$

Let $x^2=t$ , the integral ( $I$ ) becomes:

$I=\int_{0}^{\infty} \frac{\sin t }{2\sqrt{t}} \text{d}t$

Now we use the fact that:

$\frac{1}{2\sqrt{t}}=\int_{0}^{\infty} \frac{1}{\sqrt{\pi}} e^{-s^2 t} \text{d}s$

Substituting this back in the original integral gives a double integral:

$I=\int_{0}^{\infty} \sin t \int_{0}^{\infty} \frac{1}{\sqrt{\pi}} e^{-s^2 t} \text{d}s \text{d}t$

or

$I=\frac{1}{\sqrt{\pi}} \int_{0}^{\infty} \int_{0}^{\infty} \sin t \ e^{-s^2 t} \text{d}t \text{d}s$

We know by laplace transforms that:

$\int_{0}^{\infty} \sin t \ e^{-s^2 t} \text{d}t=\frac{1}{1+s^4}$

Hence:

$I=\frac{1}{\sqrt{\pi}} \int_{0}^{\infty} \frac{1}{1+s^4} \text{d}s$

let $s=\frac{1}{r}$

$I=\frac{1}{\sqrt{\pi}} \int_{0}^{\infty} \frac{r^2}{1+r^4} \text{d}r=\frac{1}{\sqrt{\pi}} \int_{0}^{\infty} \frac{s^2}{1+s^4} \text{d}s$

so

$2I=\frac{1}{\sqrt{\pi}} \int_{0}^{\infty} \frac{1+s^2}{1+s^4} \text{d}s$

finally let $z=s-\frac{1}{s}$

$2I=\frac{1}{\sqrt{\pi}} \int_{-\infty}^{\infty} \frac{1}{2+z^2} \text{d}z=\frac{1}{\sqrt{\pi}} \frac{\sqrt{2}}{2} \arctan \left[ \frac{z}{\sqrt{2}} \right]_{-\infty}^{\infty}= \frac{1}{\sqrt{\pi}} \frac{\sqrt{2}}{2} \pi$

$I=\frac{1}{2} \frac{1}{\sqrt{\pi}} \frac{\sqrt{2}}{2} \pi=\frac{\sqrt{2\pi}}{4}=0.6266$

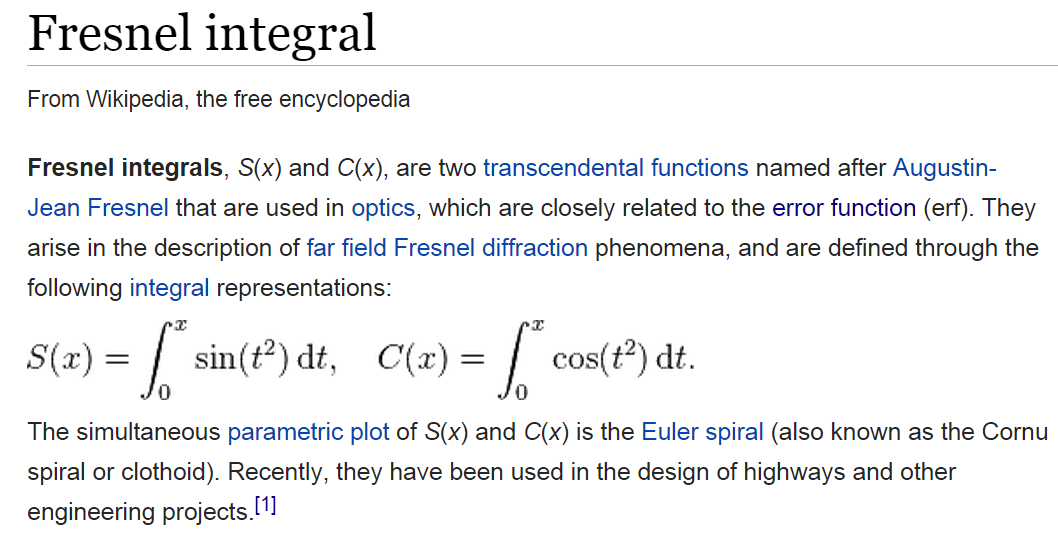
From the above definition, we have: $\int_{0}^{\infty}sin\space t^2dt = \lim \limits_{x \to \infty} S(x) = \sqrt{\pi \over 8}$

In addition, $\int_{0}^{\infty}cos\space t^2dt = \lim \limits_{x \to \infty} C(x) = \sqrt{\pi \over 8}$