Runs down a slide

Classical Mechanics Level 2

A boy runs down a slide height 4.0 m 4.0\text{ m} from home. Considering g = 10 m/s 2 g = 10\text{ m/s}^2 and 20% of the mechanical energy is dissipated during the trip, determine the speed at which the child hits the ground.


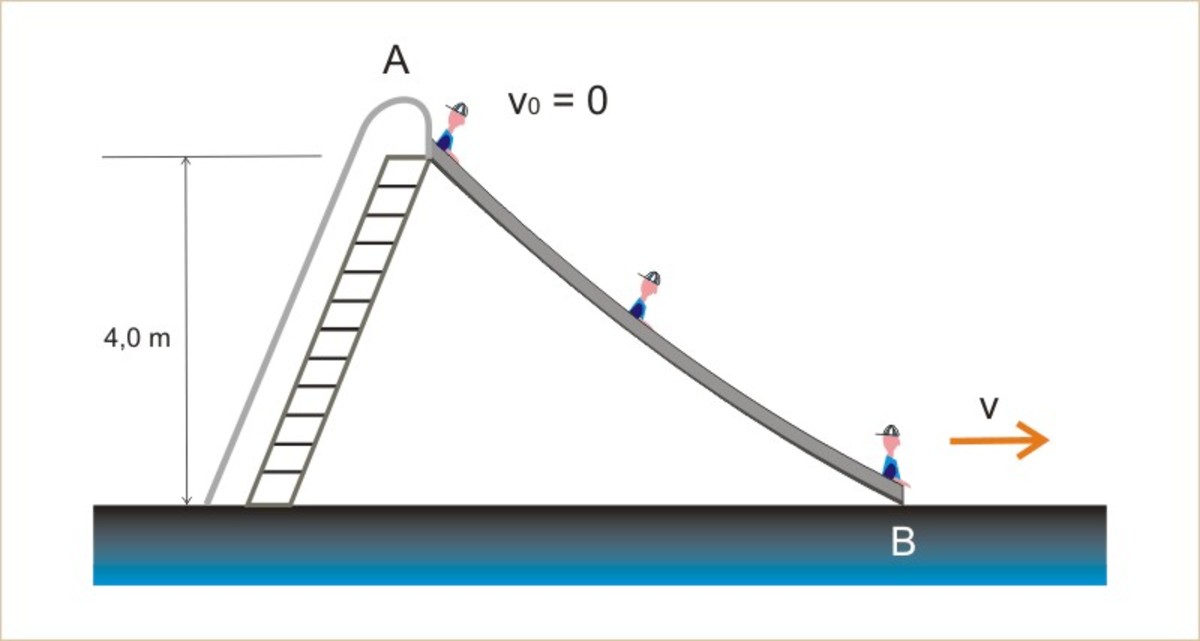
The answer is 8.

Lucas Sousa by Lucas Sousa , 26, Brazil

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Tom Engelsman
Sep 15, 2019

The required energy balance equation is just:

P E = K E + E f r i c t i o n ⇒ m g h = 1 2 m v 2 + 0.2 m g h ⇒ 0.8 m g h = 1 2 m v 2 ⇒ 1.6 g h = v PE = KE + E_{friction} \Rightarrow mgh = \frac{1}{2}mv^2 + 0.2 mgh \Rightarrow 0.8mgh = \frac{1}{2}mv^2 \Rightarrow \sqrt{1.6gh} = v .

This ultimately computes to:

v = 1.6 ( 10 ) ( 4 ) = 64 = 8 v = \sqrt{1.6(10)(4)} = \sqrt{64} = \boxed{8} m/s.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...