A calculus problem by Shourya Pandey

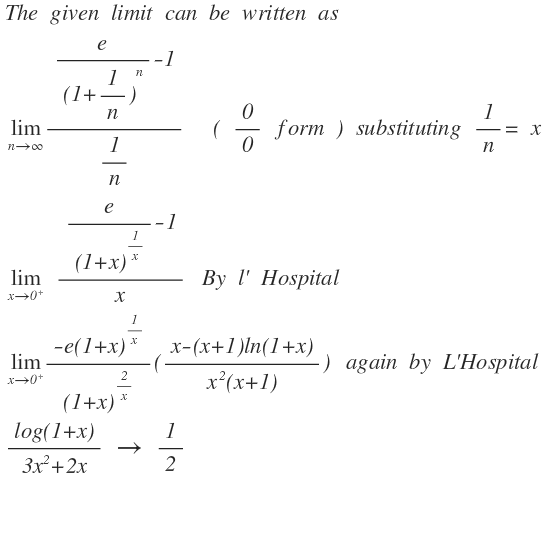
I am a little short on time so i will just give a quick insight to what I did. Take the LCM of the expression $\lim_{n \to\infty} \dfrac{n^{n+1}e-n(n+1)^n}{(n+1)^n}$ Divide numerator and denominator by $n^{n+1}$ , $\lim_{n \to\infty} \dfrac{e-(1+\frac{1}{n})^n}{\frac{1}{n}(1+\frac{1}{n})^n}$ Substitute $\frac{1}{n}=x$ and change the limit as, $\lim_{x\to 0} \dfrac{e-(1+x)^{\frac{1}{x}}}{x(1+x)^{\frac{1}{x}}}$ Now since $\lim_{x \to 0} (1+x)^{\frac{1}{x}} =e$ therefore we have the $\frac{0}{0}$ form which can be easily analyzed with L Hopitals rule. One formula which you may use while applying L Hopitals rule is $\lim_{x\to 0} \dfrac{ln(1+x)}{x}=1$