A Car on Banked Turn

Classical Mechanics Level 4

A car turns on a banked curve of $45^\circ$ angle with respect to the horizontal.

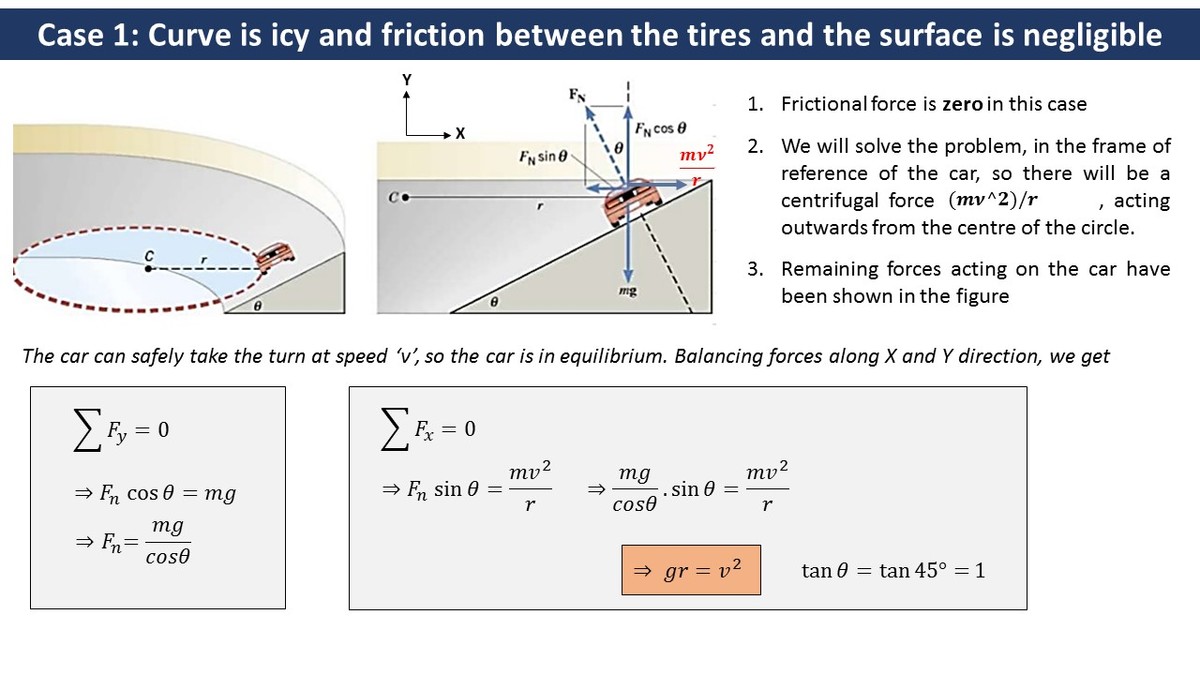
When the curve is icy and friction between the tires and the surface is negligible, the car can turn safely at speed $v$ .

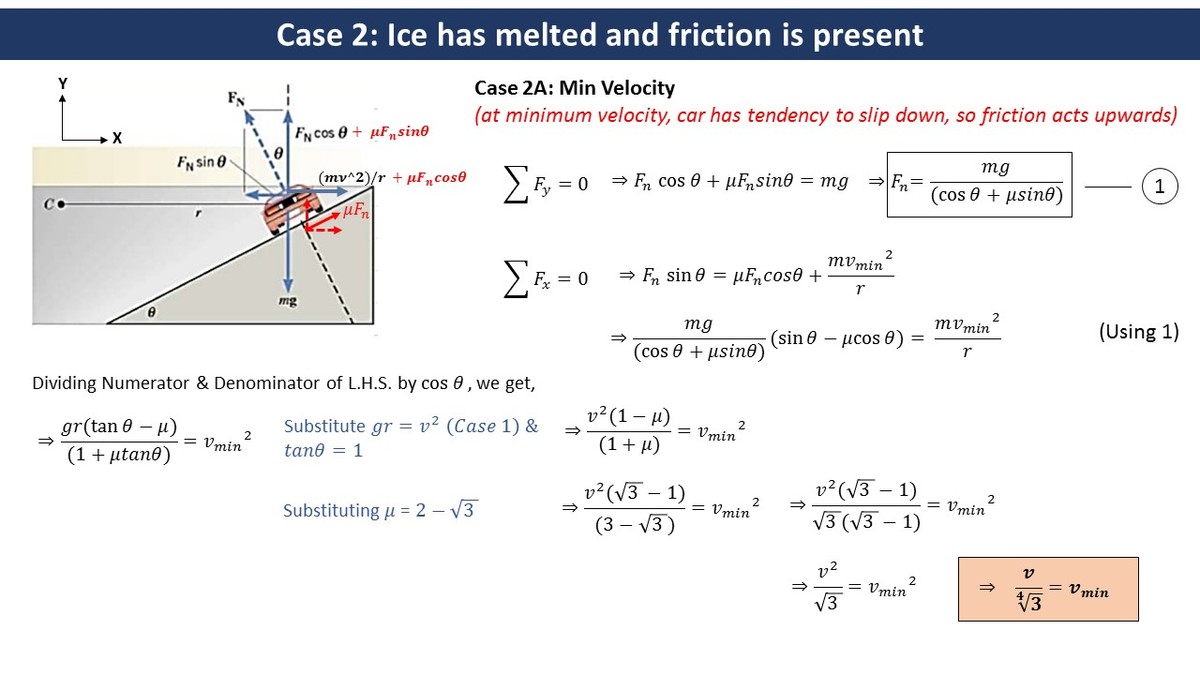
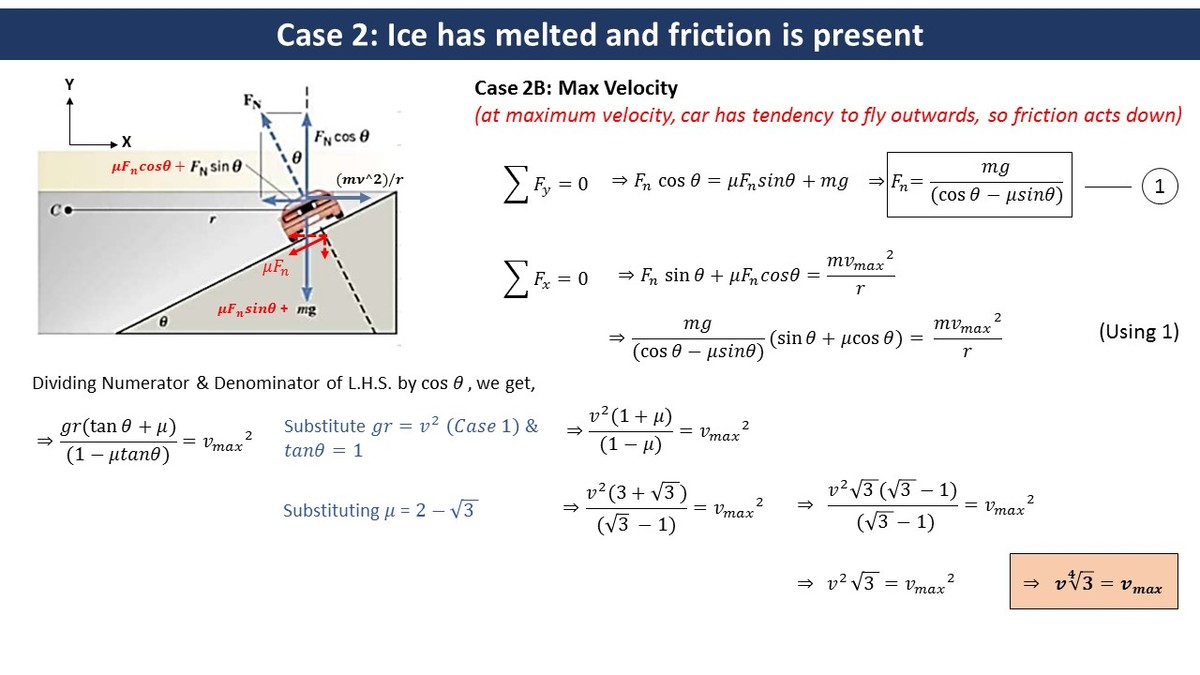
What are the maximum velocity $v_\text{max}$ and minimum velocity $v_\text{min}$ , respectively, at which the car can turn safely when the ice melts and the coefficient of static friction between the tires and the surface is $2-\sqrt 3?$

v_{max}=\sqrt[4]2v,v_{min}=\dfrac v {\sqrt[4]2}

v_{max}=\sqrt2v,v_{min}=\dfrac v {\sqrt2}

v_{max}=\sqrt3v,v_{min}=\dfrac v {\sqrt3}

v_{max}=\sqrt[4]3v,v_{min}=\dfrac v {\sqrt[4]3}

1 solution

Shouvik Chaudhury
Jul 18, 2018

A Car on Banked Turn

1 solution

0 pending reports