A charge in motion
A particle of mass m and charge q is projected with v = v 0 i ^ in a non uniform magnetic field which is given by → B = − B 0 x k ^ , where B 0 , v 0 are positive constants. Find the maximum x coordinate of the particle during its motion.
Details and Assumptions
Assume that the particle enters the x − y plane at origin with the given velocity.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
yes, I liked this problem so i decided to share it here as well.
Log in to reply
which batch? 2016 or 2017? which rank did you get in previous jee mains (in vsat)?
Log in to reply
2017 batch, I got my best ever rank in mains 6 which i gave offline, about 206. I gave mains 7 online, so i didn't get any rank, which centre do you belong to?
Really nice problem. Keep posting!
Log in to reply
Thanks Swapnil, this problem was actually given by our sir for our practice :)
@Swagat Panda Do see this solution.
Very nice, frankly speaking, I didn't know the radius of curvature formula for any curve which you mentioned prior to reading your solution. You taught me a new handy formula.
When I attempted this question for the very first time, I tried to use the centripetal force formula with Lorentz force formula to find x m a x but because I didn't know at that time that this sort of formula exists, I was unsuccessful. In a way, you fulfilled one of my incomplete wishes to solve this question in another way. :)
Nice dude …bdw are u studying in fitjee??
Yes. I'm from Fiitjee Banglore
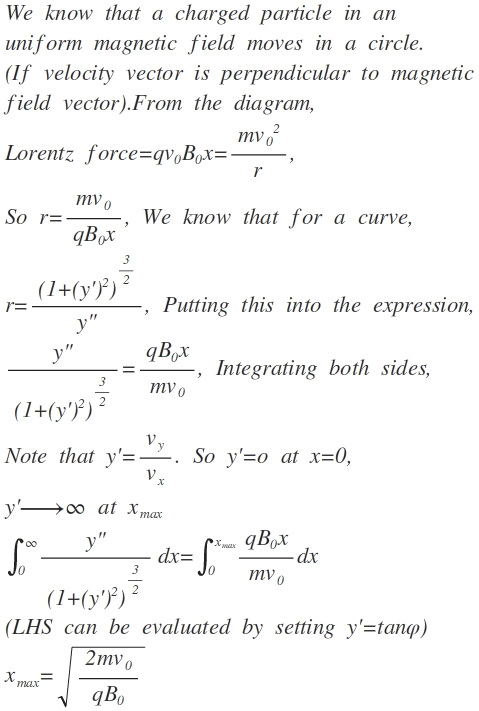
Relevant wiki: Lorentz Force Law (Magnetic and Mixed Fields)
Now, using Lorentz force equation we get m a y = q v 0 B 0 x c o s θ ⇒ m a y = q ( v 0 c o s θ ) B 0 x m d t d v y = q B 0 x d t d x ⇒ m ∫ 0 v 0 d v y = q B 0 ∫ 0 x m a x x d x m v 0 − 0 = q B 0 2 x m a x 2 ⇒ x m a x = q B 0 2 m v 0