A Cyclic Quadrilateral
In the figure, C A = 2 , C B = 3 , ∠ C A P = ∠ P B C = 9 0 º and ∠ A P B = 6 0 º . How long is P C ?
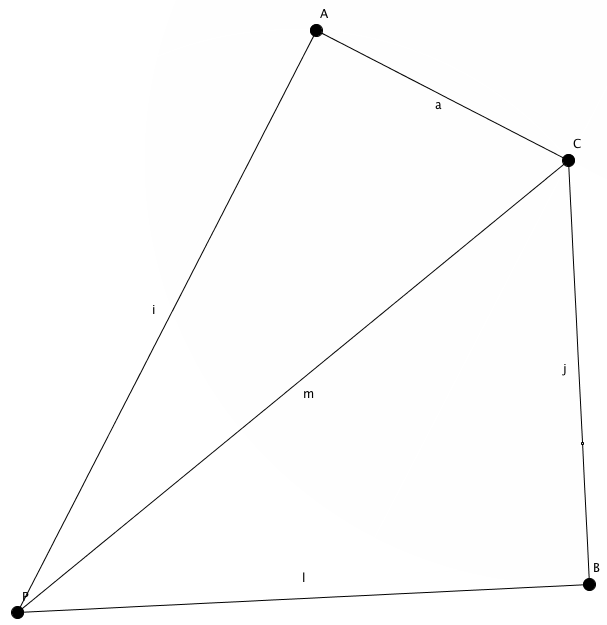
The answer is 5.033.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
nice solution sir.
In this special case, you could've used the definition of trigonometric ratios (which you have, implicitly).
A
B
=
x
⇒
x
2
=
A
C
2
+
C
B
2
−
2
⋅
A
B
⋅
B
C
⋅
cos
(
1
2
0
º
)
=
4
+
9
+
6
=
1
9
A
P
=
y
,
P
B
=
z
⇒
z
2
+
y
2
−
2
y
z
cos
(
6
0
º
)
=
x
2
⇒
z
2
+
y
2
−
y
z
=
1
9
P
C
2
=
z
2
+
3
2
=
y
2
+
2
2
⇒
y
2
=
5
+
z
2
⇒
z
2
+
y
2
−
y
z
=
z
2
+
(
5
+
z
2
)
−
z
(
5
+
z
2
)
=
1
9
⇒
z
=
3
7
⇒
P
C
=
z
2
+
3
2
=
3
4
9
+
9
=
3
2
5
7
≈
5
.
0
3
3
Let P C = x and ∠ A P C = θ . Then, by sine rule:
⎩ ⎪ ⎨ ⎪ ⎧ sin 9 0 ∘ x = sin θ 2 sin 9 0 ∘ x = sin ( 6 0 ∘ − θ ) 3 ⇒ x = sin θ 2 ⇒ x = sin ( 6 0 ∘ − θ ) 3 . . . ( 1 ) . . . ( 2 )
sin ( 6 0 ∘ − θ ) 3 3 sin θ 3 sin θ 3 sin θ 4 sin θ ⇒ tan θ ⇒ sin θ ( 1 ) : x = sin θ 2 = 2 sin ( 6 0 ∘ − θ ) = 2 ( 2 3 cos θ − 2 1 sin θ ) = 3 cos θ − sin θ = 3 cos θ = 4 3 = 1 9 3 = sin θ 2 = 2 3 1 9 ≈ 5 . 0 3 3