Balancing a Semicircle Frame

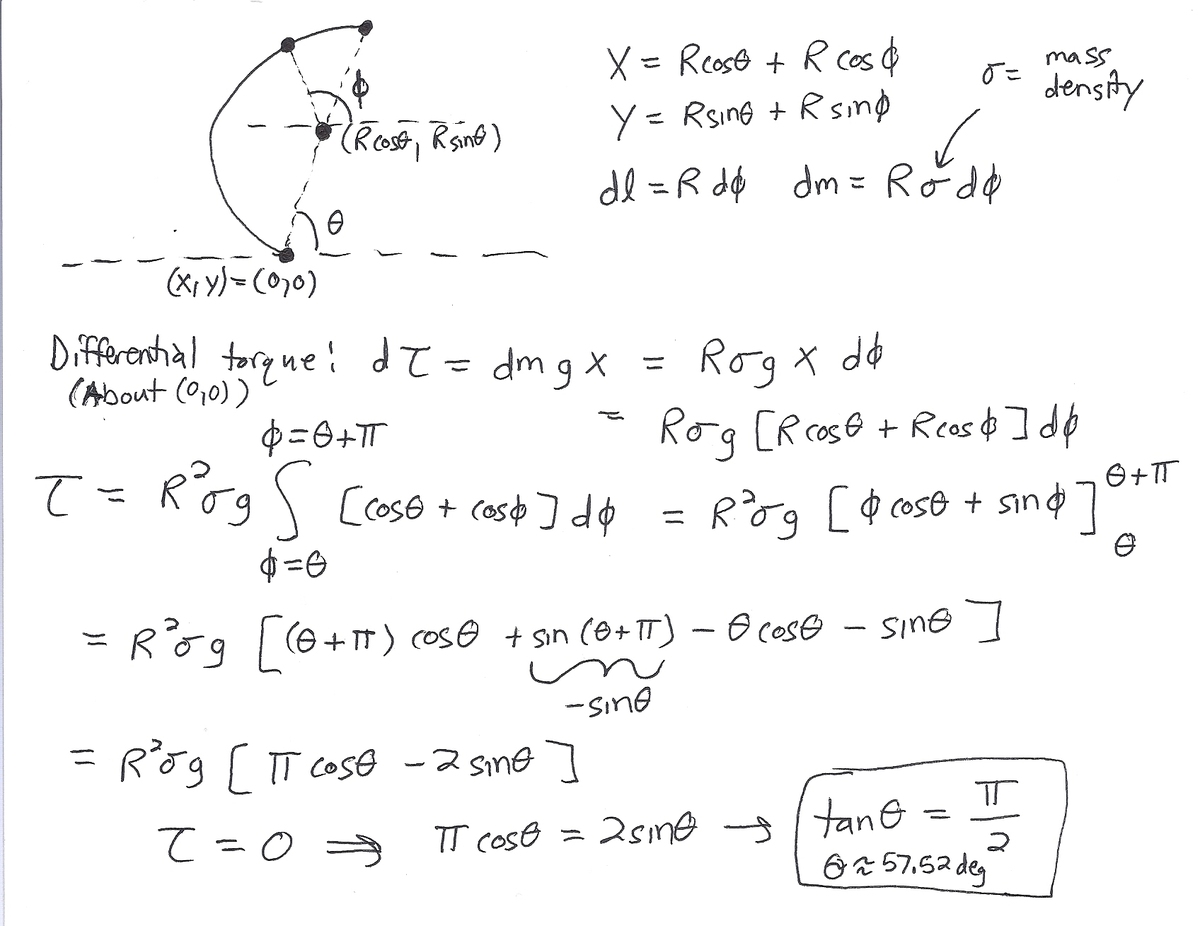
For the semicircle not to topple in any direction, the center of mass of the semicircle must be vertically on top of the contact point $P$ . Therefore we need to find the center of mass of the semicircle.

Consider a semicircle of radius $r$ as shown in the figure. Due to symmetry, the center of mass lies on $y$ -axis. Let the center of mass be $y_{c.m.}$ from the origin and the mass per unit be $\lambda$ . Then we have:

$\begin{aligned} y_{c.m.} & = \frac {\int_0^\pi \lambda r y \ d \phi}m & \small \color{#3D99F6}\text{where }m = \lambda \pi r \text{ is the mass of the semicircle} \\ & = \frac {\int_0^\pi \lambda r^2 \sin \phi \ d \phi}{\lambda \pi r} \\ & = \frac r \pi \cdot \cos \phi \big|_\pi^0 \\ & = \frac {2r} \pi \end{aligned}$

We note that $\tan (90^\circ - \theta) = \dfrac {y_{c.m.}}r = \dfrac 2\pi$ $\implies 90^\circ - \theta = 32.482$ $\implies \theta = \boxed{57.52}$ .