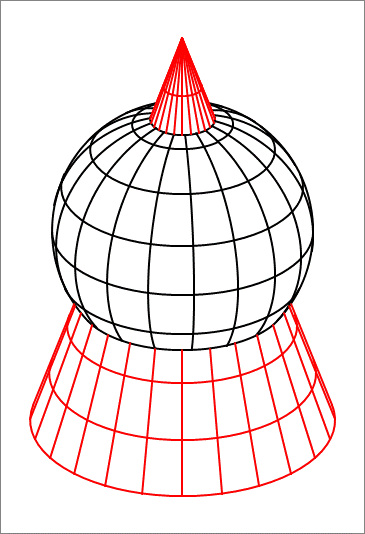
A composite of a cone and a sphere

In the cross-section, say in the $x z$ plane, the composite appears as a triangle superimposed on a circle, as shown below.

Let $\theta_0$ be the semi-vertical angle of the cone, then

$\theta_0 = \tan^{-1} \dfrac{1}{2 \sqrt{2}}$

The equation of its slant edge is $z = 20 - (\cot \theta_0) x$

while the parametric equation of the circle is $(x, z) = ( 6 \sin \theta, 0, 10 + 6 \cos \theta )$

Plugging in the second equation into the first, we can find the angles $\theta$ where the surface of the sphere intersects the surface of the cone. And this turns out to be at $\theta_1 = 0.249194$ and at $\theta_2 = 2.21272$ .

Now, the area of the surface of the sphere that is outside the cone is given by

$A_s = 2 \pi R^2 (\cos \theta_1 - \cos \theta_2 )$

where $R = 6$ is the radius of the sphere. The area of the cone that lies inside the sphere is given by

$A_c = \pi ( r_2 s_2 - r_1 s_1 ) = \pi R^2 ( \sin^2 \theta_2 - \sin^2 \theta_1 ) \left( \dfrac{1}{\sin \theta_0} \right)$

Taking as our pivot point the center of the sphere, the required volume can be written as

$V = \dfrac{1}{3} \left( R A_s - d A_c \right)$

where $d$ is the (constant) perpendicular distance from the center of the sphere to the surface of the cone. It is given by

$d = 10\sin \theta_0$

Evaluating the above, we obtain $V = 490.3663...$ ; therefore the answer is $\lfloor 1000V \rfloor =\boxed{ 490366}$ .