A Definite Problem
∫ ln 2 ln 3 sin ( x 2 ) + sin ( ln 6 − x 2 ) x sin ( x 2 ) d x
The integral above has a closed form. Find the value of this closed form.
Give your answer to 3 decimal places.
The answer is 0.101.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Same solution again, we are so in sync
Log in to reply
Haha... I have this similarity with many people on brilliant.... Welcome to that club. XD
Did the same way. (+1)

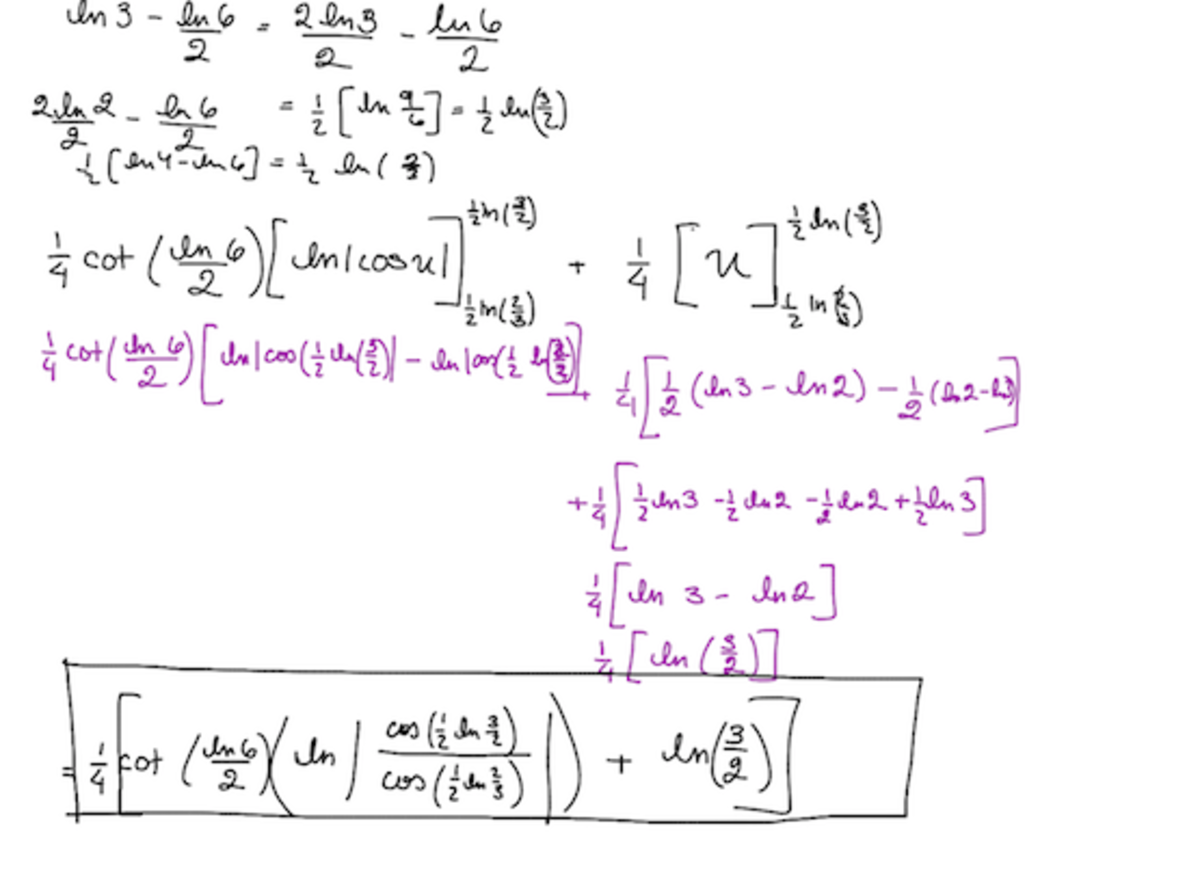
I did it the long way, but that's alright, someone ought to show the long way too :p
Relevant wiki: Integration Tricks
Substitute x 2 = t such that 2 x d x = d t
I = 2 1 ∫ ln 2 ln 3 sin t + sin ( ln 6 − t ) sin t d t
Now use ∫ a b f ( x ) d x = ∫ a b f ( a + b − x ) d x so that:
I = 2 1 ∫ ln 2 ln 3 sin t + sin ( ln 6 − t ) sin ( ln 6 − t ) d t
Adding we get I = 4 1 ∫ ln 2 ln 3 d t = 4 1 ln ( 2 3 ) ≈ 0 . 1 0 1