Ladder Dynamics

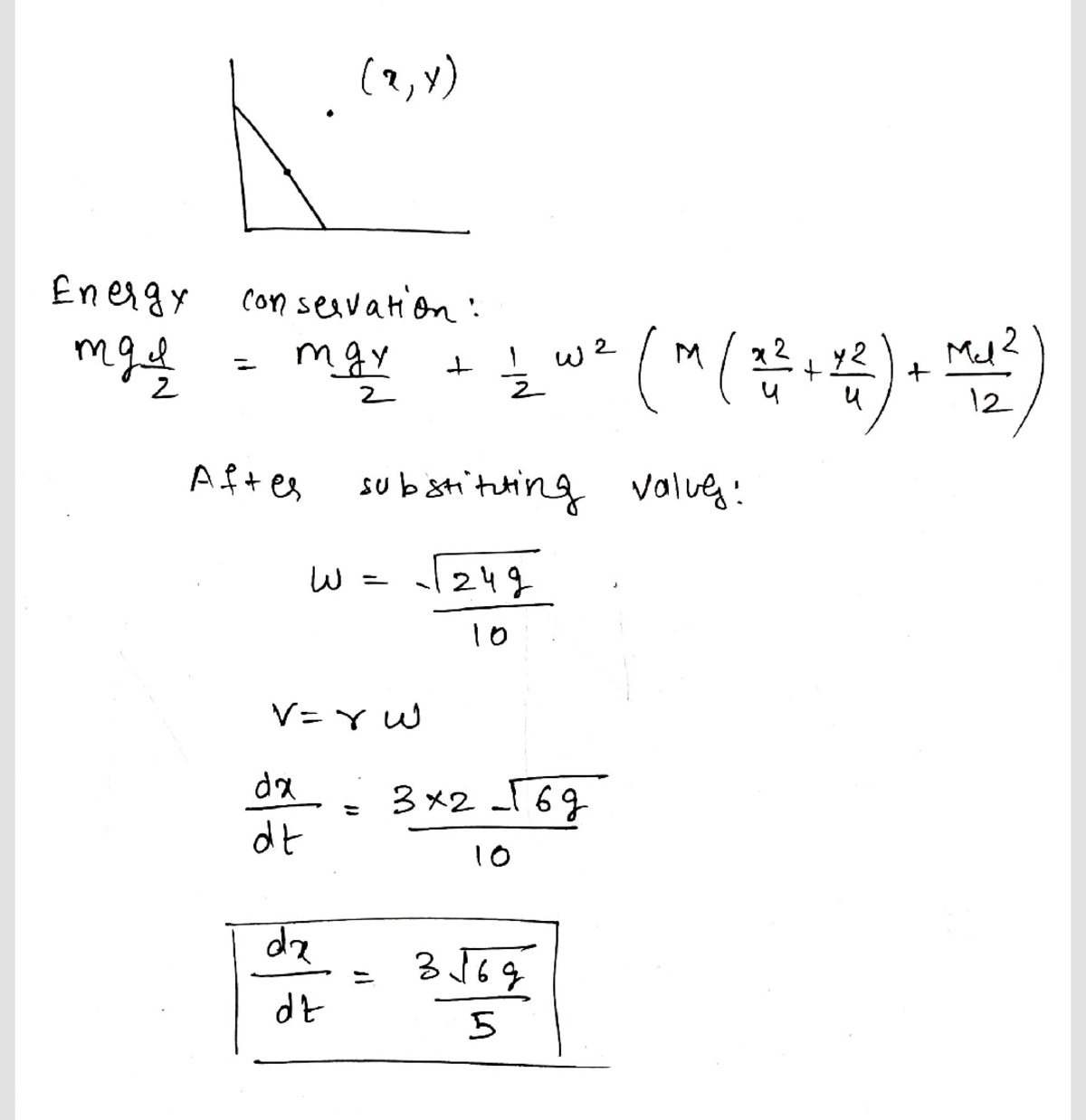
Let the acute angle that the ladder makes with the X-axis be $\theta$ . Assuming the ladder has mass uniformly distributed, it's COM lies at its geometric centre. With this in mind, the coordinates of the COG of the ladder are:

$x_L = \frac{L}{2}\cos{\theta}$ $y_L = \frac{L}{2}\cos{\theta}$

Kinetic energy of the rod:

$\mathcal{T} = \frac{m}{2}(\dot{x}_L^2 + \dot{y}_L^2) + \frac{I_{COM}}{2}\dot{\theta}^2$ $I_{COM} = \frac{mL^2}{12}$

Simplifying:

$\mathcal{T} = \frac{mL^2\dot{\theta}^2}{6}$

Potential energy of the ladder is:

$\mathcal{V} = \frac{mgL}{2}\cos{\theta}$

Applying conservation of energy gives:

$\mathcal{T} + \mathcal{V} = \frac{mgL}{2}$

Plugging in expressions and solving for $\dot{\theta}$ : $\dot{\theta}= - \sqrt{\frac{3g}{L}(1-\sin{\theta})}$

The minus sign is introduced because as $\theta$ decreases, $\dot{\theta}$ increases. When $y=3$ , $\sin{\theta} = 0.6$ which gives:

$\dot{\theta} =- \frac{\sqrt{6g}}{5}$

The X coordinate of the end of the ladder on the X axis is:

$x = L\cos{\theta}$ $\dot{x} = -L\dot{\theta}\sin{\theta} = \boxed{\frac{3}{5}\sqrt{6g}}$

I used the concept of instantaneous axis of rotation.
Through that particular point whole system begins to move in pure rotation.
Feel free to ask anything regarding my solution.