A familiar sequence

Electricity and Magnetism Level 2

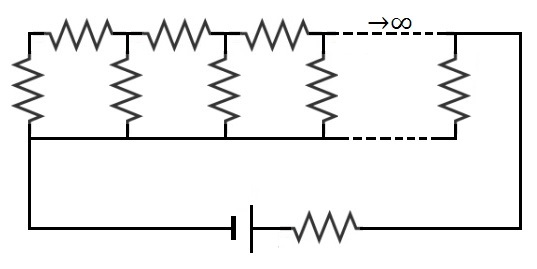
In the above circuit, each resistor has a resistance of 1 $\Omega$ and the wires have no resistance.

As the number of resistors approaches infinity, the equivalent resistance approaches $\dfrac{a + \sqrt{b}} { c},$ where $a$ , $b$ , and $c$ are positive integers, and $b$ is square free. What is $a + b + c$ ?

The answer is 8.

2 solutions

Chew-Seong Cheong
May 1, 2017

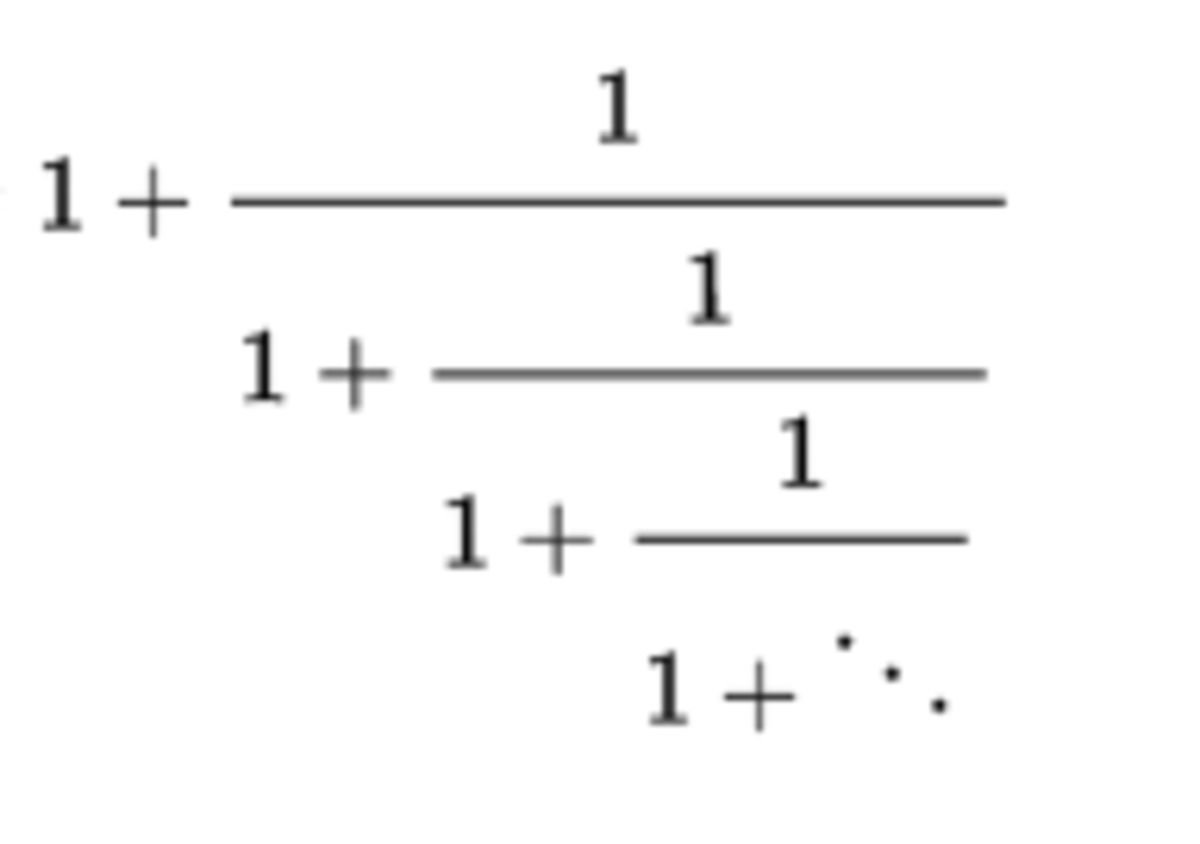
Let the equivalent resistance of the infinite lattice resistor network be $R$ , then we note that:

$\begin{aligned} R & = 1 || (1+R) \\ & = \frac {1+R}{2+R} \\ \implies R^2 + R - 1 & = 0 \\ \implies R & = \frac {\sqrt 5 - 1}2\end{aligned}$

Therefore, the equivalent resistance of the whole circuit is: $R_{eq} = 1 + R = 1+\dfrac {\sqrt 5-1}2 = \dfrac {1+\sqrt 5}2$ .

$\implies a+b+c = 1+5+2 = \boxed{8}$

Alex Li
Apr 30, 2017

The bottom resistor provides resistance 1.

The first split is in parallel, and has resistance 1 / ( 1 / 1 + 1 / (whatever the second split resists)).

The second split has resistance 1 / ( 1 / 1 + 1 / (whatever the third split resists)).

And so on.

Subsituting, we get the resistance to be

This may be familiar - it is the golden ratio! The answer is therefore (1 + sqrt(5)) / 2. 1 + 5 + 2 = 8

A familiar sequence

The answer is 8.

2 solutions

0 pending reports