A frozen pendulum
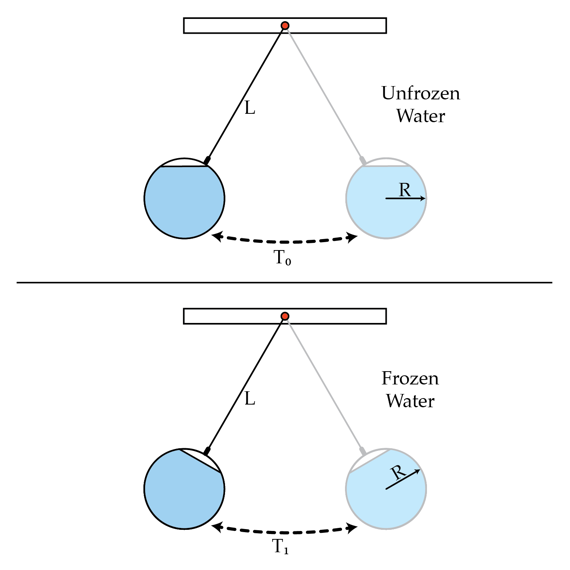
A pendulum is constructed as a light thin-walled sphere filled up with water and suspended from the ceiling by a rigid rod of negligible mass. The radius of the spherical container is R and the distance between the pivot point and the center of mass of the system is L. The period of small oscillations is T 0 . Due to a temperature drop in the room the water froze and the pendulum began to oscillate with a different frequency, corresponding to the period T 1 . Determine T 0 T 1 if R L = 2 . Neglect the viscosity (friction) of the water and the change of its volume after freezing.
The answer is 1.05.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
The key observation is the following: When the container with water ( in liquid phase) oscillates, the system behaves as a simple pendulum. Note that the liquid does not rotate around its center of mass and therefore the energy of the system is simply
E
=
2
M
v
2
+
M
g
h
where v and h are the velocity and height of the center of mass. The last expression suggests that even though we are describing an object of finite dimensions, its energy coincides with that of a point-like mass. Consequently, the system can be described as a simple pendulum.
On the other hand, when the water freezes, the system becomes rigid and it should be described as a physical pendulum. Then the total energy of the system must include the rotational kinetic energy, that is
E
=
2
M
v
2
+
2
I
ω
2
+
M
g
h
where
I
=
5
2
M
R
2
is the moment of inertia with respect to the center of mass.
Now we can apply the well-known formulas
T
0
=
2
π
g
L
for a simple pendulum and
T
1
=
2
π
M
g
L
I
+
M
L
2
for a physical pendulum.
Combining the above equations we obtain:
T
0
T
1
=
1
+
5
2
L
2
R
2
=
1
.
0
5
.
Before freezing, the water can move freely in the container and the kinetic energy of the pendulum includes only the translational energy of the center of mass. Therefore, its period of small oscillations is the same as a simple pendulum's: T 0 = 2 π g L
After freezing, the system became a rigid body which possesses also the rotational energy. Because of the spherical shape of the container, the moment of inertia of the pendulum about the pivot point is I = I 0 + m L 2 = 5 2 m R 2 + m L 2 , where m is the mass of the pendulum. The new period of small oscillations is T 1 = 2 π m g L I = 2 π g L ( 1 + 5 L 2 2 R 2 )
Finally, we receive the ratio T 0 T 1 = 1 + 5 L 2 2 R 2
If R L = 2 then T 0 T 1 ≈ 1 . 0 5