Several Areas Of Interest

Relevant wiki: Triangles - Identify Congruent Triangles

The area of square $BCDE = 12 \times 12 = 144$ . The area of $\triangle ACD$ equals twice the area of square $BCDE$ . Therefore area $\triangle ACD = 288$ .

$\large \begin{aligned} \text{Area of }\triangle ACD & = \frac{CD \times AD}{2} \\ 288 & = \frac{12\,\, AC}{2}\\ 288&=6\,\,AC \\ \therefore \quad \quad AC&=48 \end{aligned}$ .

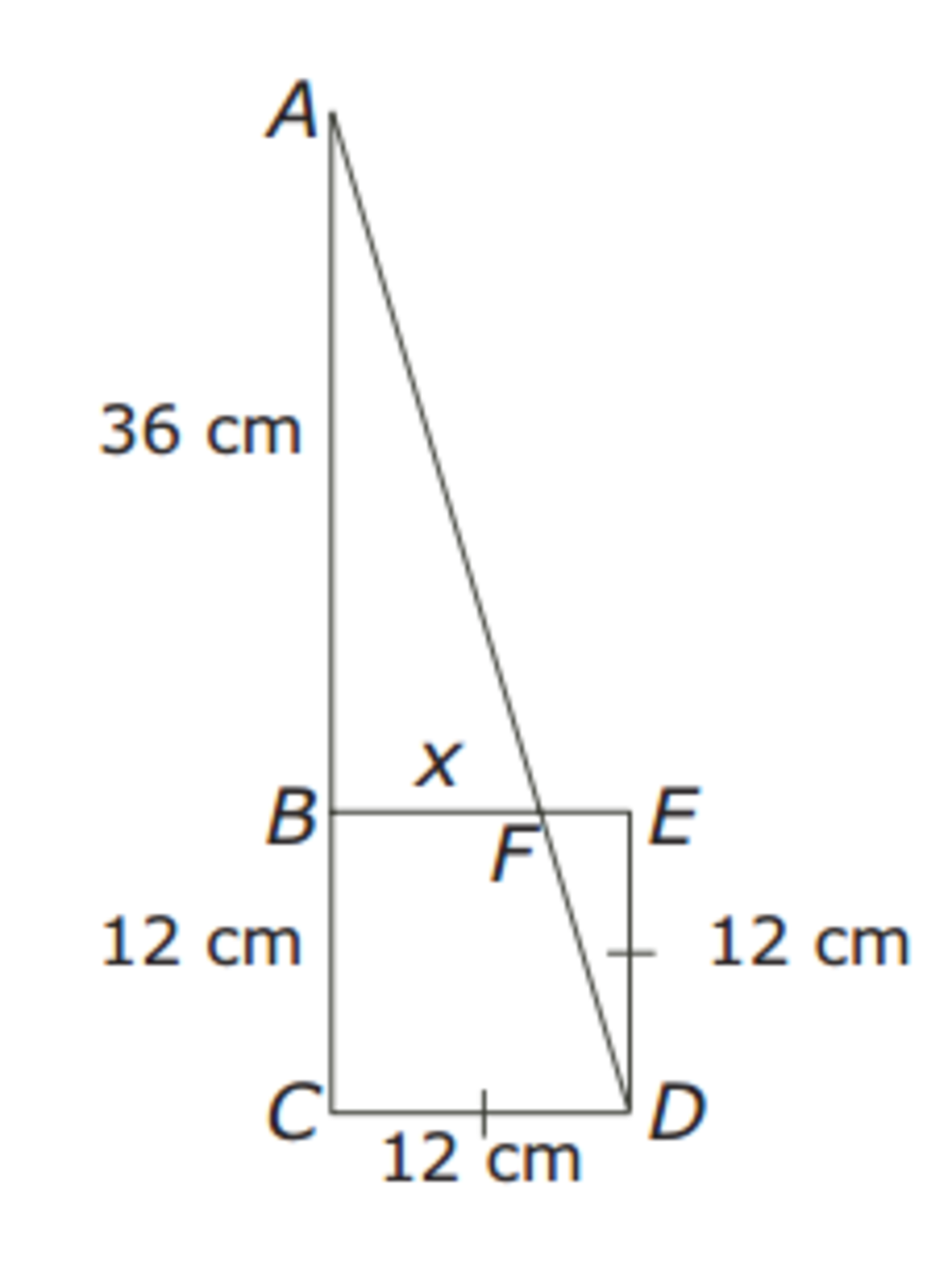
Let $BF=x$ .

In $\triangle ABF$ and $\triangle ACD$ , $\angle A$ is common and $\angle ABF = \angle ACD = 90^{\circ}$

It follows that $\triangle ABF$ and $\triangle ACD$ are similar and $\frac{AB}{AC}$ $=$ $\frac{BF}{CD}$ .

$\therefore \frac{36}{48}= \frac{x}{12}$ . So $x=9$

Since $BF$ is parallel to $CD$ , the quadrilateral $BCDF$ is a trapezium / trapezoid.

$\large \begin{aligned} \text{Area of } BCDF &=\frac{BC(BF+CD)}{2} \\ &= \frac{12(9+12)}{2} \\&=6(21)\\ &= \boxed{\boxed{\boxed{126 \text{unit}^2}}} \end{aligned}$

if the area of the triangle is twice the area of the square we could cut off the top of the triangle and put point A to point D and cut it such that we have a rectangle formed by a second square on top of BCDE. this means AC must be 48 and if the slope of AD is -4 then FE is 1/4 BE or 3. then the area of DEF is 18 and the area of BCDF is 144 - 18 = 126.