Middle Square Gone Missing!
Geometry
Level
1
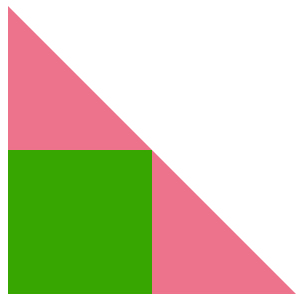
Consider a right-angled isosceles triangle with a square inscribed in the corner.
Which area is larger, the green area or the pink area?
Green Area
Pink Area
Equal Area
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Relevant wiki: Length and Area
Let's call the side of the square A and the side of the big triangle L .
The area of the square is A 2 .
Both small triangles have sides A and L − A ,
therefore they have the same areas.
The pink area is 2 ( L − A ) ( A ) ⋅ 2 = A L − A 2 .
Now, the area of the big triangle is 2 L 2 = ( A L − A 2 ) + A 2 = A L . And so, 2 A = L .
Replacing we get that the pink area is 2 A 2 − A 2 = A 2 , therefore both areas are equal.