A geometry problem by Harrison Froats

I used the 30-60-90 triangles with factor 10.5. This means the other sides are 10.5 * 0.5 and 40 * 0.5 * sqrt(3).

=sin(30)\(\frac{opp}{10.5} )

$opp=sin(30) x 10.5$

$opp=5.25cm$

$=10.5^2 - 5.25^2$

$= /sqrt[2]{82.68}$

$=9.09$

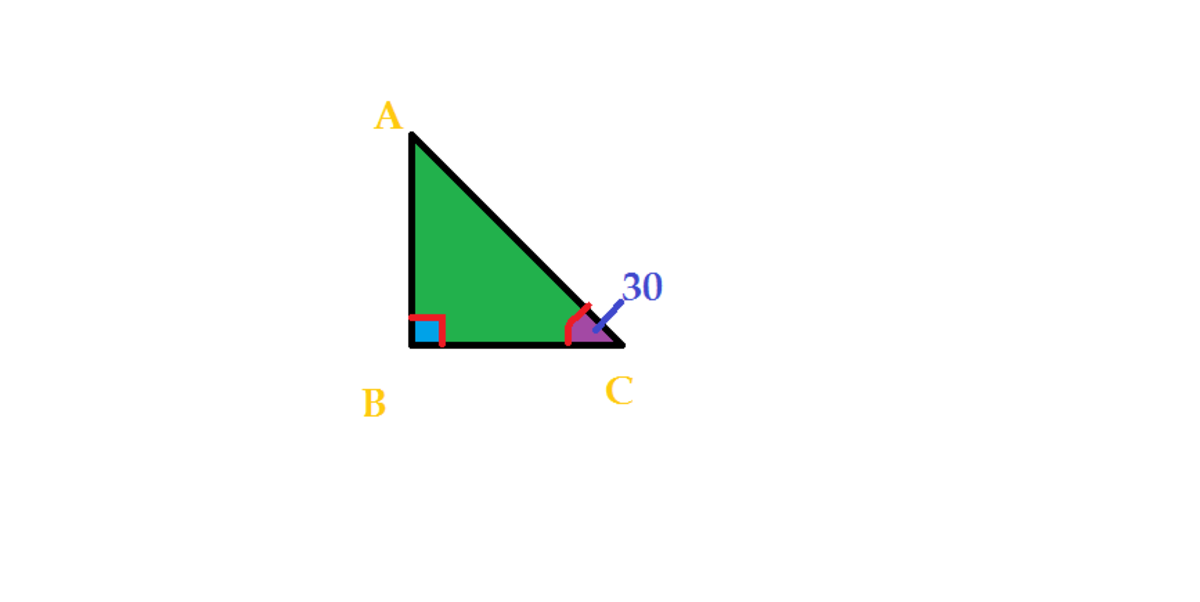$Here, AC = 10.5 \\ \begin{aligned} Also, \angle C = 30^\circ \implies \sin 30 = \dfrac{1}{2} \\ \implies \dfrac{AB}{AC} = \dfrac12 \\ \implies \dfrac{AB}{10.5} = \dfrac12 \\ \therefore AB = \boxed{5.25} \end{aligned} \\ Now, \cos 30 = \dfrac{\sqrt 3}{2} \\ \dfrac{BC}{AC} = \dfrac{\sqrt 3}{2} \\ \dfrac{BC}{10.5} = \dfrac{\sqrt 3}{2} \implies BC = 5.25\sqrt3 \approx \boxed{9.09}$

Given that we have 1 side length (10.5cm) and 2 angles (30° and 90°), we can use sine law.

$\frac{a}{sinA}=\frac{b}{sinB}$

$a=sin(30°)×\frac{10.5cm}{sin(90°)}$

$a=5.25cm$

There is only one answer that has 5.25cm as a side length, therefore we don't have to solve for the other side length as it is the only option.