A geometry problem by A Former Brilliant Member

$AC=\sqrt{36^2+27^2}=45$

$\triangle ABC$ is similar to $\triangle AOF \implies \dfrac{OF}{AO}=\dfrac{BC}{AB}=\dfrac{27}{36}=\dfrac34$

$EF=2\times OF=2\times\dfrac34AO=\dfrac32\times\dfrac{45}2=\dfrac{135}4$

Answer $=135+4=\boxed{139}$

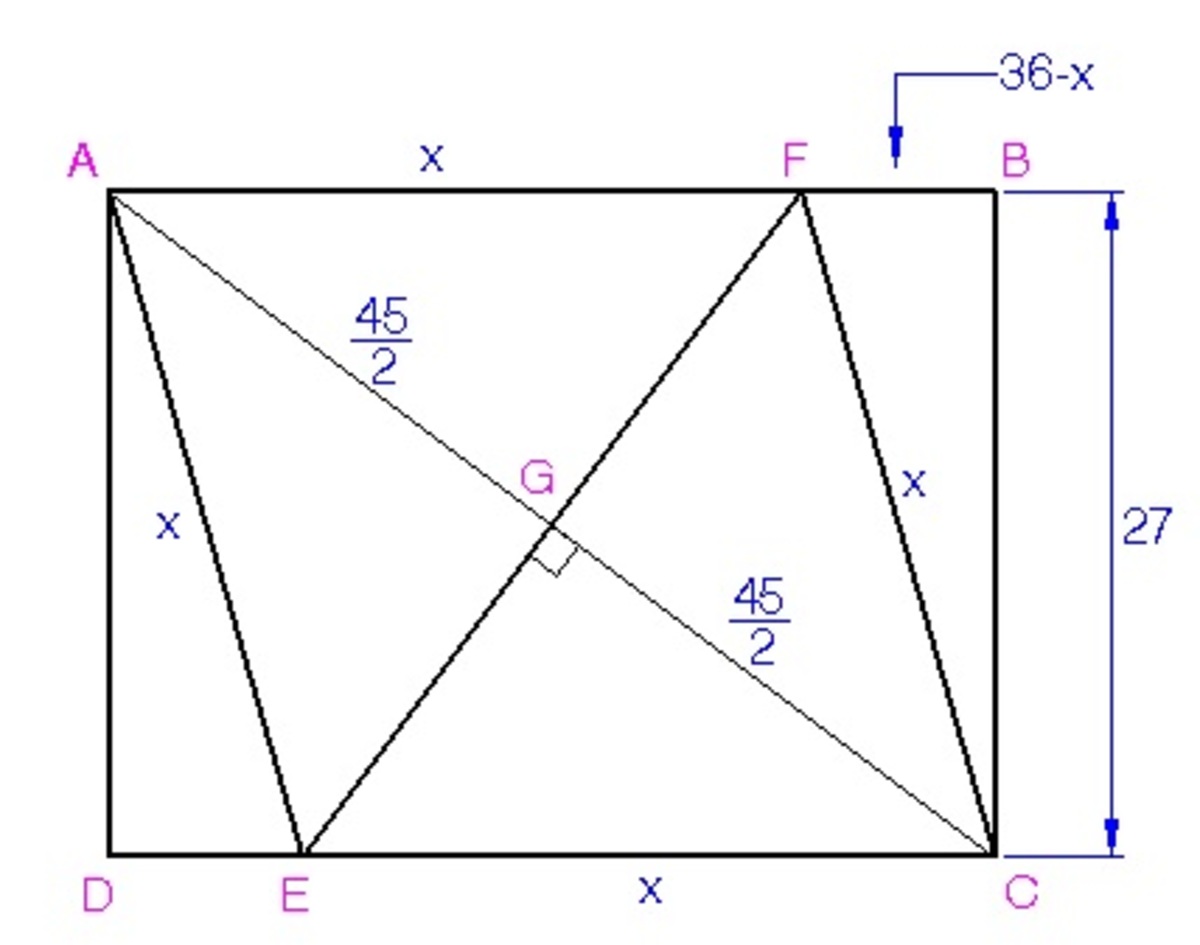Let the each side length of the rhombus be $x$ .

Apply pythagorean theorem on $\triangle FBC$ :

$x^2=(36-x)^2+27^2=36^2-72x+x^2+729$ $\implies$ $72x=2025$ $\implies$ $x=\dfrac{225}{8}$

Apply pythagorean theorem on $\triangle ADC$ :

$(AC)^2=36^2+27^2$ $\implies$ $AC=45$

Since the diagonals of a rhombus are perpendicular and bisect each other, $\triangle EGC$ is a right $\triangle$ , and $GC=\dfrac{45}{2}$ .

Apply pythagorean theorem on $\triangle EGC$ .

$(EG)^2=(EC)^2-(GC)^2=\left(\dfrac{225}{8}\right)^2-\left(\dfrac{45}{2}\right)^2=\dfrac{50625}{64}-\dfrac{2025}{4}=\dfrac{18225}{64}$

$EG=\sqrt{\dfrac{18225}{64}}=\dfrac{135}{8}$

It follows that,

$EF=2EG=2\left(\dfrac{135}{8}\right)=\dfrac{135}{4}$

Finally,

$a+b=135+4=$ $\boxed{139}$