About Parallels Misconceptions

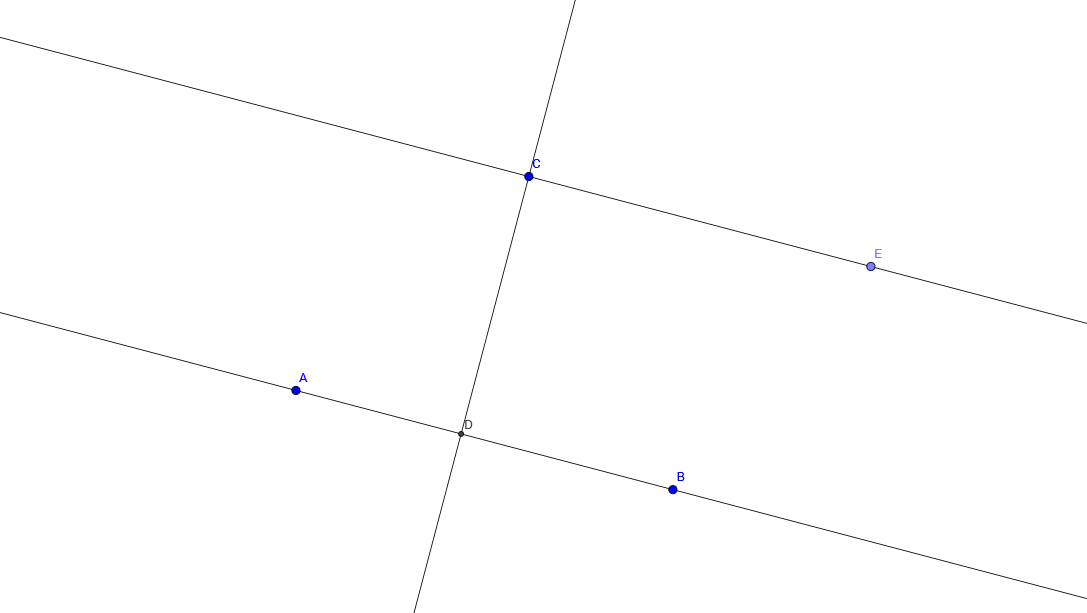
Let $F$ be a plane. Let $\overleftrightarrow { AB }$ be a line such that $\overleftrightarrow { AB } \subseteq F$ and let C be a point such that $C\in F$ , but $C\notin \overleftrightarrow { AB }$ . From previous theorems we know that there exists one and only one perpendicular line to $\overleftrightarrow { AB }$ through point $C$ that does not yield on the line. Let $\overleftrightarrow { CD }$ be that line. Another previous theorem states that there exists one and only one perpendicular line to $\overleftrightarrow { CD }$ through point $C$ (which yields on $\overleftrightarrow { CD }$ ). Let $\overleftrightarrow { CE }$ be that line. This line seems to be the candidate to be parallel to $\overleftrightarrow { AB }$ .

I will procede by contradiction to show that, in fact, $\overleftrightarrow { CE }$ is parallel to $\overleftrightarrow { AB }$ . Let's suppose that they are not parallel and let G denote their intersection. As $\overleftrightarrow { CE }$ is perpendicular to $\overleftrightarrow { CD }$ , they form 4 right angles. As $\overleftrightarrow { AB }$ is perpendicular to $\overleftrightarrow { CD }$ , they form 4 right angles. By the Exterior Angle Theorem, we know that in every triangle, the exterior angle is greater than the triangle angles that are not adjacent. Thus, we have a contradiction, because the exterior angle is equal to one of the not-adjacent angles of the triangle. Hence, the lines are parallel. Note that I have proved the existence of the parallel line, but no one can proof that this line is unique. Therefore, there is at least one line that can be drawn parallel to another given one through an external point.