A geometry problem by Mehul Chaturvedi
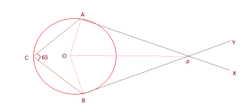 A circle with centre
is touching two intersecting lines
and
. The two points of contact
and
subtend an angle of 65 degreess at any point C on the circumference of the circle. If
is the point of the intersection of the two lines, then find the measure of angle
given that straight lines
and
are tangents.
A circle with centre
is touching two intersecting lines
and
. The two points of contact
and
subtend an angle of 65 degreess at any point C on the circumference of the circle. If
is the point of the intersection of the two lines, then find the measure of angle
given that straight lines
and
are tangents.
The answer is 25.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Angle AOB= 2xAngle ACB= 130
Now, Angle AOP= Half Angle ACB= 65
By angle sum property, we get Angle APO= 180- (65+90)
= 180- 155
=25