A geometry problem by Muhammad Maulana

Geometry Level 3

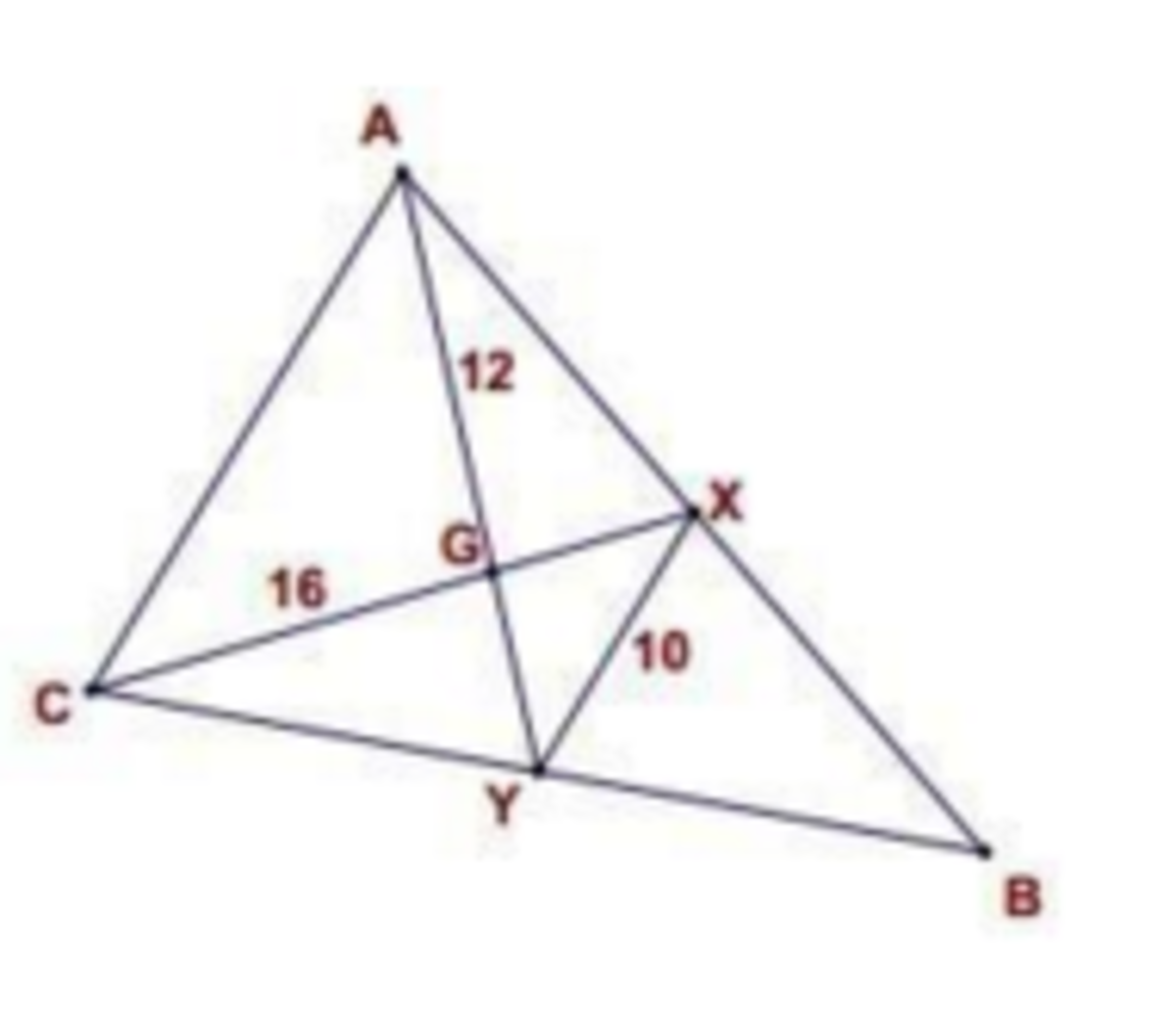
In triangle $ABC$ , let $X$ and $Y$ be the midpoints of side $AB$ and $BC$ respectively. Let $AY$ and $CX$ intersect at $G$ . If $AG = 12, CG = 16, XY = 10$ , what is the area of the triangle $GXY$ ?

The answer is 24.

1 solution

Venkata Karthik Bandaru
Feb 14, 2016

Since $AY, CX$ are medians and $G$ is the centroid, $GY = \dfrac{AG}{2} = \dfrac{12}{2} = 6$ units [Because centroid divides median in $2:1$ ratio]. Similarly, $GX = \dfrac{CG}{2} = 8$ units. Now, since we know side lengths of $\Delta GXY$ , apply Heron's formula to compute its area : $\boxed{24}$ square units.

Yes correct(+1)... $CX$ and $AY$ is midpoint in triangle $ABC$ , since: $CG:GX = AG:GY = 2:1 ...(1)$ Similarly $GX = 8 ~and~ GY = 6.$ Because $XY = 10$ , thus triangle $GXY$ where $\angle G=90 ^{\circ}$ . The area of the triangle of $GXY = \frac {(6.8)}2 = \boxed{24}$

Muhammad Maulana - 5 years, 4 months ago

A geometry problem by Muhammad Maulana

The answer is 24.

1 solution

0 pending reports