A geometry problem by Tanishq Varshney

Geometry Level 3

Consider a point $P$ on the line $y=x$ and two fixed point $A(3,4)$ and $B(6,13)$ .Then find $P$ such that $PA+PB$ is minimum.

\left(\frac {9}4, \frac {9}4 \right)

\left(\frac {15}2, \frac {15}2 \right)

\left(\frac {5}2, \frac {5}2 \right)

\left(\frac {17}4, \frac {17}4 \right)

2 solutions

Tanishq Varshney
Dec 25, 2014

take the image of point p in the line y=x which comes out to be A (4,3) using congruent triangles u can see that PA=PA Now take a point p ony=x say (t,t) from the slope we have slope of A B=PB solve for t A is the image of A in y=x. we cannaot take point P1 as PA+PB will not be minimum. also tr(APM) is congruent to tr(A*PM) by SAS RULE.

Elaborate it

Ankit Jain - 6 years, 5 months ago

I have tried this problem out in two different ways: Firstly graphically. The locus of points that have PA+PB constant is an ellipse with points A and B as its focuses. To minimise the size of PA+PB we need to find the smallest ellipse that will just touch the line y=x; Constructing this graphically in a CAD package shows the tangent being very close to (17/4,17/4).

Secondly algebraically: since x=y on the line we can substitute x for y and get;

PA + PB is $\sqrt{(x-3)^2 + (x-4)^2} + \sqrt{(x-6)^2+(x-13)^2}$

differentiating the above, then considering only the numerator, gives

$(x-7)\sqrt{2x^2-38x+205} + (x-19)\sqrt{2x^2-14x+25}$

this must be zero at the point where AP+BP is a minimum (or maximum), and it can be solved numerically to be exactly 17/4.

Ed Sirett - 4 years, 8 months ago

Chew-Seong Cheong
Apr 2, 2019

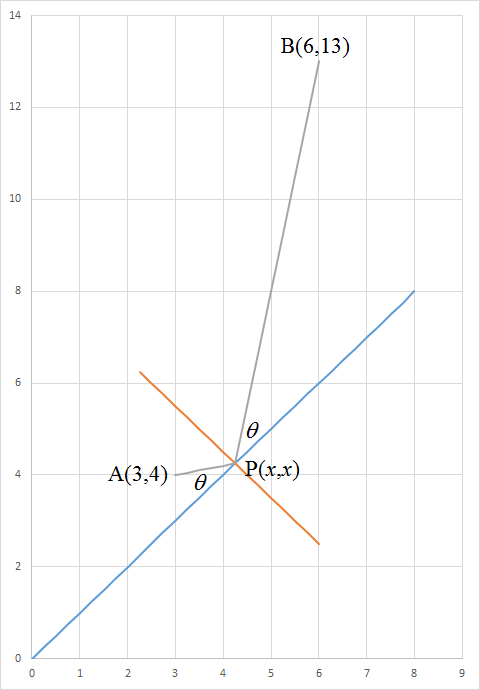
Since $P$ is on the line $y=x$ , let its coordinates be $P(x,x)$ . The shortest $PA+PB$ is when $PA$ is an incident light ray and $PB$ the reflected light ray at point $P$ on the mirror $y=x$ as shown in the figure. Since the incident angle equals to the reflective angle, the angle $PA$ makes with $y=x$ is the same as $PB$ makes with $y=x$ and equals to $\theta$ . Then the gradients of $PA$ and $PB$ , $m_A$ and $m_B$ respectively are given by:

$\begin{cases} m_A = \tan \left(\dfrac \pi 4 - \theta\right) = \dfrac {x-4}{x-3} & \implies \theta = \dfrac \pi 4 - \tan^{-1} \left(\dfrac {x-4}{x-3} \right) \\ m_B = \tan \left(\dfrac \pi 4 + \theta\right) = \dfrac {x-13}{x-6} & \implies \theta = \tan^{-1} \left(\dfrac {x-13}{x-6} \right) - \dfrac \pi 4 \end{cases}$

$\begin{aligned} \implies \frac \pi 4 - \tan^{-1} \left(\frac {x-4}{x-3} \right) & = \tan^{-1} \left(\frac {x-13}{x-6} \right) - \frac \pi 4 \\ \frac \pi 2 - \tan^{-1} \left(\frac {x-4}{x-3} \right) & = \tan^{-1} \left(\frac {x-13}{x-6} \right) \\ \cot^{-1} \left(\frac {x-4}{x-3} \right) & = \tan^{-1} \left(\frac {x-13}{x-6} \right) \\ \tan^{-1} \left(\frac {x-3}{x-4} \right) & = \tan^{-1} \left(\frac {x-13}{x-6} \right) \\ \frac {x-3}{x-4} & = \frac {x-13}{x-6} \\ (x-3)(x-6) & = (x-13)(x-4) \\ x^2 - 9x + 18 & = x^2 - 17x + 52 \\ 8x & = 34 \\ \implies x & = \frac {17}4 \end{aligned}$

Therefore, $P\boxed{\left(\frac {17}4, \frac {17}4\right)}$ .

A geometry problem by Tanishq Varshney

2 solutions

0 pending reports