Geometry Question by Mithil Shah # 1
Two sides of a triangle are 3 cms and 2 cms. The medians to these sides are perpendicular to each other. Find the third side. If the answer is x , then find the value of x .
The answer is 1.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
A slightly different method from @Marta Reece :
a 2 + 4 b 2 = 4 3
b 2 + 4 a 2 = 2 1
Upon adding the two equations,
5 ( a 2 + b 2 ) = 4 5
The desired result is:
4 ( a 2 + b 2 ) = 5 4 × 4 5 = 1 = 1
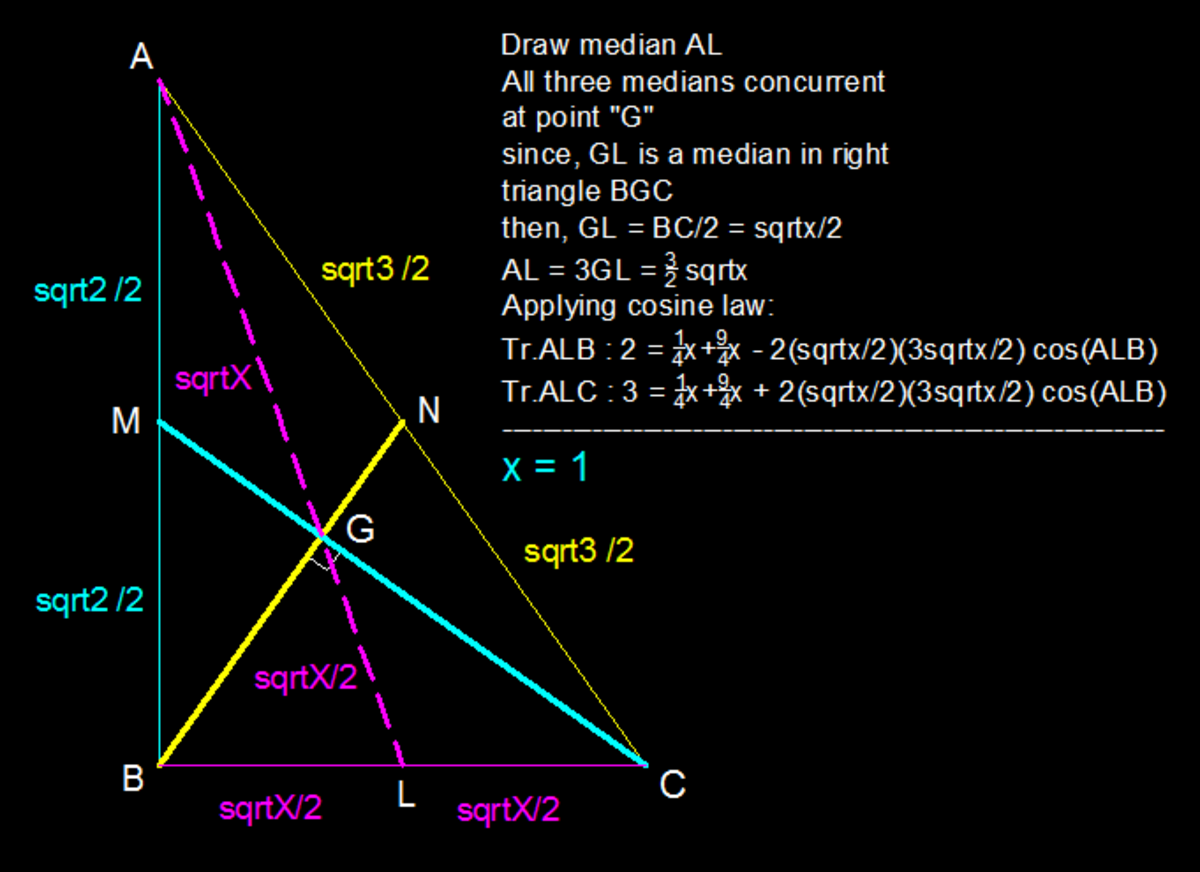
b 2 + 4 a 2 = 2 1
4 b 2 + a 2 = 4 3
Solving these leads to: a 2 = 6 1 , b 2 = 1 2 1
Remaining side is from the pink triangle 4 a 2 + 4 b 2 = 4 × ( 6 1 + 1 2 1 ) = 1