A geometry test #3
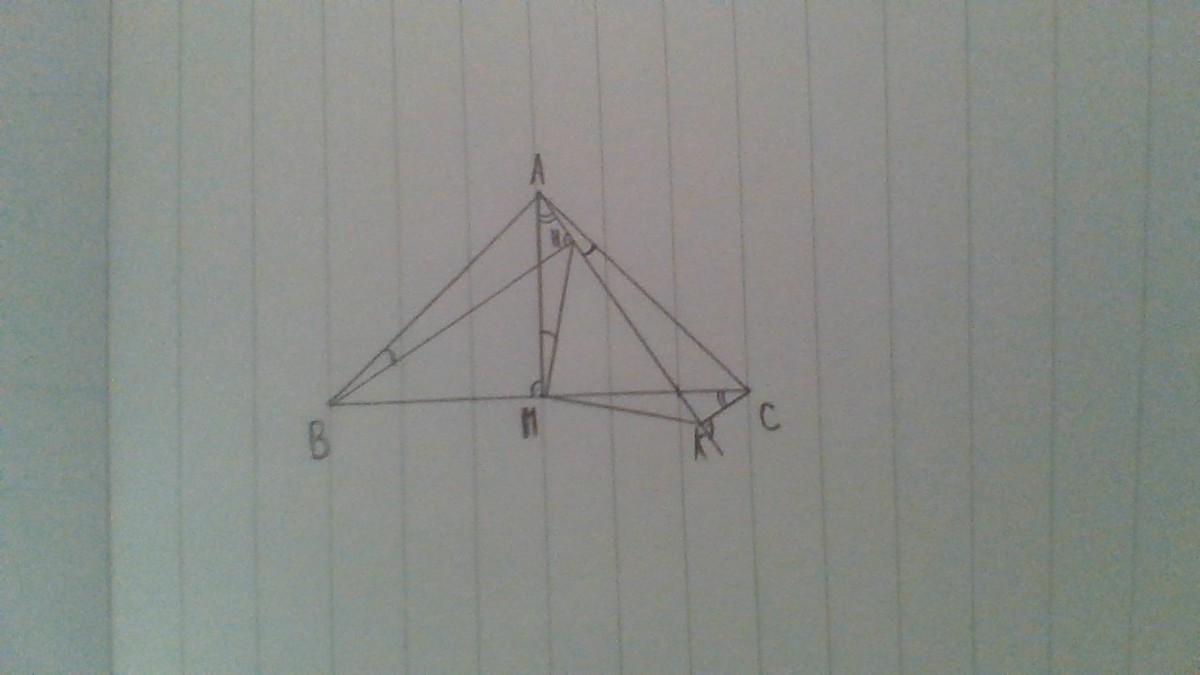
Given an isosceles right triangle ABC (BC is the hypotenuse). Draw M on BC so that AM is a median line. Draw E on BC (E mustn't overlap B, M or C). Draw BH and CK perpendicular to AE at H and K. Find H M K in degrees.
The answer is 90.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Actually all these long solutions are unnecessary if you note that Quad ACMK is concyclic since / CKA=/ CMA both being 90° and hence / MKH=/ ACM=45°. Similarly, Quad ABHM is concyclic and therefore / MHA=/ MBA=45°. So /_HMK=90°. QED.
 According to Thales's theorem quadrilateral AHMB is cyclic. And because it is cyclic we can use Inscribed angle theorem to show that angles ABH and AMH are congruent. And by calculating the value of the angle KAC we can easly conclude that it is equal to the value of the angle ABH. And because the triangle ABC is isosceles we know that |AB|=|AC| so triangles ABH and AKC are congruent (same angles and same hypotenuse), by this we can know that |AH|=|CK|. And again because the triangle ABC is right and isosceles we know that |AM|=|MC|. Now because triangles ABH and AKC are congruent we know that angles ACK and BAH are congruent, we can calculate from this that angles MAK and MCK are also congruent. And by this information we can conclude that triangles AHM and CKM are congruent so the angles AMH and CMK are also congruent. That means that the angle HMK is only rotated angle AMC, so |HMK|=90°.
According to Thales's theorem quadrilateral AHMB is cyclic. And because it is cyclic we can use Inscribed angle theorem to show that angles ABH and AMH are congruent. And by calculating the value of the angle KAC we can easly conclude that it is equal to the value of the angle ABH. And because the triangle ABC is isosceles we know that |AB|=|AC| so triangles ABH and AKC are congruent (same angles and same hypotenuse), by this we can know that |AH|=|CK|. And again because the triangle ABC is right and isosceles we know that |AM|=|MC|. Now because triangles ABH and AKC are congruent we know that angles ACK and BAH are congruent, we can calculate from this that angles MAK and MCK are also congruent. And by this information we can conclude that triangles AHM and CKM are congruent so the angles AMH and CMK are also congruent. That means that the angle HMK is only rotated angle AMC, so |HMK|=90°.
Place △ A B C on a coordinate plane so that A is at the origin, B is on the y -axis at ( b , 0 ) , and C is on the x -axis at ( 0 , b ) .
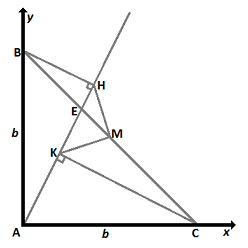
The midpoint of B C is M ( 2 b , 2 b ) .
Let A E have the equation y = m x . Since B H is perpendicular to y = m x and through ( b , 0 ) it has the equation y = − m 1 x + b and intersects y = m x at H ( m 2 + 1 b m , m 2 + 1 b m 2 ) , and since C K is perpendicular to y = m x and through ( 0 , b ) it has the equation y = − m 1 x + m b and intersects y = m x at K ( m 2 + 1 b , m 2 + 1 b m ) .
The slope of H M is m H M = 2 b − m 2 + 1 b m 2 b − m 2 + 1 b m 2 = − ( m − 1 ) ( m + 1 ) , and the slope of K M is m K M = 2 b − m 2 + 1 b 2 b − m 2 + 1 b m = ( m + 1 ) ( m − 1 ) . Since m H M m K M = − ( m − 1 ) ( m + 1 ) ⋅ ( m + 1 ) ( m − 1 ) = − 1 , H M and K M are perpendicular, so ∠ H M K = 9 0 ° .
David, nice solution; I did it the same way, but the product of slopes just missed being -1;
Dave, in your diagram, I let point H have coordinates (x 0,y 0). The slope of MH is (b/2 - y 0)/(b/2 - x 0). I calculated the coordinates of point K by taking the intersection of lines AH and CK, then computed the slope of MK. I won't waste your time on the details, but the product of the two slopes would be -1 if two terms had an opposite sign. I think the method is OK, but obviously there is a mistake . Thanks for your interest.
AM is the median line to BC, the angle bisector of B A C and the perpendicular line to BC.
A B C = A C B = 4 5 o
The median line AM is half the hypotenuse BC. Therefore, A M = B M = C M .
Therefore, B A M = C A M = 2 A B C = 2 9 0 o = 4 5 o .
We can clearly see that M A D + A D M = 9 0 o (Since AMD is a right triangle) and H B D + H D B = 9 0 o (Since HBD is a right triangle).
Therefore, M A D + A D M = H B D + H D B . As A D M and H D B are vertically opposite angles, H D B = A D M .
Hence, M A D = H B D .
We see that C A K = C A M + D A M = 4 5 o + D A M and A B H = A B C + D B H = 4 5 o + D B H .
Since M A D = H B D , C A K = A B H .
Hence we can deduce that 2 right triangles AKC and BHK are congruent (Hypotenuse-Acute Angle).
Therefore, A K = B H .
We can also deduce that 2 triangles AKM and BHM are congruent (Side-Angle-Side). Therefore A M K = H M D .
We can see that: A M K and K M D are complementary angles. Since A M K = H M D , K M D and K M D are complementary angles, which also means that H M K = 9 0 o