A Golden Substitution

What I like about this is that you don't have to solve the quadratic! In fact, notice that by substitution: $\frac{1}{x+1} = \frac{1}{x^2}$ due to the given fact.

Now simplify: $\frac{1}{x} + \frac{1}{x+1} \\ = \frac{1}{x} + \frac{1}{x^2} \\ = \frac{x+1}{x^2}$

But remember that $x^2 = x+1$ , so $\frac{x+1}{x^2} = \frac{x^2}{x^2} = \boxed{1}$

It is given that: $x^2 = x+1$ . Dividing throughout by $x^2$ , we have $1=\dfrac{1}{x}+\dfrac {1}{x^2}$ . Since $x^2 = x+1$ , then $\dfrac{1}{x}+\dfrac {1}{x+1} = \dfrac{1}{x}+\dfrac {1}{x^2} = \boxed{1}$ .

$x^2 = x + 1 \\ \Rightarrow \frac{1}{x} + \frac{1}{x+1} = \frac{1}{x} + \frac{1}{x^2} \\ = \frac{x+1}{x^2} \\ = \frac{x^2}{x^2} \\ = \boxed{1}$ This doesn't account for the possibilities $x=0$ or $x=-1,$ so we need to check if they satisfy the original equation: $0^2 \neq 0 + 1 \\ (-1)^2 \neq -1 + 1$

Just look at the question you will get the answer.

When we proceed for (1÷x) + [1÷(x+1)], we get (2x + 1)÷(x^2 + x). Substituting numerator x + x + 1, we get x + x^2 {from given equation}. Thus numerator and denominator cancel each other out and we obtain result as 1.

Just simply take the LCM, 1/x + 1/(x+1) = (x+1+x)/x(x+1) = ( x^2 +x )/ x.x^2 = x(x +1)/ x.x^2 = 1

x²=x+1.now,x²-x=1.now,x(x-1)=1,now,x-1=1/x,now substituting in 1/x +1/x+1. We do ,(x-1)+1/(x+1). Solving it we get 1

$x^2=x+1 \Rightarrow x=\frac{x+1}{x} \Rightarrow \frac{1}{x}=\frac{x}{x+1} \Rightarrow \frac{1}{x}=\frac{x+1-1}{x+1} \Rightarrow \frac{1}{x}=1-\frac{1}{x+1}$

$\Longrightarrow \frac{1}{x} + \frac{1}{x+1} = 1$

x^{2}=x+1 -> x^{2}-x=1 -> x(x-1)= thus, x=1

Here is what I did

1/x + 1/(x + 1) = 1/x + 1/x^2 = (x + 1)/x^2 = 1

This is the same as 1/x + 1/x^2 or x/x^2 + 1/x^2 or (x+1)/x^2 or x^2 over x^2 which is 1.

Well first I modified the first equation and factored it

I got these results. I substituted -1 to x in the expression, however one of the denominators would be 0 making it undefined. So I substituted 2 to the expression and got this:

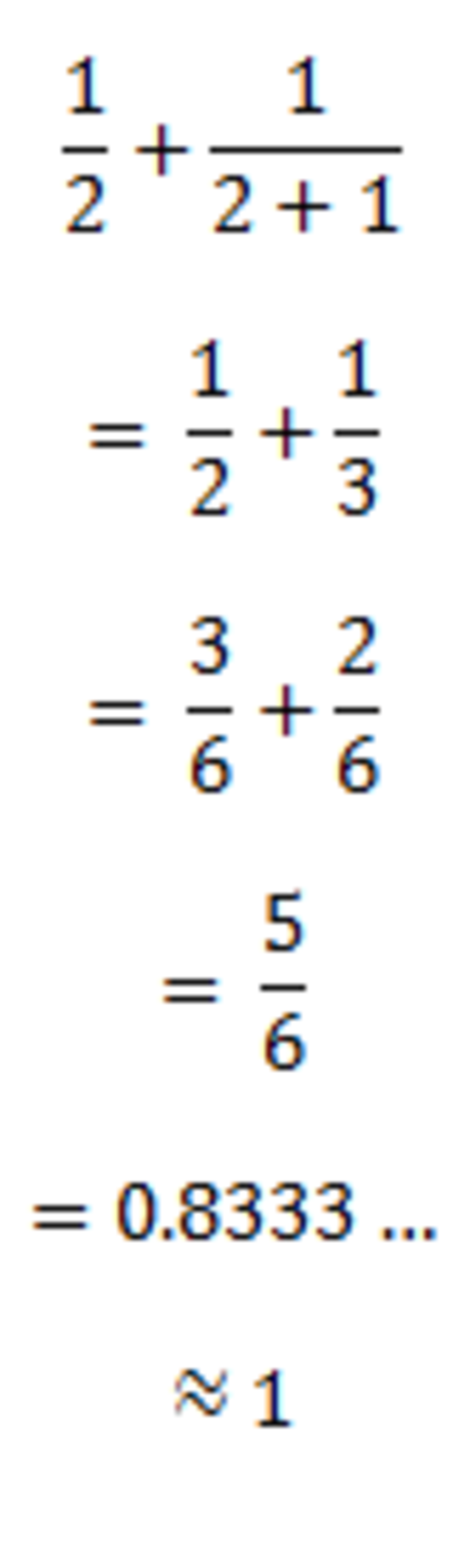
Since the answer should be an integer, I rounded it up and answered 1 rather than 0.83333.....

The answer is 1.

If x^2 = x + 1, then x^2 + x = x + 1 + x <=> x^2 + x = 2x + 1

So:

1/x + 1/(x + 1) = (x + 1 + x)/(x^2 + x) = (2x + 1)/(x^2 + x) =

= (2x + 1)/(2x + 1) = 1

What i did is to find like denominators, first. This obtains $\displaystyle\frac{2x+1}{x^{2}+x}$ If you add x to both sides of the given, you get that $x^{2} + x = 2x + 1$ Thus, the answer is 1.

Since $x^2=x+1, x^2+x=2x+1$ .

$\dfrac{1}{x}+\dfrac{1}{x+1} = \dfrac{2x+1}{x^2+x}=\dfrac{2x+1}{2x+1}=1.$