An Grid of Pieces

Dan and Sam play a game on a grid, on which each one chooses and puts, in his turn, a single piece like these:

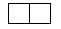
The pieces must not overlap and can't be partially outside of the grid.
The game finishes when someone can't put a piece on the board in his turn following the rules (who is the loser). If Dan begins, who will win? This means, who has a winning strategy?
This is the tenth problem of the set Winning Strategies .
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Every time Dan puts down a piece, Sam will put down a piece that is 180 ∘ away (rotating about the middle of the square). Since the board has even dimensions, by symmetry this will always be possible if Dan's move was possible. Therefore, Sam wins.