A long shot
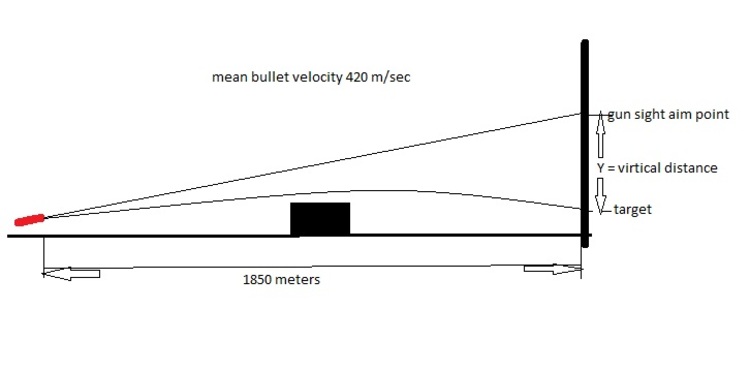 A marksman is attempting to hit a target that he cannot see, because of an obstacle blocking his view. He knows the target is at the same elevation and
meters from his location. He also knows that the target is on a vertical wall. With his high power gun site he can see a vertical line extending upward on the wall. He knows the target is on that line. He can see marking or features on the wall that enable him to determine the vertical distance above the target.
A marksman is attempting to hit a target that he cannot see, because of an obstacle blocking his view. He knows the target is at the same elevation and
meters from his location. He also knows that the target is on a vertical wall. With his high power gun site he can see a vertical line extending upward on the wall. He knows the target is on that line. He can see marking or features on the wall that enable him to determine the vertical distance above the target.
What is the vertical distance above the target where he should aim in order to hit the target? Give your answer in meters.
Details and Assumptions
-
The mean horizontal speed of the bullet is 420 m/s.
-
The gravitational acceleration is
-
The gun site is set parallel to the guns barrel for the shot.
The answer is 95.07.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
time of flight of the shot 4 2 0 1 8 5 0 = 4 . 4 0 4 8 sec
bullet drop do to gravity in 4.405 sec
2 ( 4 . 4 0 5 ) 2 ∗ 9 . 8 = 9 5 . 0 7 meter.