This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
31 solutions
Very nice... (y)
Omg I had to do a long polynomial to get 23.0004. Your way is much quicker Wow!!!
that is perfect
Elegant solution. Love it
Exact same thing that I did!
love the creativity
A really nice solution you found!
let x = a , x 1 = b ,
then a b = 1
a + b = 5
( a + b ) 2 = 5 2
a 2 + b 2 + 2 a b = 2 5
a 2 + b 2 + 2 = 2 5
a 2 + b 2 = 2 5 − 2
a 2 + b 2 = 2 3
x 2 + x 2 1 = 2 3
This was very clear to me. Thanks.
Log in to reply
@Michael Drum @Tahera Faruque you're welcome
Very well explained.
Why a+b = 1?
Thanks you So much for making too easy!!!
On squaring both sides and subtracting 2 from each side...you get the answer to be 23!!
(X+(1/x))²=25 X²+2x×(1/x)+1/x²=25 So, x²+1/x²=23
how? i don't understand?
only squaring both side solve (X+(1/x))²=25 X²+2x×(1/x)+1/x²=25 So, x²+1/x²=23
Where the minus come from
Ok, but WHY to subtract 2? Is it a mathematical property? @Tanya Gupta
Log in to reply
: : let 1/x = y : : (x+y)^2 =5^2 : : x^2 + 2xy + y^2 = 25 : : [using (a+b)^2 = a^2 + 2ab + b^2 ] : : x^2 + 2 . x .1/x + 1/x^2 = 25 : : x^2 + 2 + 1/x^2 = 25 : : x^2 + 1/x^2 = 25 - 2 = 23
Following (a+b)^2 procedure, you will get x^2 + (1/x^2) + 2, on left hand side. 25 will come on right hand side.
25-2 = 23 = x^2 + (1/x^2)
x+1/x = 5 squaring on both sides we get (x^2 + 2x + 1)/x^2 = 25
on subtracting 2 from both sides we get (-x^2 + 2x + 1)/ x^2 = 23
it is asked to find x^2 + 1/x^2 which will be = 16.0625
Log in to reply
Thanks for pointing out the ambiguity in the question. I've updated the question so that it displays x + x 1 instead of "x + 1 / x".
please make me understand. how can u say that??
I get 4.25 in this question.
The answer is false, if you square both sides you can't square x and add to that the square of 1/x, you can only do (x+1/x)^2
Slightly Longer Than Other Answers But Makes More Sense? Rearrange equation to get quadratic. Solve quadratic for x and then put back into equation. Rearrange by moving x across and then multiplying by x X(5-X)=1 multiply out 5X-X^2=1 X^2-5X+1=0 SOLVE PUT X BACK IN
x+ 1/x = 5
squaring both sides
x^2 + 1/x^2 +2 = 25
x^2 + 1/x^2 = 23
i did it exactly the same way
Where did you get the plus 2?
Log in to reply
let use the first equation x +1/x =5, square both sides so
(x+1/x)(x+1/x) = 5 * 5
foil the terms so recall that foil = First, outside, inside, last this results in the following quadratic equation
x^2 +x/x +x/x + 1/x^2 =25 recall that x/x equals 1
reduce x^2 +2 +1/x^2 =25
group like items, in other words move the two to the other side by subtracting from both sides
x^2 +1/x^2 =23
Sorry this is incorrect
Where did the 2 on the left side come from?
x + x 1 = 5 ⟹ ( x + x 1 ) 2 = 2 5 ⟹ x 2 + 2 + x 2 1 = 2 5 ⟹ x 2 + x 2 1 = 2 3
we know that, (a^2+b^2)=(a+b)^2-2ab
so, in this case,x^2+1/x^2=(x+1/x)^2- 2 .x . 1/x
=5^2-2=23
Solution 1
Working on x + x 1 = 5
x + x 1 = 5
( x + x 1 ) 2 = 5 2
We know that, ( a + b ) 2 = a 2 + 2 a b + b 2 so,
x 2 + 2 ⋅ x x 1 + ( x 1 ) 2 = 2 5
x 2 + 2 + x 2 1 = 2 5 ;[ x x 1 is cancelled]
x 2 + x 2 1 = 2 5 − 2
Therefore x 2 + x 2 1 = 2 3
Solution 2
Given that,
- x + x 1 = 5
Now,
( x + x 1 ) 2 − 2 ⋅ x x 1
( 5 ) 2 − 2 ;[ x x 1 is cancelled]
= 2 3
Square both sides so we will get, X^2 +2x1/x+1/x^2=25 Thus. X2+1/x2=25-2=23
x2+1/x2 = (x+1/x)2-2= 25-2=23
Square both side . after Solve the answer is 23
(x+1\x)^2=25 X^2+2 +(1\x^2)=25 X^2+(1\x^2)=23
x^2+1/x^2 =(x+1/x)^2-2.x.1/x =(5)^2-2 =25-2 =23
Here's another way to solve the problem (For those of you who like calculators):
Step 1: Manipulate the first equation into a solvable form:
x + 1/x = 5 -> 1/x = 5 - x -> 1 =x(5-x) -> 1 = 5x - x^2 -> x^2 - 5x + 1 = 0
Step 2: Use the Quadratic Formula to arrive at these answers:
x=(5+sqrt 21)/2, x=(5-sqrt 21)/2
Step 3: Plug in either value of X into the second equation, x^2+1/x^2=?, and you should get 23.
Use solver in excel.
Set A1 to 1. Set A2 to =A1 + (1/A1) Set B2 = A1^2+(1/(A1^2)) Run solver add in, find solution for B1 by manipulating A1. cell B2 has your solution.
Solver has destroyed my paper and pencil algebra ability.
Since; x+1/x =5 Squaring on both the side (x+1/x)² = x²+1/x²+2•x•1/x= 5² Or ; x²+1/x²+2= 25 Or; x²+1/x²= 25-2 =23
On squaring both sides, you get the answer to be 23
See..do (x+1/x)^2.. Exapnd it normally and subtract 2 to get the answer...so according to.our logic... The solution is... (5)^2-2=23
x^2 + (i/x)^2 = (x + 1/x)^2 - 2 = 5^2 - 2 = 23
just use a formula( x+1 upon x) whole square = x square + 1 upon x square. hence, by putting value you will get the answer
square both sides and you're done!
Squaring both sides we get the value of expression in question 25 -2 = 23 Ans K.KL.GARG,India
Square both sides of the equation. After the first method, you will come up with an answer that expresses the arithmetical equation x(squared) + 2 + 1/x(squared) = 25. Simply put 2 to the right side of the equation. Therefore, when 2 is transposed, it will become -2.. There is 25 at the right side of the equation: 25-2=23. I feel apologetic for the solution. I know it's awfully cryptic to decipher well.
( x + x 1 ) 2 = x 2 + 2 + x 2 1
So,
x 2 + x 2 1 = ( x + x 1 ) 2 - 2
x 2 + x 2 1 = 5 2 - 2
x 2 + x 2 1 = 2 5 - 2
x 2 + x 2 1 = 2 3
x + 1/x =5 || (x+1/x)^2 = 5^2 || We know that (a + b)^2 = a^2 + 2ab + b^2 || continuance : x^2 + 2.x.1/x +(1/x)^2 = 25 || x^2 + 2 + 1/x^2 = 25 || x^2 + 1/x^2 = 25-2 || x^2 + 1/x^2 = 23
we actually have 2 solution for this. first undo the 1/x. this mean we have to multiply everything in the equation by x. we will have this x^2+1=5x. now rearrange the equation so it equal to zero. x^2-5x+1=0. now complete the square so we end up with (x-2.5)^2-6.25+1=0. tidy it up and we get (x-2.5)^2-5.2=0 . rearrange the equation again to give (x-2.5)^2=5.25 . this mean we have x-2.5=+ or - square root of 5.25 (which is +-2.29). now the equation is x-2.5=+-2.29 now just take the rearrange to make x= -0.4 or 23
as we know a square + b square = a+b sqaure -2ab, applying this property we get (x + 1/x)square -2 * x* 1/x=25-2=23
squaring both sides. (X+1/X)^2 = (5)^2 ; X^2 + (1/X^2) + 2 = 25 ; X^2 + (1/X^2) = 23
haysss I get 12 in this question
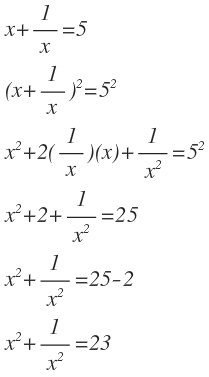
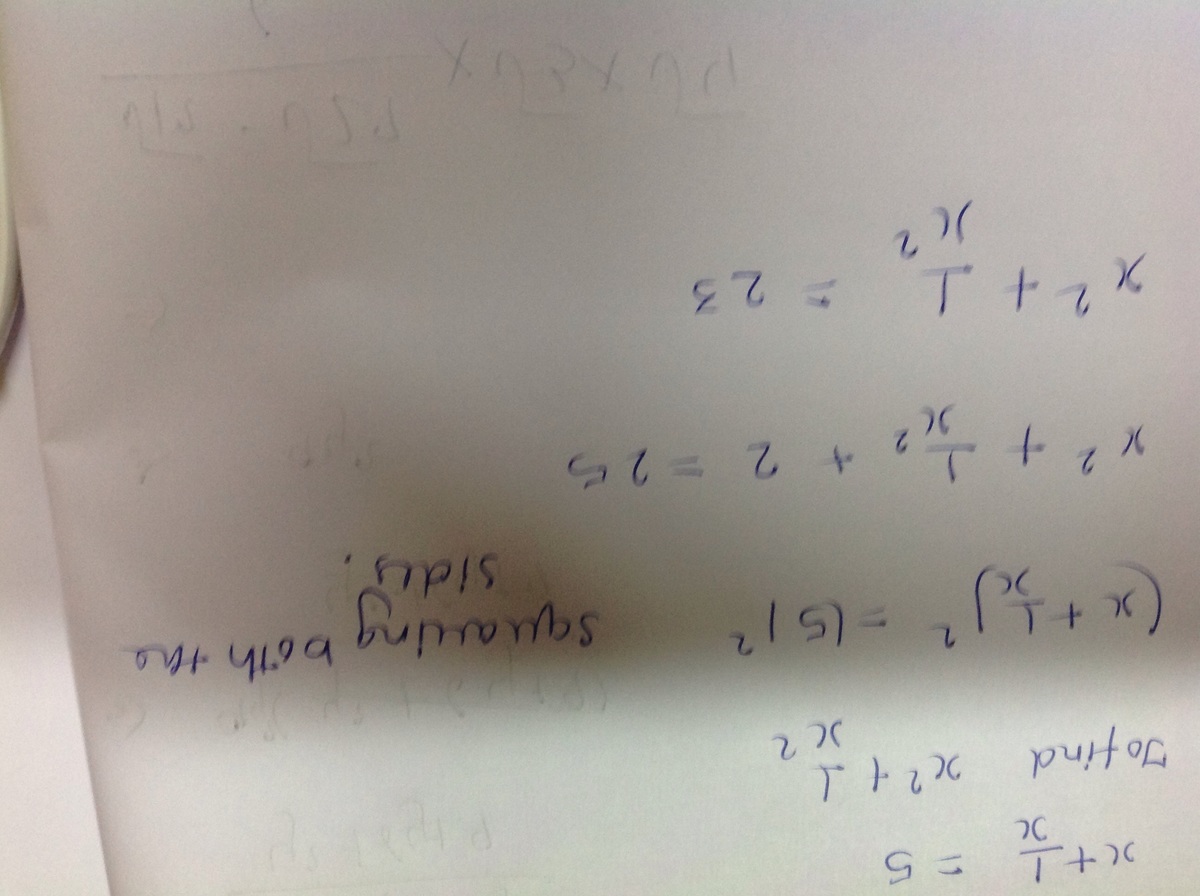
x + x 1 = 5
⇒ ( x + x 1 ) 2 = 5 2
⇒ x 2 + x x + x x + x 2 1 = 5 2
⇒ x 2 + 2 + x 2 1 = 2 5
⇒ x 2 + x 2 1 = 2 5 − 2
⇒ x 2 + x 2 1 = 2 3