Mystery of the square

Probability Level 2

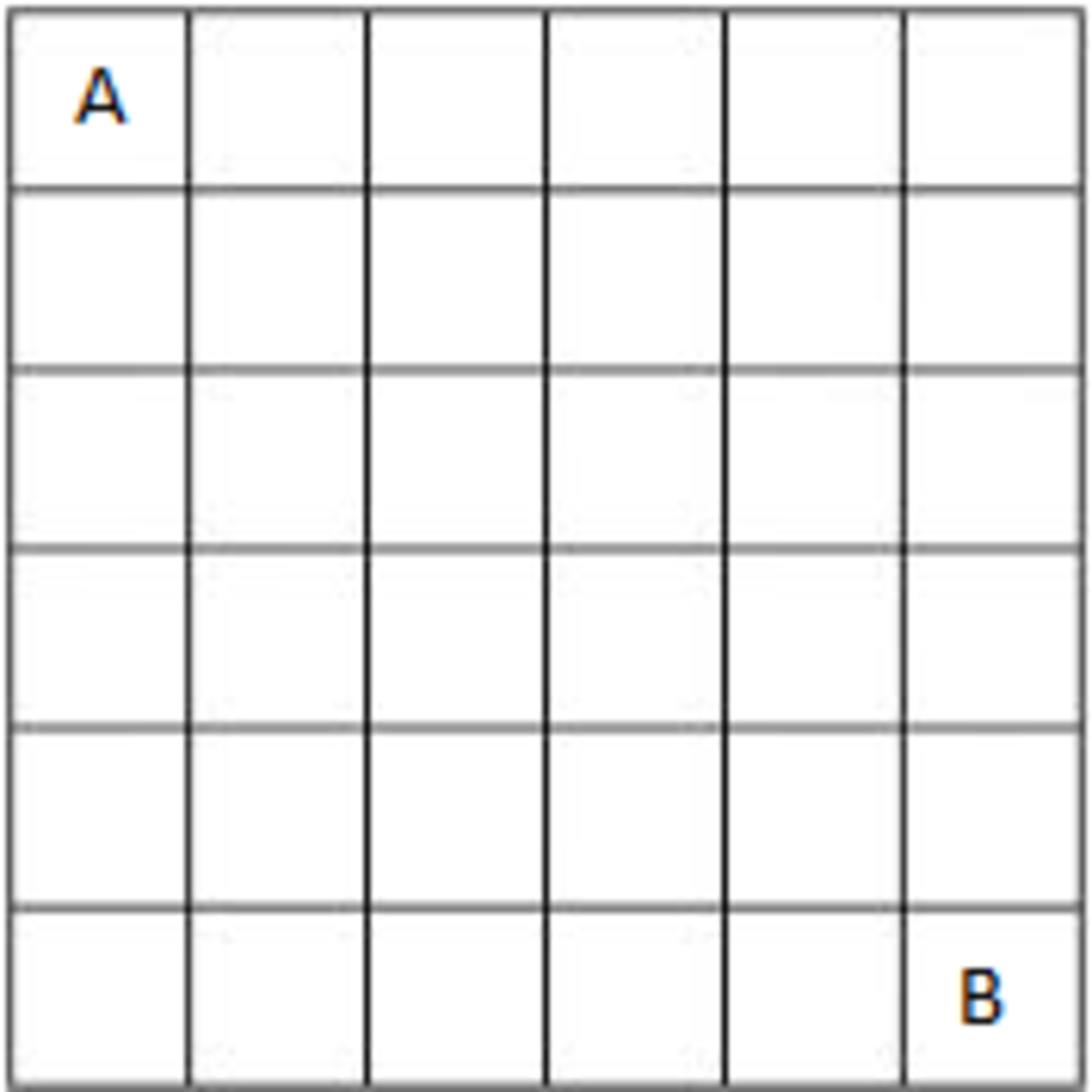
How many ways are there to get from A to B?

Note that

  1. You can only move to the right and down

  2. You can't move to the left and you can't move up

  3. You cannot move diagonally

512 126 504 252

Shravan Kumar by Shravan Kumar , 28, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Yee-Lynn Lee
Sep 6, 2015

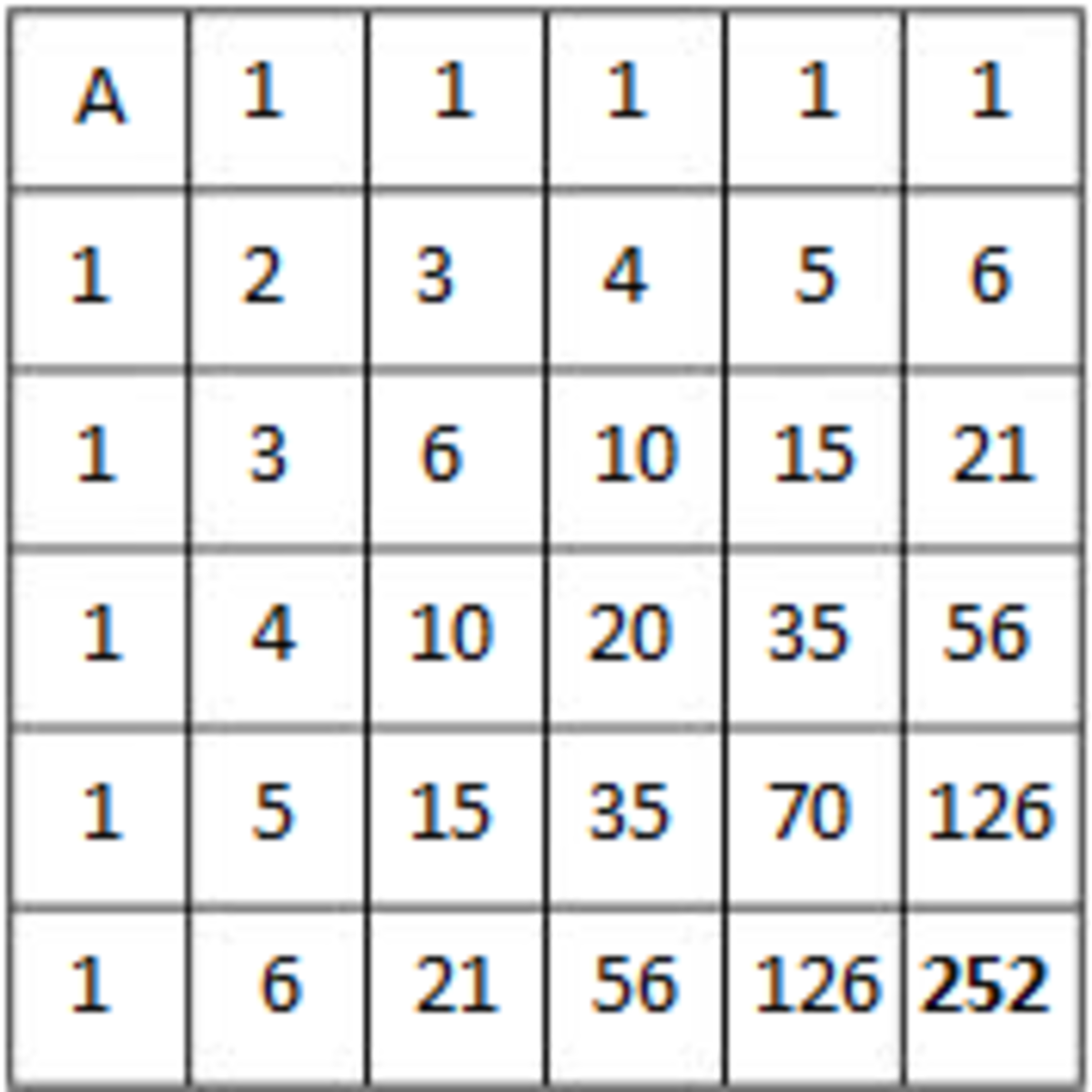
In order to get from A to B, you must move 5 spaces right and 5 spaces down. We "choose" out of 10 choices between down and right which ones will be down.

( 10 5 ) = 252 \begin{pmatrix} 10 \\ 5 \end{pmatrix}=\boxed{252}

1 Helpful 0 Interesting 0 Brilliant 0 Confused

I don't get it.

Francis Dave Cabanting - 5 years, 9 months ago

Log in to reply

To get from A to B, you have to move 10 spaces, five down and five right. Every time you want to move a space, you have to "choose" whether to go right or down. But, you can only "choose" down five times and right five times. Say you order your choices from 1 to 10. Whichever 5 you choose for down, the rest will be right and vice versa. You basically pick 5 numbers out of the numbers 1 to 10, to be your right or your down choices. You can use the binomial coefficient to figure out how many ways you can choose these 5 numbers, and that is your answer. Is this any clearer?

Yee-Lynn Lee - 5 years, 9 months ago
Shravan Kumar
Sep 6, 2015

Since we can move only to the right or down, we can use pascal's triangle.

So by using pascal's triangle we get 252

1 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...