A pentagon inscribed in a square
A regular pentagon is inscribed in a unit square. If the side length of the pentagon is a , then find ⌊ 1 0 5 a ⌋
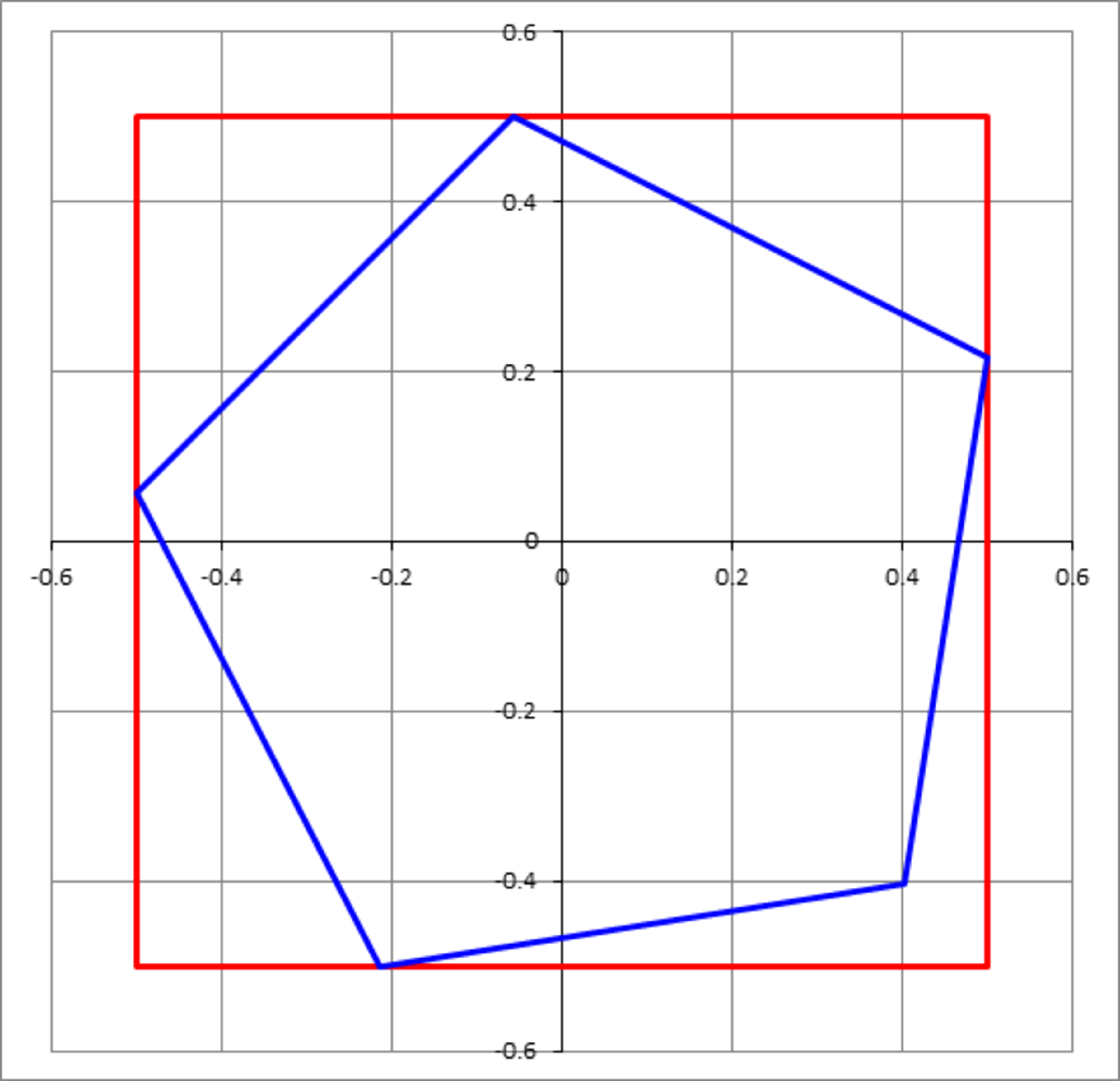
The answer is 62573.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
How do you prove that there is an axis of symmetry?
@Atomsky Jahid I hope my reedited solution gives an answer.
For those who find nested radicals more aesthetic than sin 2 7 ∘ , I'll offer 8 − 2 2 ( 5 + 5 ) ≈ 0 . 6 2 5 7 3 7
A E = 2 a and ∠ A E F = 4 5 ∘ . Since for the angle of the regular pentagon we have ∠ F E G = 1 0 8 ∘ , we get
∠ B E G = 1 8 0 ∘ − ( ∠ A E F + ∠ F E G ) = 1 8 0 ∘ − ( 4 5 ∘ + 1 0 8 ∘ ) = 2 7 ∘ ⇒ E B = a ⋅ cos 2 7 ∘ Consequently,
A E + E B = A B ⇒ 2 a + a ⋅ cos 2 7 ∘ = 1 ⇒ a = 2 1 + cos 2 7 ∘ 1 ⇒ a ≈ 0 . 6 2 5 7 3 7 8 6 For the answer,
⌊ 1 0 5 a ⌋ = 6 2 5 7 3 .
Here is a proof of the fact that △ A E F is an isosceles right triangle, without taking symmetry for granted: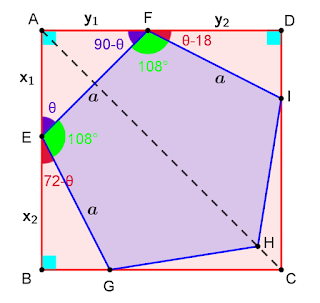 Then,
∠
B
E
G
=
1
8
0
∘
−
(
1
0
8
∘
+
θ
∘
)
=
7
2
∘
−
θ
∘
∠
A
F
E
=
9
0
∘
−
θ
∘
∠
D
F
I
=
1
8
0
∘
−
(
1
0
8
∘
+
9
0
∘
−
θ
∘
)
=
θ
∘
−
1
8
∘
Hence,
x
1
=
a
⋅
cos
(
θ
∘
)
x
2
=
a
⋅
cos
(
7
2
∘
−
θ
∘
)
y
1
=
a
⋅
sin
(
θ
∘
)
y
2
=
a
⋅
cos
(
θ
∘
−
1
8
∘
)
Now, we can construct an equation for
θ
:
Then,
∠
B
E
G
=
1
8
0
∘
−
(
1
0
8
∘
+
θ
∘
)
=
7
2
∘
−
θ
∘
∠
A
F
E
=
9
0
∘
−
θ
∘
∠
D
F
I
=
1
8
0
∘
−
(
1
0
8
∘
+
9
0
∘
−
θ
∘
)
=
θ
∘
−
1
8
∘
Hence,
x
1
=
a
⋅
cos
(
θ
∘
)
x
2
=
a
⋅
cos
(
7
2
∘
−
θ
∘
)
y
1
=
a
⋅
sin
(
θ
∘
)
y
2
=
a
⋅
cos
(
θ
∘
−
1
8
∘
)
Now, we can construct an equation for
θ
:
Let ∠ A E F = θ ∘ , A E = x 1 , E B = x 2 , A F = y 1 , F D = y 2 .
x 1 + x 2 = 1 = y 1 + y 2 ⇔ a ⋅ cos ( θ ∘ ) + a ⋅ cos ( 7 2 ∘ − θ ∘ ) = a ⋅ sin ( θ ∘ ) + a ⋅ cos ( θ ∘ − 1 8 ∘ ) ⇔ cos ( θ ∘ ) + cos ( 7 2 ∘ ) cos ( θ ∘ ) + sin ( 7 2 ∘ ) sin ( θ ∘ ) = sin ( θ ∘ ) + cos ( θ ∘ ) cos ( 1 8 ∘ ) + sin ( θ ∘ ) sin ( 1 8 ∘ ) ⇔ cos ( θ ∘ ) [ 1 + cos ( 7 2 ∘ ) − cos ( 1 8 ∘ ) ] = sin ( θ ∘ ) [ 1 + sin ( 1 8 ∘ ) − sin ( 7 2 ∘ ) ] ⇔ cos ( θ ∘ ) = sin ( θ ∘ ) , since cos ( 7 2 ∘ ) = sin ( 1 8 ∘ ) and cos ( 1 8 ∘ ) = sin ( 7 2 ∘ ) ⇔ tan ( θ ∘ ) = 1 ⇔ θ = 4 5 Hence, △ A E F is an isosceles right triangle.
Note: @Atomsky Jahid This last statement implies that A C is, indeed, an axis of symmetry of the compound shape.