A Pre-RMO question! -12
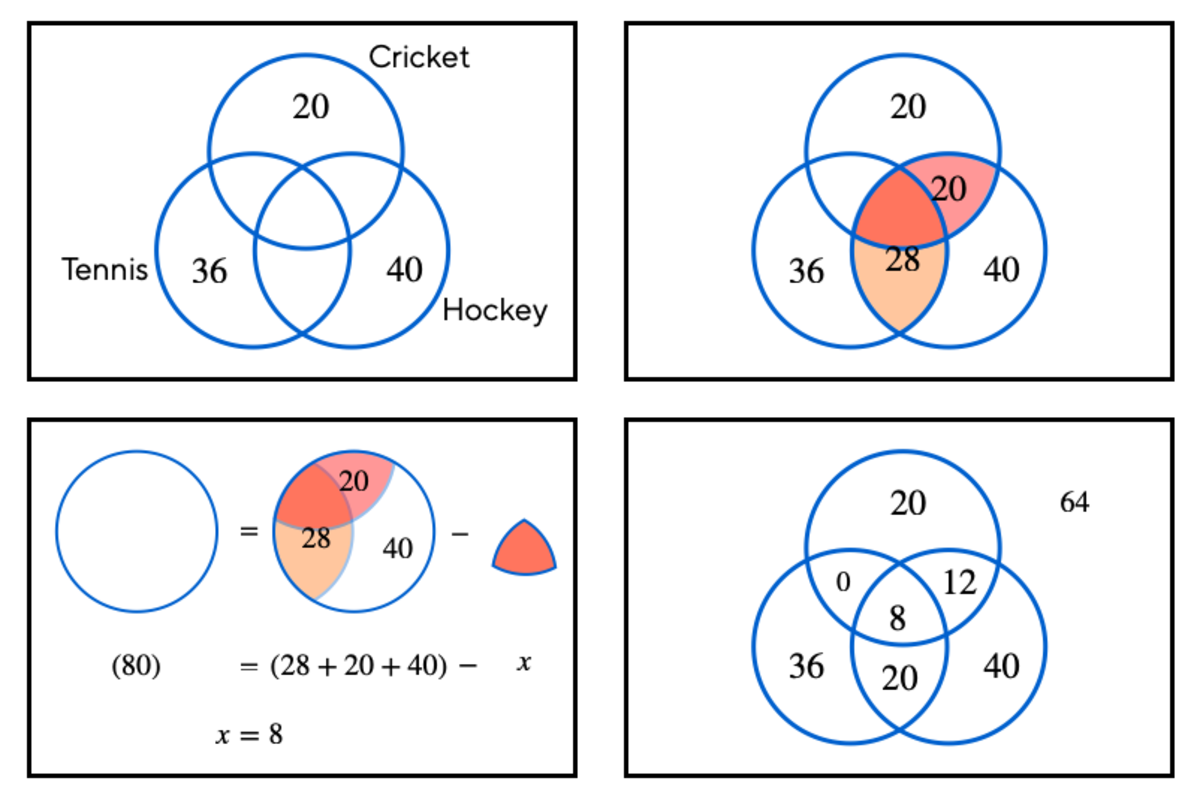
In a group of 2 0 0 students, 2 0 played cricket only, 3 6 played tennis only, 4 0 played hockey only, 8 played cricket and tennis, 2 0 played cricket and hockey, 2 8 played hockey and tennis and 80 played hockey.
Find the sum of number of students who did not played any of the games and number of students who only played hockey and cricket.
The answer is 76.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Thus the required answer is
6 4 + 1 2 = 7 6
Damn this one's a hard one
Let the number of students who played none of the games be n , played cricket and hockey only be x , played cricket and tennis only be y , played hockey and tennis only be u , and played all the games be z .
Then
4 0 + 2 0 + 3 6 + x + y + z + u + n = 2 0 0
⟹ x + y + z + u + n = 1 0 4 ,
x + z = 2 0 ,
y + z = 8 ,
u + z = 2 8 ,
4 0 + x + z + u = 8 0 ⟹ x + z + u = 4 0 ,
y + n = 6 4 ,
x − y = 1 2
⟹ x + n = 1 2 + y + n = 1 2 + 6 4 = 7 6 .
Hence the required answer is 7 6 .
Label the unknowns as in the Venn diagram above. Then we have:
⎩ ⎪ ⎪ ⎪ ⎨ ⎪ ⎪ ⎪ ⎧ a + b = 8 a + d = 2 0 a + c = 2 8 a + c + d = 4 0 . . . ( 1 ) . . . ( 2 ) . . . ( 3 ) . . . ( 4 )
From ( 4 ) − ( 3 ) : ⟹ d = 1 2 , ( 2 ) : ⟹ a = 8 , ( 1 ) : ⟹ b = 0 , ( 3 ) : ⟹ c = 2 0 , and f = 2 0 0 − 2 0 − 3 6 − 4 0 − a − b − c − d = 6 4 . The sum of numbers who played no game and only played cricket and hockey is f + d = 6 4 + 1 2 = 7 6 .