A geometry problem by Akhitha Babu

Geometry Level 2

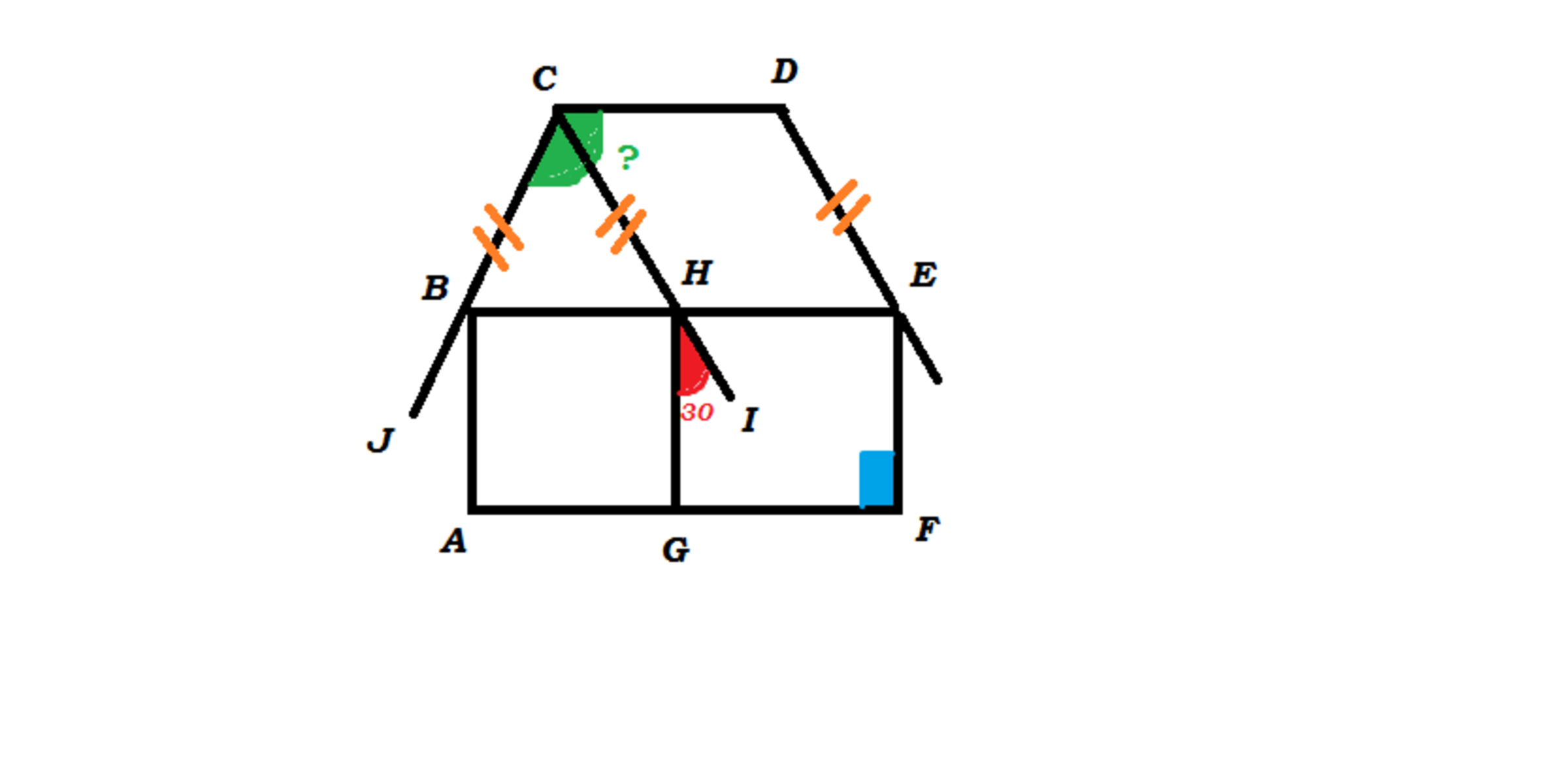
Lines $AB, GH$ and $EF$ are parallel to each other. Lines $GF, BH$ and $CD$ are also parallel to each other. Also the lines $BC, CH$ and $DE$ are equal in length. Lines $GF$ and $EF$ are perpendicular to each other. If $\angle GHI= 30^\circ$ , what is $\angle JCD$ ?

60 90 80 150 120

1 solution

Akhitha Babu
Sep 16, 2016

Given AB|| GH|| EF .
And GF|| BH|| CD.
This implies AF|| BE|| CD.
Also given BC= CH= DE.
This implies <CBH= <CHB= <DEH.
Also given GF and EF are perpendicular to each other.
This implies AB, GH and EF are perpendicular to AF.
ie, <ABG= <BHG= <HGA= <GAB=<GHE= <HEF= <EFG= <FGH= 90 degree.
Therefore, <CBA= <CHG= <DEF
We know that
<GHI + <BHG + <CHB = 180 degree
30 + 90 + <CHB = 180.
<CHB= 180-30-90= 60 degree.
This implies <CBH= <CHB= <DEH = 60 degree.
ie, CBH is an equilateral traingle.
Thus <BCH= <HBC= <CHB= 60 degree.
Since BCDE is a quadrilateral,
<CBH+ <BCD = 180 degree.
90 + <JCD = 180.
Therefore <JCD= 180-90 =120 degree

A geometry problem by Akhitha Babu

1 solution

0 pending reports