Middle man
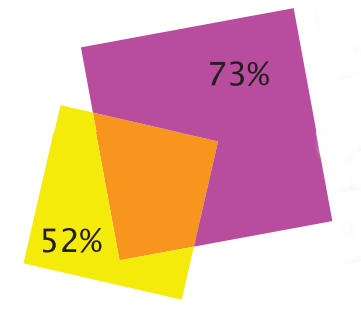 Diagram above shows two pieces of overlapping paper. The non-overlapping area of the smaller square corresponds to 52% of the area of this square and non-overlapping area of the larger square is 73% of the area of this square. What is the ratio between the sides of the smaller square and the largest square?
Diagram above shows two pieces of overlapping paper. The non-overlapping area of the smaller square corresponds to 52% of the area of this square and non-overlapping area of the larger square is 73% of the area of this square. What is the ratio between the sides of the smaller square and the largest square?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Note that 4 8 % of the area of the smaller square is equivalent to 2 7 % of the area of the larger square. Thus, the total areas must be in a 4 8 1 0 0 : 2 7 1 0 0 = 2 7 : 4 8 ratio. The ratio of the side lengths is thus 4 8 2 7 = 4 3 .