Concentric Circle Area Ratios
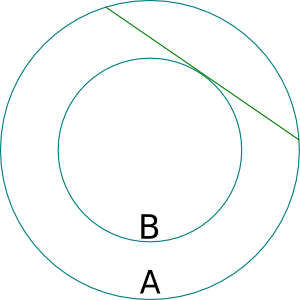 Circle A and circle B are concentric. A chord of circle A that is tangent to circle B has a length that is twice the radius of circle B. What is the ratio of the area of circle A to the area circle B?
Circle A and circle B are concentric. A chord of circle A that is tangent to circle B has a length that is twice the radius of circle B. What is the ratio of the area of circle A to the area circle B?
The answer is 2.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
15 solutions
i can't understand..
yes 2:1 is the right answer
A : B
pi*r^2 : pi*r^2
pi*2(r^2) : pi*r^2 // Circle A's radius is twice as B
2 : 1 // which yields 2 : 1 at the end.
Log in to reply
yes
okk...
better
yea ratio A:B=2:1
so intelligent.... :)
Yes
thanks
thanks for the solution
tB {0
P_{0}
when its area then its 4:1
Let radius of circle B be 'r'. So length of chord is 2r. Perpendicular from center of the circles bisects the chord.Therefore By using Area of Circle I solve this problem.
Get a life
Let radius of circle B be 'r'. So length of chord is 2r. Perpendicular from center of the circles bisects the chord. By Pythagoras theorem, radius of the circle A will be (\sqrt{2})r. Area of circle A is Pi2rr and area of circle B is Pir*r. Thus ratio of area of circle A to area of circle B is 2:1
Hooh.. Just 2/1! Cancel pi, and radius then the quotient is 2! It sucks!
Small Circle Radius = r Large Circle Radius = R Tangent Chord Length = d
We are told: 2r = d
(if you are doodling, draw a line from the center to the point the tangent line intersects, then another line from the center to a point that line intersects the larger circle)
R = √( r^2 + (d/2)^2 )
* d/2 since we only care about half of the chord (the hypotenuse of the triangle we drew)
R = √( r^2 + (2r/2)^2 ) -----> R = √( r^2 + r^2 )
*
since 2r = d, so d/2 = r
R = √(2r^2) -----> R = r *√(2)
π r^2 : π R^2 -----( R = r √2 )------> π r^2 : π (r√2)^2
-------> π r^2 : 2π r^2
-------> 1:2
2:1 is the right answer.....!!!!
suppose chord is KN....... bt it is gvn dat KN=2R where R be the radius of the circle B As chrd to circle A is tangent to the B hence it ll mek rt L triangle.CTN in which CN is radius of circle A
from P.Thm. CN=(R^.5+R^.5) area will be fr crcle A=2(pi)R^(2) and for crcle B=(pi)R^(2) Hence ratio=2
easily solv by usig pythagoras
buy using pythagoras ? how ?
since, the chord of circle A (C 1) is tang. to circle B so radius at its pt. of contact is its perpendicular bisector .Using Pythagoras theorem in the triangle formed by radius of both circles and half the chord ,find radius of (C 1) and then the ratio of the area of circle A to the area circle B.
The radius of Circle B forms an isosceles triangle, and use Pythagoras Theorem. Then find ratio by comparing their areas with Area Formula of Circle.
Assume area of the inner circle is "r". Area of Circle B = pi*(r^2) center point of circle is C Assume tangent is XY touching circle B at point M draw a perpendicular to a point where tangent is touching the inner circle On circle B with the center, i.e perpendicular to line XY at M. join MC and XC. where XC is radius of Circle A.
by Pythagoras thesis, xc^2 =XM^2 + XC^2 X = root(2)*r hence ratio of Area of circles = 2
Now we need to find size of hypotenuse of a triangle formed y
How ? All Circle Have 1 Unit .. Therefore Circle A And Circle Have The Same Unit ..
radius of circle B = X(let) then radius of circle A is \sqrt{2}*X Area's ratio= 2:1
The chord is tangent to the radius and is twice as long as the radius. Because the circles are concentric, the radius is tangent to the chord at its middle. If the median of an interval is equal to its half, they form a right triangle, and thus the central angle supported by the chord is of 90 degrees. The inscribed angle supported by a chord is worth half the central angle supported by the chord and thus is 45 degrees, and the inscribed angle on the opposite side is worth 180 degrees minus the inscribed angle, thus 135 degrees. Half of this inscribed angle is worth 67.5 degrees. If we continue the raduis beyond the chord till it meets with the outer circle and forms its radius, and then connect the end of the radius with the meeting point of the chord and the outer circle, we get a right triangle with angles of 67.5, 90 and 22.5. Let the radius of B be r and the radius of A be R. \frac{r}{(R-r)}=tan67.5= 2.414 r= 2.414 * (R-r) // divide by 2.414 R-r= \frac{r}{2.414}= 0.414 * r
R= 1.414 * r= \sqrt{2} * r R^2= 2 * r^2
that's it
Construct a line segment from the center of the circles to the middle of the chord, this is the radius of the smaller circle.. Then construct line segments from the center of the circles to the ends of the chord, these are the radii of the larger circle. The triangle formed by the radius of the small circle, the radius of the large circle, and half the chord is isosceles with the two equal line segments the radius of the small circle and half the chord. Using the Pythagorean theorem the length of the third side, the radius of the large circle, is square root of 2 times the length of the radius of the small circle. Therefore, if the radius of the small circle is x then it's area is x squared times pi. The area of the large circle is 2 times x squared times pi. The ratio of the area of the large circle to the small circle is 2.
Can you not
As I read this again and the others I realized that I did not establish that the triangle was a RIGHT isosceles triangle.
Let radius of circle B be 'r'. So length of chord is 2r. Perpendicular from center of the circles bisects the chord. By Pythagoras theorem, radius of the circle A will be (\sqrt{2})r. Area of circle A is Pi 2 r r and area of circle B is Pi r*r. Thus ratio of area of circle A to area of circle B is 2:1