The Sufficient Sums
The sum of the first three terms of a geometric progression is 8. The sum of the first six terms of the same geometric progression is 12.
Find the common ratio of this geometric progression.
Express your answer in two decimal places
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
5 solutions
Correct! Though we can further reduce the calculation if we just do the following:
a ( 1 + r + r 2 ) a r 3 ( 1 + r + r 2 ) = 8 4 ⇒ r 3 = 2 1 .
Thanks! ⌣ ¨
The sum of the first n -terms of a geometric progression is S n = r − 1 a ( r n − 1 ) or S n = 1 − r a ( 1 − r n ) , where a is the first term of the GP, r be the common ratio, S n be the sum of the first n -terms of a GP.
Given that S 3 = r − 1 a ( r 3 − 1 ) = 8 . . . ( 1 )
S 6 = r − 1 a ( r 6 − 1 ) = 1 2 . . . ( 2 )
( 1 ) : ( 2 ) gives r 6 − 1 r 3 − 1 = 3 2
By factoring and dividing, we have
r 3 + 1 1 = 3 2
r 3 + 1 = 2 3
r 3 = 2 1
r = 3 2 1 ≈ 0 . 7 9
Case 1
:-
Let the numbers be
r
a
,
a
and
a
r
r
a
+
a
+
a
r
=
8
r
a
+
a
r
+
a
r
2
=
8
a
(
1
+
r
+
r
2
)
=
8
r
Let
(
1
+
r
+
r
2
)
be
x
∴
a
x
=
8
r
a
=
x
8
r
→
1
Case 2
:-
Let the numbers be
r
2
a
,
r
a
,
a
,
a
r
,
a
r
2
and
a
r
3
r
2
a
+
r
a
+
a
+
a
r
+
a
r
2
+
a
r
3
= 12)
r
2
a
+
a
r
+
a
r
2
+
a
r
3
+
a
r
4
+
a
r
5
=
1
2
a
(
1
+
r
+
r
2
+
r
3
+
r
4
+
r
5
)
=
1
2
r
2
Substituting the value of
x
we get:-
a
(
x
+
x
r
3
)
=
1
2
r
2
a
=
x
(
1
+
r
3
)
1
2
r
2
→
2
From
1
and
2
we get:-
x
8
r
=
x
(
1
+
r
3
)
1
2
r
2
2
+
2
r
3
=
3
2
r
3
=
1
r
3
=
2
1
r
=
3
2
1
r
≈
0
.
7
9
.
a(r^3-1)/(r-1)=8 ---------(1) a(r^6-1)/(r-1)=12------(2) Simplifying L.H.S of eq(2) we get a{(r^3-1)(r^3+1)/(r-1)}=8(r^3+1)=12 r^3= (12/8)-1=0.5 Now r = cubroot of 0.5 =0.79
Cheers!
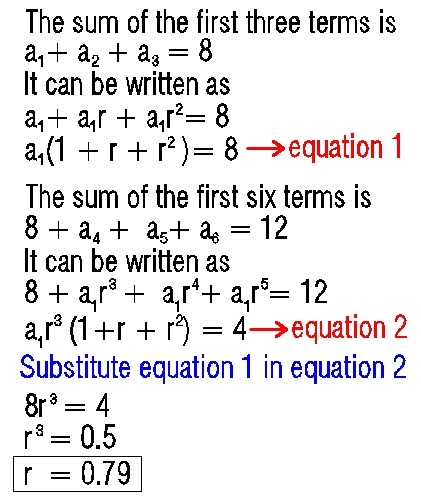
Let r = common ratio and a = first term
Sum of first 3 terms = a + a r + a r^2 = 8
a * (1 + r + r^2) = 8 => 1 + r + r^2 = 8/a
Sum of first 6 terms = a + a r + a r^2 + a r^3 + a r^4 + a*r^5 = 12
a * (1 + r + r^2) + a * (r^3 + r^4 + r^5) = 12
Since first term is = 8, then a * (r^3 + r^4 + r^5) = 4
a * r^3 * ( 1 + r + r^2) = 4
a * r^3 * (8/a) = 4
r^3 * 8 = 4
r^3 = 0.5
r = 0.79