A Projection Of Circular Proportions!

Classical Mechanics Level 5

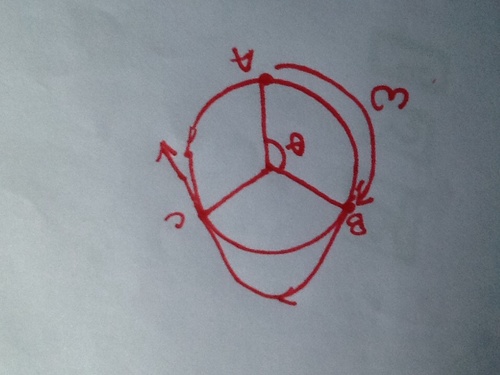Consider a circle of radius 50 units, with a point sized object affixed to it at $A$ . The circle rotates with angular velocity $\omega$ , through an angle $\theta$ , when, after a time $t$ , it stops almost immediately. The object is projected should be projected in such a way that it falls tangentially on the other side of the circle, through the horizontal passing through the point of projection. If $\omega =\frac{\pi}{5}$ radians per second, and $g=9.8$ units per second, find the maximum of $t$ , such that the above projection occurs. Round to the nearest hundredth.

The answer is 3.33.

2 solutions

Ronak Agarwal
Jul 27, 2014

I can't exactly understood the term maximum of t meant.But anyway here is my solution. Revolve Revolve We will simply say that the motion of the projectile will be symmetric about the vertical passing through the centre.

$\Rightarrow Half\quad the\quad range=rsin\theta$

Using our formula for range we have :

$rsin\theta =\frac { { v }^{ 2 }sin\theta cos\theta }{ g }$

$\Rightarrow sec\theta =\frac { { v }^{ 2 } }{ Rg }$

$also\quad v=\omega R\quad hence\quad we\quad have\quad v=\frac { \pi }{ 5 } R$

Finally we have $cos\theta =\frac { 25g }{ { \pi }^{ 2 }r }$

Put the values to get $\theta =1.051$

So we get $t=\frac { \pi -1.051 }{ (\frac { \pi }{ 5 } ) } \quad \approx 3.326$

Satvik Pandey
Aug 12, 2014

I think the figure is up side down.

Then too his language is not clear

Ronak Agarwal - 6 years, 10 months ago

But the question was nice.

satvik pandey - 6 years, 10 months ago

A Projection Of Circular Proportions!

The answer is 3.33.

2 solutions

0 pending reports