A rod near a point charge
A point charge of magnitude 1 mC is brought to a distance of 1 cm from the end of a horizontal, one-dimensional uniformly charged non-conducting rod of length 10 cm. The point charge is on the axis of the rod. If the net horizontal force on the rod is 10 N, what is the charge of the rod in nano-Coulombs ?
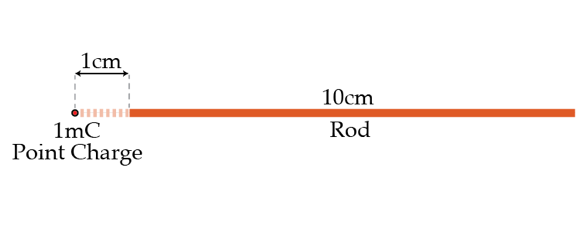
Details and assumptions
- You may take k to be .
The answer is 1.22.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The net force on the rod is given by an integral of the forces on infinitesimal sections of the rod, i.e.
F = ∫ 0 L k q ( L + d − x ) 2 λ d x
where L is the length of the rod, d is the distance the point charge is from the rod, q is the magnitude of the point charge, and λ is the linear charge density on the rod. We can explicitly evaluate this integral to get
F = ( L + d ) d k q L λ = ( L + d ) d k q Q
where Q is the total charge of the rod. We can then solve this for Q = 1 . 2 2 nC .