A rose by any other name
 The collection of polar graphs,
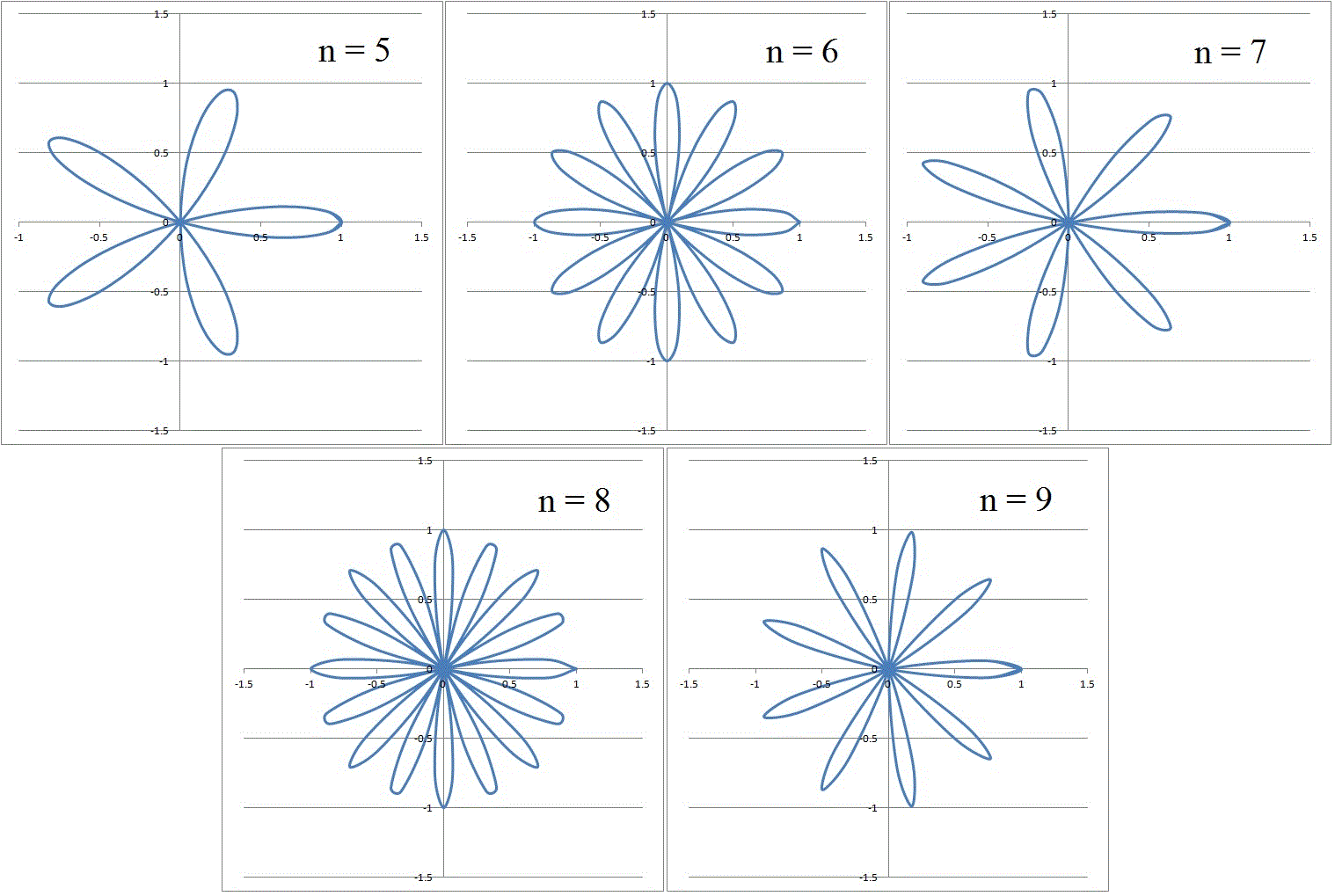
, is a family of images of flowers (roses) of
or
petals depending on
being even or odd.
The collection of polar graphs,
, is a family of images of flowers (roses) of
or
petals depending on
being even or odd.
Out of the options given , which rose with that number of non-overlapping petals would have the greatest total area?
Image Credit: Flickr David Gilson .
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Very interesting problem!
We can integrate the polar equation for the area of exactly one petal, as follows
∫ − 2 n π 2 n π 2 1 ( C o s ( n t ) ) 2 d t = 4 n π
so that if there are n petals, the total area is 4 π , and if there are 2 n petals, it's 2 π . Hence, for the number of petals given as choices 5 , 6 , 7 , 8 , 9 , we should look at 6 and 8 as candidates. However, the 6 petal version, requiring that n = 2 3 , overlaps, which makes moot its total area. Ergo, the answer is 8 . Here are the 6 and 8 rose petal curves