A Simple but Convoluted Pendulum.

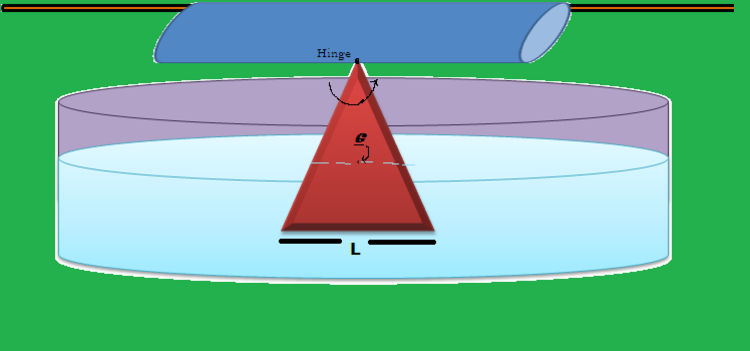The arrangement shown is of a Laminar Equilateral Triangle which is hinged to the surface of a cylinder whose radius $r=\frac{L}{\sqrt{3}}$ which rests on a smooth very thin fixed rod as shown.

The arrangement is dipped in a large stationary beaker containing a liquid of density one tenth of that of the lamina such that the centroid of the equilateral triangle lies just below the liquid surface.The length of the triangle is L.Initially the whole arrangement is in equilibrium. When we displace the lamina by a very very small angular displacement along an axis which perpendicular to the plane of triangle as well as perpendicular to the axis of cylinder it undergoes oscillations with a time period of $T=\pi s$ .The distance of the centre of mass of the whole system of lamina and cylinder is 20m from the rod .The cylinder is a right circular cylinder whose axis is in the plane of the triangle. In the whole process assume that the centroid of the triangle is approximately on the liquid surface. Assume that the water is always steady.Assume that the cylinder is free to slide on the rod and the lamina can freely rotate about the hinge about the above mentioned axis. Take the value of

$g=10\frac{m}{s^{2}}$

$L=\frac{a}{b\sqrt{c}}$

where c is prime a and b are integers then a+b is.