A simple triangle-semi-circle problem

△ A B C has sides of lengths 5 , 7 and 8 . You draw a semi-circle on the longest side touching the 5 and 7 sides, as in the figure above. Find the radius of the semi-circle. If the radius can be written as b a , where b is square-free, then enter a + b .
The answer is 8.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Hmmmm, interesting. I'm not aware of the formula Area of a triangle = 2 1 × ( sum of 2 of the triangles’ lengths ) × ( radius of the semicircle inscribed in said triangle, tangent to both the chosen sides, and its diameter lie on the remaining side )
What a mouthful!
Got a proof for it?
The proof is very straightforward. Connect the center of the semi-circle (point D) with point B with a line segment. This line segment divides △ A B C into two smaller triangles. Since the the semi-circle is tangent to AB (say at point E) and to BC (say at point F), then DE is equal to the radius in length and is perpendicular to AB, and the same can be said as DF, its length is R, and it is perpendicular to BC. Thus in △ A D B the base is A B and the height is DE = R, and similarly in △ D B C the base is BC and the height is DF = R, and this implies that
Area of △ A B C = Area of △ A D B + Area of △ D B C = 2 1 ( A B + B C ) R
Q . E . D .
Reflect the triangle about the line AC to form a tangential quadrilateral (a kite, specifically). Then solve for the radius of the inscribed circle.
inradius = s K as shown here
where K is the area of the quadrilateral (twice the area of the triangle) and s is its semiperimeter.
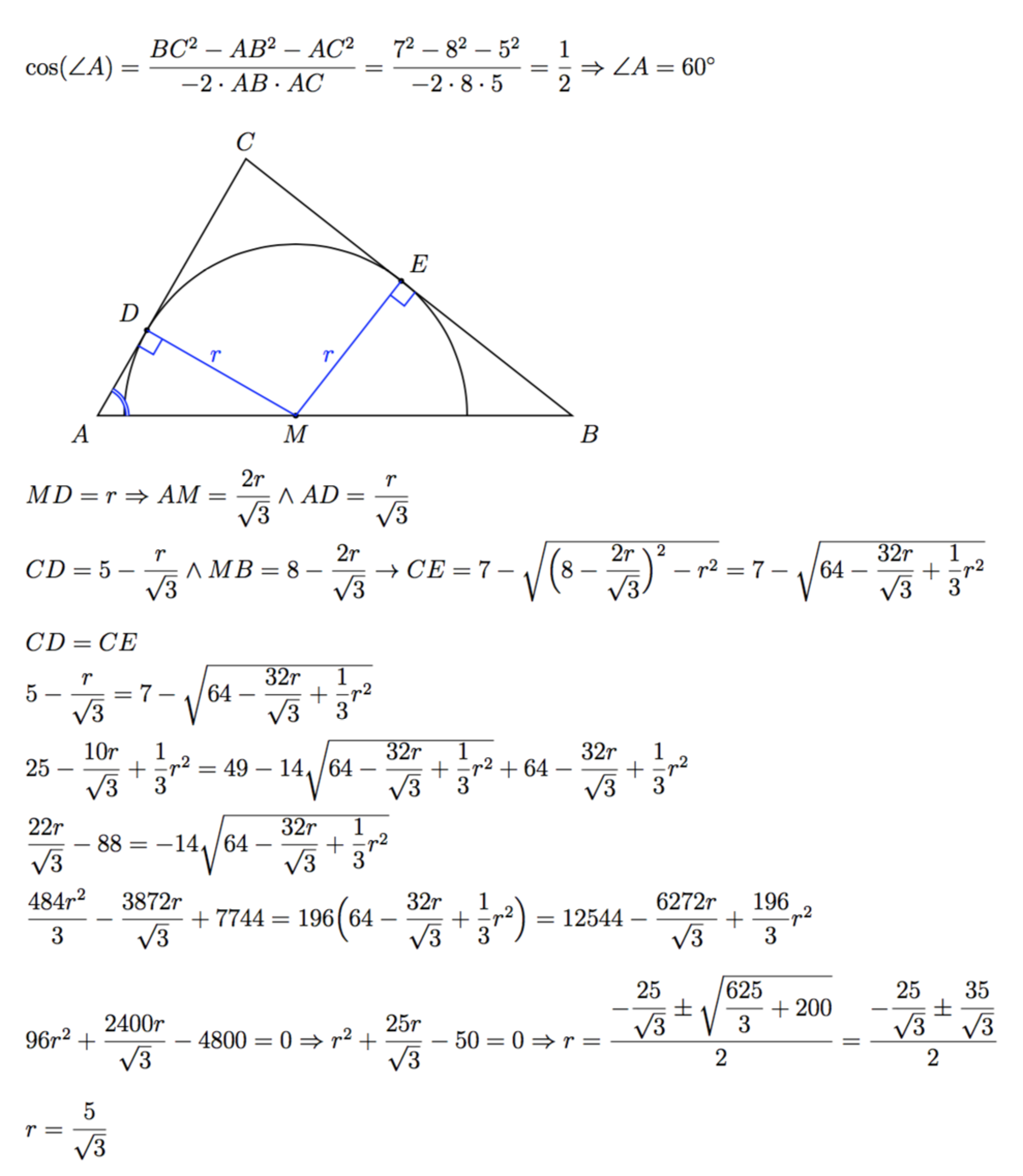
The area of △ A B C by Heron's formula is A = s ( s − a ) ( s − b ) ( s − c ) , where s = ( 1 / 2 ) ( 8 + 5 + 7 ) = 1 0 . Thus, A = 1 0 ( 2 ) ( 5 ) ( 3 ) = 1 0 3 . A , on the other hand, is given by A = ( 1 / 2 ) R ( 5 + 7 ) = 6 R , so R = 3 5 3 = 3 5 .