A solid from triangles

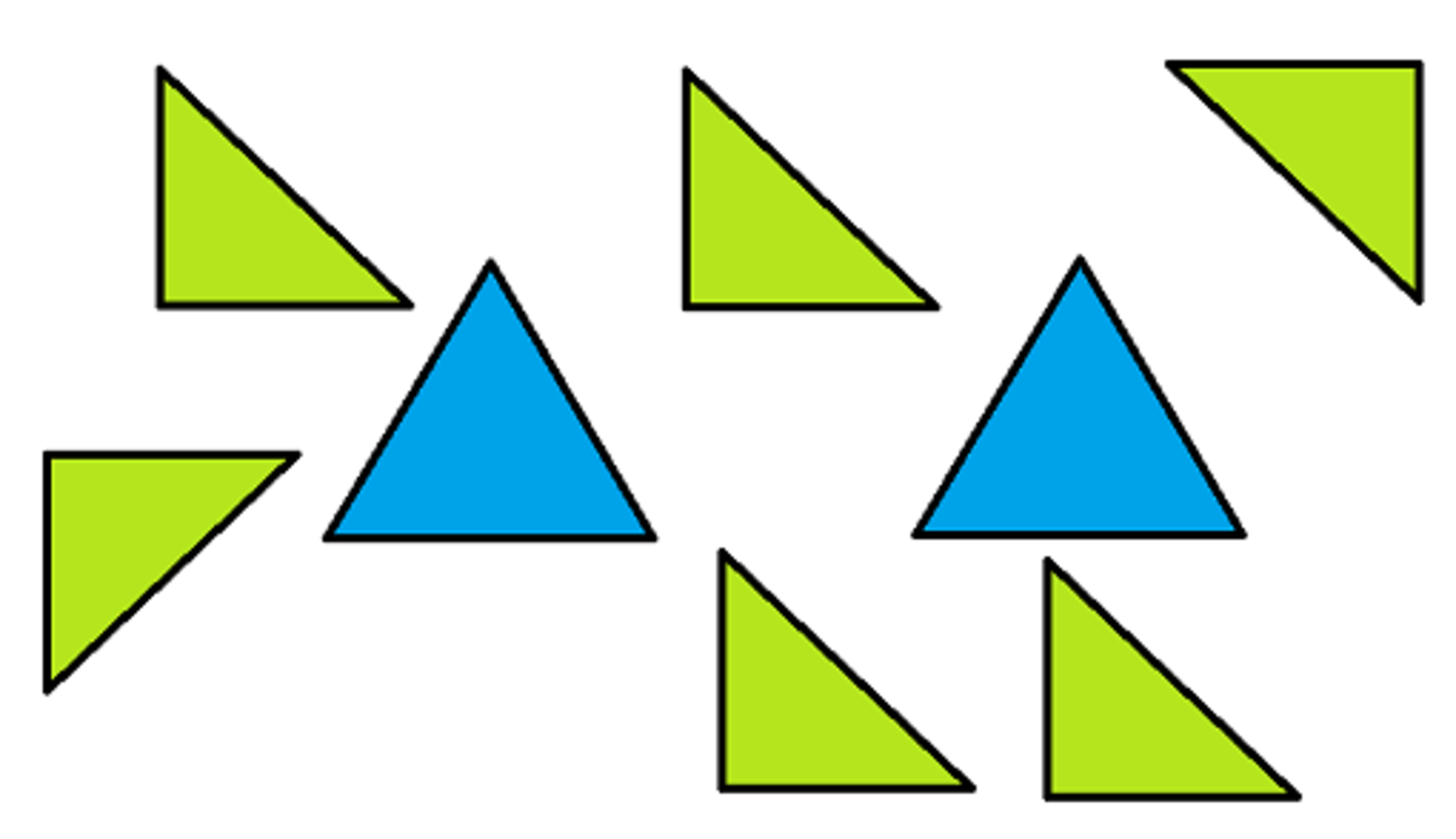
You have the following 8 triangles:
- 2 equilateral triangles (side length 2 )
- 6 isosceles triangles (side lengths 1 , 1 , 2 )
If you connect them at their edges to form a solid, such that the two equilateral triangles don't share an edge, what is the volume of the resulting solid?
The answer is 0.667.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
I created a different solid with the same configuration of sides.
 intriguing Solid
Is this the same as Marta's?
intriguing Solid
Is this the same as Marta's?
Log in to reply
Actually this is the same solid... It may not have been clear from my picture, but it only shows one of the tetrahedra cut off... When you cut the second one off the opposite corner you get your solid pictured here!
Log in to reply
I was misled by orientating the solid so that one of the equilateral triangles was the base.
Result is a triangular anti-prism with bases side a = 2 . Regular triangular anti-prism is an octahedron with volume (for edge a = 2 )
V
=
3
2
a
3
=
3
4
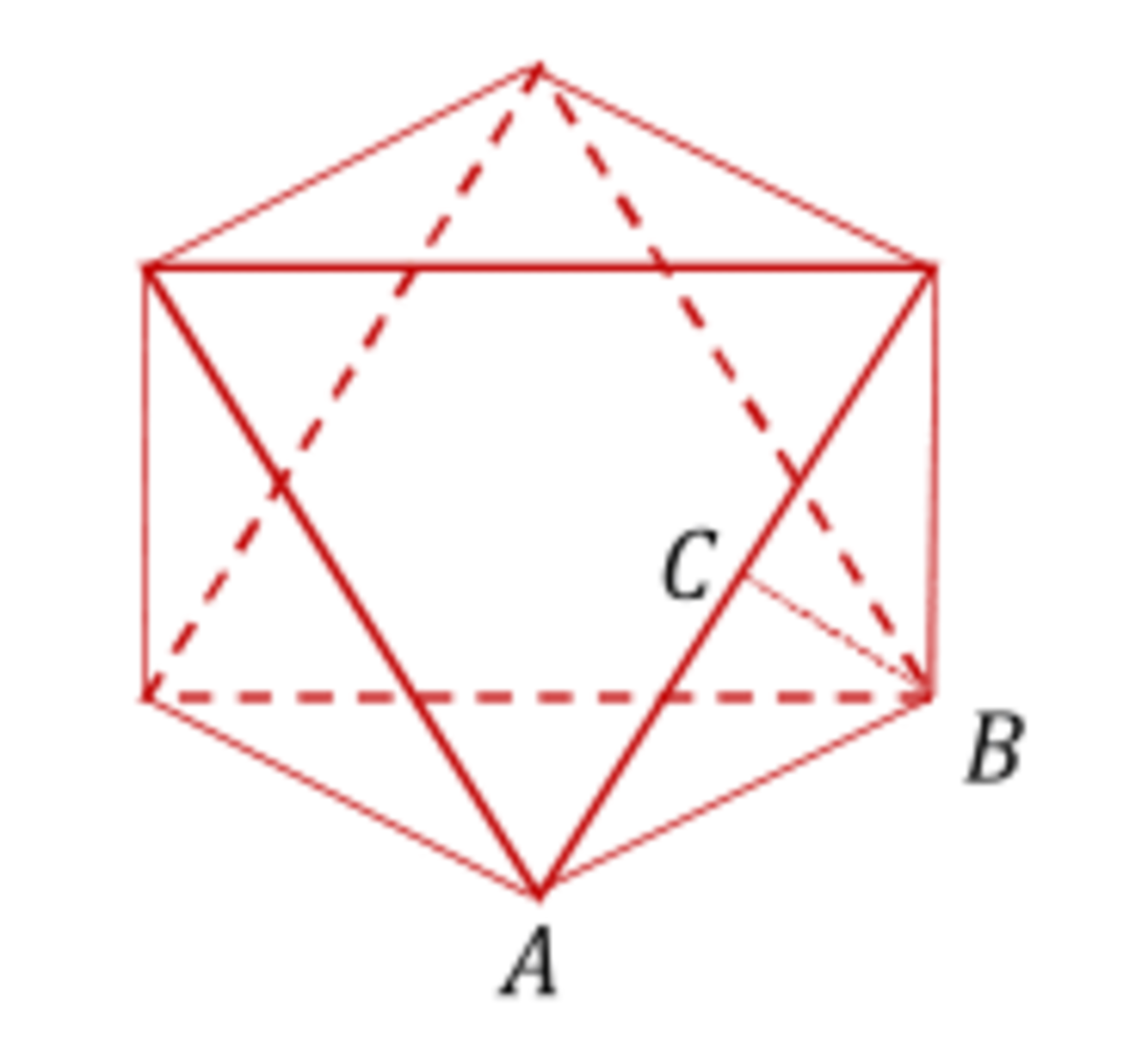 In top view
A
C
=
2
1
,
∠
A
C
B
=
9
0
∘
,
∠
C
A
B
=
3
0
∘
therefore
A
B
=
3
2
In top view
A
C
=
2
1
,
∠
A
C
B
=
9
0
∘
,
∠
C
A
B
=
3
0
∘
therefore
A
B
=
3
2

With side edge a = 2 the height would be h 2 = 2 − A B 2 = 2 − 3 2 = 3 2
With side edge a = 1 the height is instead h 1 = 1 − A B 2 = 1 − 3 2 = 3 1
So we need to take the volume of a regular tetrahedron and multiply it by the ration of the heights.
V = 3 4 × h 2 h 1 = 3 4 × 2 1 = 3 2
@Marta Reece Interesting approach... But I think that is the volume for a regular octahedron... This octahedran isn't quite regular since its faces aren't all equilateral triangles.
In fact, its volume is exactly half the area of a regular octahedron.
Log in to reply
Still working on it. It didn't want to give me preview without posting first. Sorry.
Log in to reply
Ah ok, got it... Figured it might be something like that... (Since you got the answer correct! :0) )

The isosceles triangles are 45-90-45 with unit sides.
The resulting solid is a unit cube with two tetrahedral corners cut off. Here is an image of one of them:
Since the areas of those tetrahedral corners are each 6 1 then the volume of the resulting cube will be given by:
V = 1 − 6 1 − 6 1 = 3 2