A squeezed in circle!

Geometry Level pending

In a square with side 32, two-quarter circles are drawn. Another smaller circle is drawn such that it is tangent to the quarter circles and the square. What is the radius of the smaller circle?


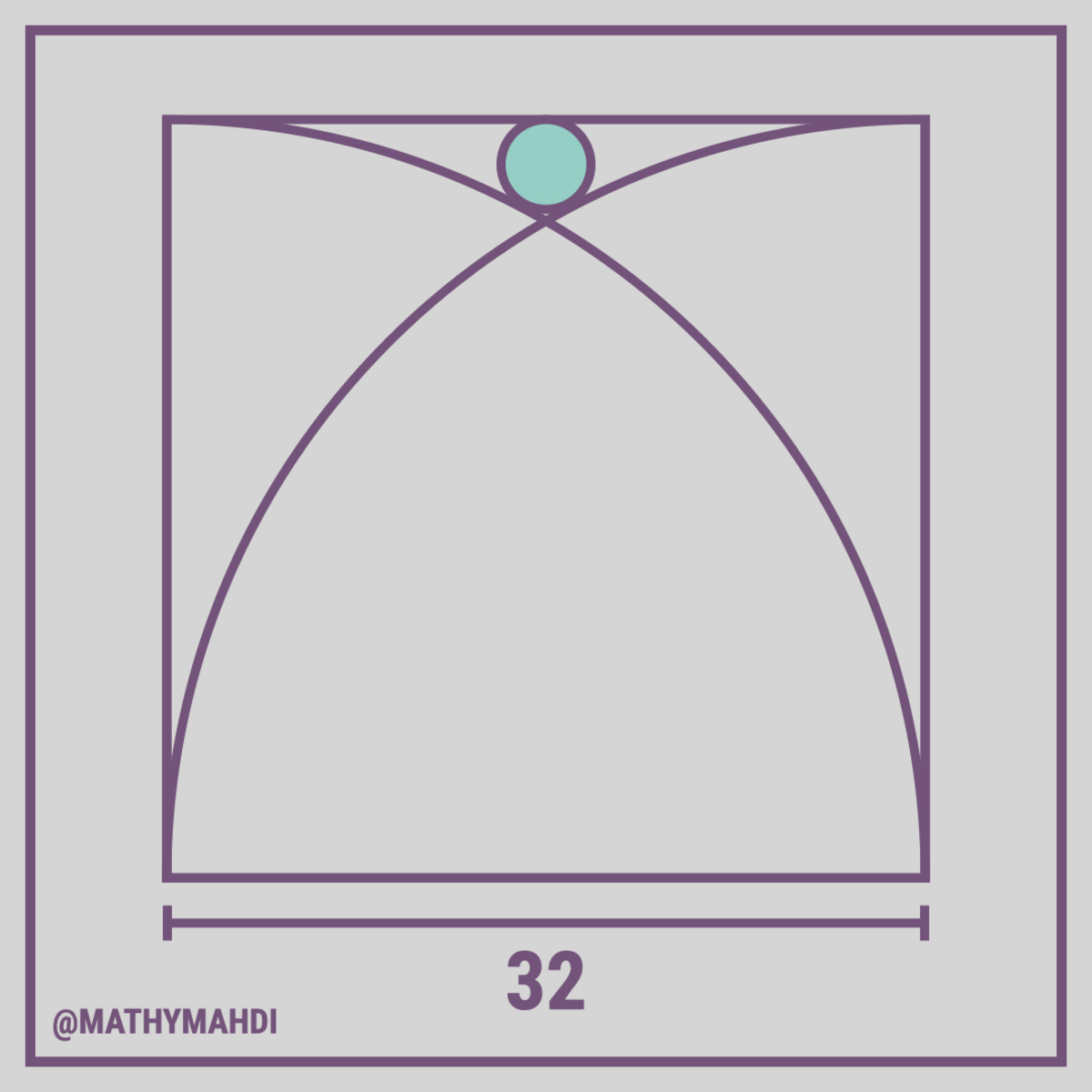
The answer is 2.

Mahdi Raza by Mahdi Raza , 16, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Chris Lewis
Jun 8, 2021

Say the radius of the circle is r r and its centre C C . Let A A be one of the vertices of the bottom edge of the square and M M its midpoint, so Δ A M C \Delta AMC is a right-angled triangle: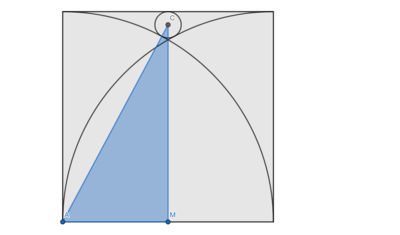

We have A M = 16 AM=16 , A C = 32 + r AC=32+r and M C = 32 − r MC=32-r ; so by Pythagoras, A C 2 − M C 2 = A M 2 ( 32 + r ) 2 − ( 32 − r ) 2 = 1 6 2 64 ⋅ 2 r = 256 r = 2 \begin{aligned} AC^2-MC^2 &=AM^2 \\ (32+r)^2-(32-r)^2 &=16^2 \\ 64\cdot 2r &=256 \\ r &= \boxed{2} \end{aligned}

4 Helpful 1 Interesting 2 Brilliant 0 Confused

Thanks for all the problems, Mahdi!

Chris Lewis - 4 days, 11 hours ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...