A Student Who Did The Impossible With Toilet Paper
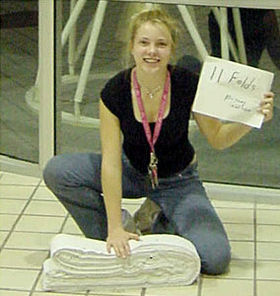 In 2002, a high school student, Britney Gallivan, folded a single piece of 0.1 mm-thick paper completely in half 12 times, breaking the world record at the time.
In 2002, a high school student, Britney Gallivan, folded a single piece of 0.1 mm-thick paper completely in half 12 times, breaking the world record at the time.
Using just the information given above, can you approximate the height of Britney's final folded paper?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
35 solutions
I did a small miscalculation and that's why ended up doing 2 n − 1 instead of 2 n . And hence I got the wrong answer! ^^
yes,i do the same with your solution
the answer in the problem should be approximately 41 cm but since there is no 41 in the choices, the nearest possible answer is 40
me, instead of raising 2 o the 12th power then multiply to 0.1, what i did is.. (0.1)(2*12)..ahaha thats why my answer is wrong
same here
opps forgot 2 change in cm..
Log in to reply
I hate your profile pic.
Log in to reply
dear i don't apply my profile pic for you to like it fellow
http://bit.ly/1hZBQ1b
here it is asked that she folded the paper 12 times so the series started from 0.2+0.4+0.8+1.6... and sum for twelve term becomes 819 mm or 81.9 cm
Number of layers after one fold = 2 = 2 1
No of layers after 12 folds = 2 1 2 = 4096
Thickness of one layer = 0.01 cm
Thickness of 4096 layers = 4096 × 0.01 = 40.96
hence, answer = 4 0
Great answer!
The stack doubles in thickness for every fold, so the final thickness will be 0 . 1 × 2 1 2 = 4 0 9 . 6 mm , which is approximately 4 0 cm
nice solution
Every time she folds the paper, its thickness doubles. So the thickness of the final folded paper will be 2^12 times the thickness of single sheet of paper.
i answerd it it will be 40
Let the paper she folded had a thickness a = 2 0 a
At 1 -st folding, the thickness is 2 a = 2 1 a
At 2 -nd folding, the thickness is 4 a = 2 2 a
At 3 -rd folding, the thickness is 8 a = 2 3 a
.
.
.
At n -th folding the thickness is 2 n a .
Given that a = 0 , 1 m m and it folded 1 2 times.
Then the thickness is 2 1 2 x 0 , 1 m m = 4 0 9 , 6 m m = 4 0 , 9 6 c m
So, the closest to the thickness if the final folded paper is 4 0 c m
Every time the paper is folded, the thickness of the paper is double the previous thickness.
For example, if the paper is x thick, then after it is folded for one time, it becomes 2x.
When it is folded again, it becomes 2(2x)=4x
When it is folded again, it becomes 2(4x)=8x
You get the idea.
In mathematics, this is a geometric progression, with the common ratio 8x/4x = 2, hence the nth term that represents the number of fold at the instant is T=(0.1 x 10^-3)(2)^n
(nth term in Geometric Series, T=ar^n, where a is the first term, r is the common ratio (T n/T (n-1)), and n is the number of term)
Thus, for n=12,
T=(0.1 x 10^-3)(2)^12=0.4096=40.96cm
Which is approximately 40cm.
This is my initial thinking before the geometric realization.
I don't have a calculator at the moment, so I did the calculation in the computer notepad. Here is what I wrote.
1 - 0.2mm
2 - 0.4mm
3 - 0.8mm
4 - 1.6
5 - 3.2
6 - 6.4
7 - 12.8
8 - 25.6
9 - 51.2
10 - 102.4
11 - 204.8
12 - 409.6mm - 40.96cm
Note that I just double the number for every fold made.
I have used geometric progression to solve this.
i HAVE USED MY TOILET PAPER TO solve this.....;p
Log in to reply
Good Job! Finally you figured it out! Attention to all users ' Arya Samanta' has used toilet PRACTICALLY to solve this !
double the thickness for each time..
0.1--> 0.2{first fold} -->0.4 (2nd fold) -->0.8 -->1.6 -->3.2 -->6.4 -->12.8 -->25.6 -->51.2 -->102.4 -->204.8 -->409.6(12th fold)
So after 12 folds The thickness of paper is 405.6 mm., we knew 1cm=10mm.,
so thickness = (405.6/10)cm = 40.56 cm
102.4-->204.8-->409.6mm means 40.96cm
In my view it should be started with 0.2 and go on like this 0.2,0.4,0.6,.....making a GPseries with first term0.2 and common ratio2
- For Every one fold the layer of Paper is Doubled.
- i.e, for 12 times the no. of Layer on upon the other will be 2 1 2 = 4096.
- Hence total width = 4096 X .1 mm.
- 40.96 cm ~ 40 cm.
the thickness after 12 turns =2^12*0.1mm= 40cm(approx)
Let the thickness per sheet of paper = x, When we fold half the thickness=2x Again fold half thickness=4x =2^{2}x , Again Fold Half thickness=8x=2^{3}x.................Similarly At the last fold i.e. 12th times the thickness=2^{12}x=4096x But By The question,thickness per sheet of paper=.1mm i.e. x=.1mm So THICKNESS=4096*.1mm= 40.96cm.....
its (2^12)*0.1 = 409.6 mm or 40.96 cm the closest answer among the answers is 40 cm.
This folded paper will have 2^12=4096 folds which will have a height of 40.96 c. m. which approximately equals to 40 c. m.
0.1*2 (raised to power 12) = 40.96cm
its pretty simple. Every time you fold the paper the thickness doubles, so basically its a G.P . with common ratio 2 : 1/10, 2/10, 4/10 and so on. On the 12th fold, it is 2^12/10 mm, or 2^12/(10*10) cm, which is 40.96 . Hence the answer is 40 .
Yeah. It's r8.
2^12 X 0.1 m.m=40.96 c.m ---- which should be rounded off to 41 c.m instead of 40 c.m....i just picked the closest!
We should first understand that while folding the toilet paper, after each fold, the height becomes double of the previous height. This is because the half part of the same paper is folded on top of the other half and since both halves have same height, the resultant height after the fold is double of its previous height. Since, the paper was folded 12 times, we have the height h as :
h = ( 0 . 1 × 2 1 2 ) mm = ( 0 . 1 × 4 0 9 6 ) mm = 4 0 9 . 6 mm = 4 0 . 9 6 cm
So, the close approximation of the height of the toilet paper after folding 12 times = 4 0 cm
Given thickness of each layer is 0.1mm=0.01cm
n folds will give 2^n layers of paper..
Hence,the total height will be 2^n * 0.01
Here,n=12
The Total Height will be 2^12 *0.01=4096/100=40.96( Approx. 40)
Everytime the paper is folded, the thickness doubles. As the initial height was 0.1, after 12 folds the height would be 0 . 1 × 2 1 2 = 4 0 9 . 6 m m ≈ 4 0 c m
for twelve half folds(6 full folds) it means from each side it will be 2 times so 4 times the height from both sides. for first fold 0.1 4=0.4; fir second fold 0.4 4=1.6; for third fold 1.6 4= 6.4; for fourth fold 6.4 4 =25.6; for fifth fold 25.4 4 =102.4; and for last fold 102.4 4=409.6 mm 409.6 mm=40.96 cm so approximatly 40 cm
0,1mm x 2^12 = 409,6mm = +/- 41 cm
Every time you fold this paper, it doubles in thickness. So the answer is 2 to the 12th. But because it has to be in centimeters, you divide by 100, because 2 to the 12th is how many 0.1 mm you have. So the answer is 40.96, or approximately 40.
The problem can be written as 0 . 1 × 2 1 2 , where 0 . 1 is the thickness of the paper in millimeters and 2 1 2 , the height of the paper after folding it twelve times. So,
0 . 1 × 2 1 2
= 0 . 1 × 4 0 9 6
= 4 0 9 . 6
Converting millimeters to centimeters,
4 0 9 . 6 m m = 4 0 . 9 6 c m
The best estimate is 4 0 centimeters.
For each fold, height will be double than previous fold.
After the first fold, h e i g h t = 2 × ( p a p e r t h i c k n e s s ) = 2 1 × ( p a p e r t h i c k n e s s )
After the second fold, h e i g h t = 2 × ( h e i g h t o f t h e f i r s t f o l d e d p a p e r ) = 4 × ( p a p e r t h i c k n e s s ) = 2 2 × ( p a p e r t h i c k n e s s )
After the third fold, h e i g h t = 2 × ( h e i g h t o f t h e s e c o n d f o l d e d p a p e r ) = 8 × ( p a p e r t h i c k n e s s ) = 2 3 × ( p a p e r t h i c k n e s s )
Thus, after the n t h fold, h e i g h t = 2 n × ( p a p e r t h i c k n e s s )
Hence, after the 1 2 t h fold, h e i g h t = 2 1 2 × ( p a p e r t h i c k n e s s ) = 2 1 2 × 0 . 1 m m = 4 0 9 . 6 m m = 4 0 . 9 6 c m
Therefore, h e i g h t = 4 0 c m ( a p p r o x i m a t e l y )
each time folded, the thickness is doubled. so for "n" folds, the height = (2^n)*thickness
summing up a GP series....
so simple every time she fold it double so 1st time 2 fold=2^0 2nd time 4 fold=2^2 3rd time 8 fold =2^3 so 12th time 2^12=4096 i fold is 0.1 mm so 4096 fold 409.6 mm= 40.96 cm, so the closest answer is 40cm
x=(2^12)*0.1 x=409.6 mm
x=409.6 mm/10 mm x=40.96 cm
so we have 0.1 that doubles 12 times . We can write it as 0.1 x 2^12 . which gives us 409.6 mm . Converting it into cm we get 40.96 and rounding it we get 40 cm
i think rounding the answer up will give 41 cm....
The final thickness will be 2 1 2 = 4 0 6 9 × 0 . 1 m m = 4 0 . 9 c m
One fold for every paper that has been doubled.
i.e, for 12 times the no. of Layer on upon the other will be 2 1 2 = 4096
Final Thickness = 4 0 9 6 × 0 . 1
4 0
juz find out 2 raised to 12
Since the thickness doubles on each fold and there are 12 such folds.
We can approach it by=== 2 to the power 12 * 0.1mm
1 fold----> 2 layers= 2^1 layers 2 fold----> 4 layers=2^2 layers . . . 12 folds----->2^12 layers. So, height= (2^12)*0.1 mm
The thickness of the paper doubled on every fold. So, after n folds the thickness of the finally folded paper would be 2^n times the original thickness making the answer as = (2^12)*0.1 mm = 409.6 mm = 40.96 cm.
By the way, with proper rounding off the number to make it an integer, the answer should have been 41 cm instead of 40 cm !!!
http://academic.brooklyn.cuny.edu/geology/leveson/core/linksa/roundoff.html