Absolutely absolute

Algebra Level 4

Solve the equation

$\left| 2x-3y \right| +\sqrt { 7x-3y-13 } =0$

Given that the value of $x$ and the value of $y$ can be expressed as $\frac{a}{b}$ and $\frac{c}{d}$ respectively and that they are both real, find the value of $a+b+c+ d$

If you think that there are infinitely many rational solutions for $x$ and $y$ , input $0$ as your answer.

If you think that there are no solutions for $x$ and $y$ , input $-1$ as your answer.

The answer is 59.

3 solutions

Julian Poon
Feb 13, 2015

Notice that $\left| 2x-3y \right|$ is always positive and real and $\sqrt { 7x-3y-13 }$ is always non negative if it is real.

Since both sides are to be positive if $x$ and $y$ are real, then

$2x-3y =0$ $7x-3y-13=0$

Solving for these simultaneously gives

$x=\frac{13}{5}$

$y=\frac{26}{15}$

Therefore, $a+b+c+d=13+5+26+15=\boxed{59}$

You should use the word non-negative instead of positive in your solution since $0\notin \mathbb{R^+}$ , where $\mathbb{R^+}$ denotes the set of positive real numbers.

Prasun Biswas - 6 years, 3 months ago

Niranjan Khanderia
Mar 3, 2018

Tín Phạm Nguyễn
Mar 2, 2015

The equation can be rewritten as follows:

$|2x-3y|=-\sqrt {7x-3y-13}$

For all real x and y, it is obvious that $LHS \ge 0$ and $RHS \le 0$

Therefore: $LHS = RHS$ if and only if both equalities occur.

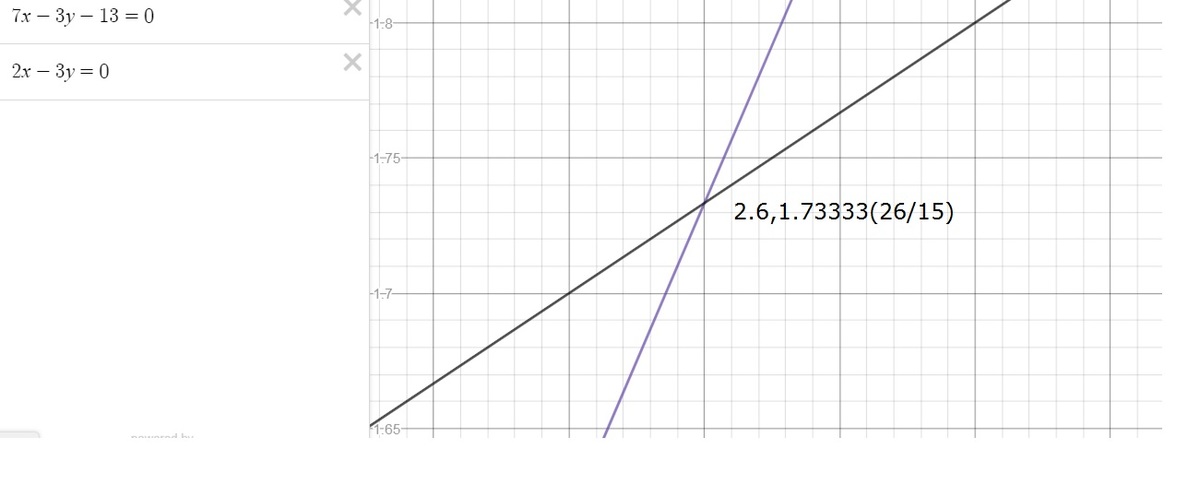
$\left\{ \begin{array}{l} 2x - 3y = 0 \\ 7x - 3y - 13 = 0 \\ \end{array} \right.$

$\left\{ \begin{array}{l} x = \frac{{13}}{5} \\ y = \frac{{26}}{{15}} \\ \end{array} \right.$

Therefore, $a+b+c+d = 26+15+13+5=\boxed{59}$

Absolutely absolute

The answer is 59.

3 solutions

0 pending reports