Adding to 15

Reading the question, we find that this is a game. Obviously, the first thing everyone solving this should do is to

$\huge \text{Play the game!}$

Let's play a few variations and see what we get:

$\begin{array}{c|c} \text{Calvin} & \text{Patrick}\\ \hline 5 & 3\\ 8 & 2\\ 1 & 6\\ 9 & \text{ } \\ \end{array}$

Calvin has got the numbers $(9, 5, 1)$ which add to 15, so he won. Let's play another game.

$\begin{array}{c|c} \text{Calvin} & \text{Patrick}\\ \hline 5 & 4\\ 8 & 2\\ 9 & 1\\ 3 & 7\\ 6 & \text{ } \\ \end{array}$

This time, we find out that a draw has occurred.

Playing a few other games might make you confident that there is no winning strategy, but how would you prove it. Have a think about this before scrolling down.

---

Anyone think up a way of solving this? This was my method below:

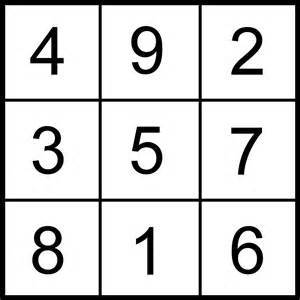
Notice that this game is equivalent to playing Tic-Tac-Toe on a 3x3 magic square! Let's prove this statement.

The number of ways of writing 15 as the sum of three distinct integers less than 10 can be counted as below:

$\begin{array}{ccccc} 15= & 1+5+9, & 2+4+9, & 3+4+8, & 4+5+6,\\ \text{ } & 1+6+8, & 2+5+8, & 3+5+7, & \text{ }\\ \text{ } & \text{ } & 2+6+7, & \text{ } & \text{ }\\ \end{array}$

Thus, there are 8 ways of doing so. Furthermore, in a magic square, there are 8 rows, column and diagonals that add up to 15, each of which is distinct. Thus, all sums of distinct integers under 10 that add up to 15 are present in a 3x3 magic square.

To prove that this is a drawn game, we notice that Tic-Tac-Toe is also a drawn game if played optimally. Using this fact, we get that there is no winning strategy.

Here is a diagram of the magic square:

$\begin{array}{|c|c|c|} \hline 4 & 3 & 8\\ \hline 9 & 5 & 1\\ \hline 2 & 7 & 6\\ \hline \end{array}$

The first game would have the Tic-Tac-Toe representation of (where the $X$ is Calvin and the $O$ is Patrick)

$\begin{array}{|c|c|c|} \hline \text{ } & O & X\\ \hline O & X & X\\ \hline O & \text{ } & X\\ \hline \end{array}$

The second game would have the Tic-Tac-Toe representation of

$\begin{array}{|c|c|c|} \hline O & X & X\\ \hline X & X & O\\ \hline O & O & X\\ \hline \end{array}$

(Try to overlay the diagrams over the magic square. I, unfortunately, couldn't get that to work because Brilliant doesn't allow the \xcancel command)

The cool part of this problem is when you realize you are just playing a regular game of Tic Tac Toe. Imagine if the numbers were laid out as a magic square:

and the winner is the first to pick 3 numbers that are in a row. All the combinations that add up to 15 are included in the magic square, which can be counted as follows:

• All the combinations which include 1: 1-5-9, 1-6-8
• All the combinations which include 2: 2-6-7, 2-5-8, 2-4-9
• All the combinations which include 3: 3-5-7, 3-4-8
• All the combinations which include 4 (but nothing lower): 4-5-6

And, as is well known for that game $\boxed{\mbox{neither player}}$ can guarantee a win.