Airborne Biker

Classical Mechanics Level 1

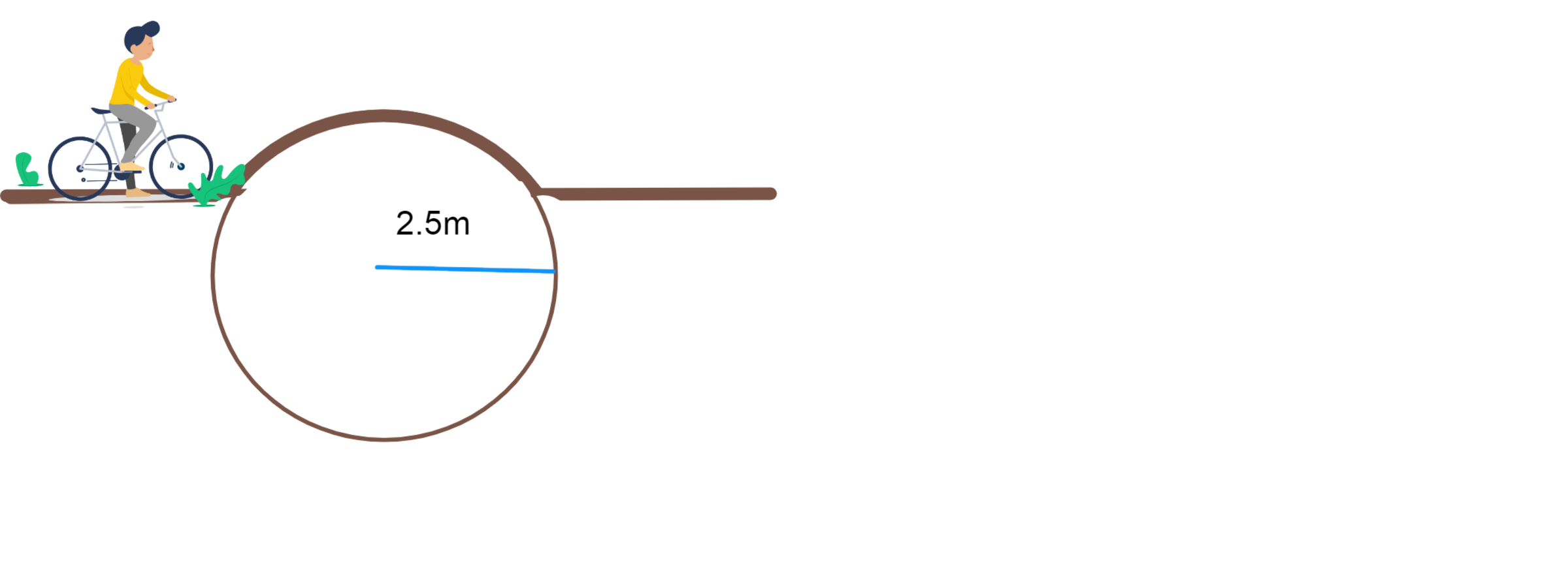
Assuming that the biker has a mass of $100$ $\text{kg}$ and $g$ is $10$ $\text{m/s}^{2}$ , how slow can he travel whilst still becoming airborne after he hits the bump?

$\text{You need to find the velocity}$

The answer is 5.

1 solution

Shane Sarosh
May 2, 2021

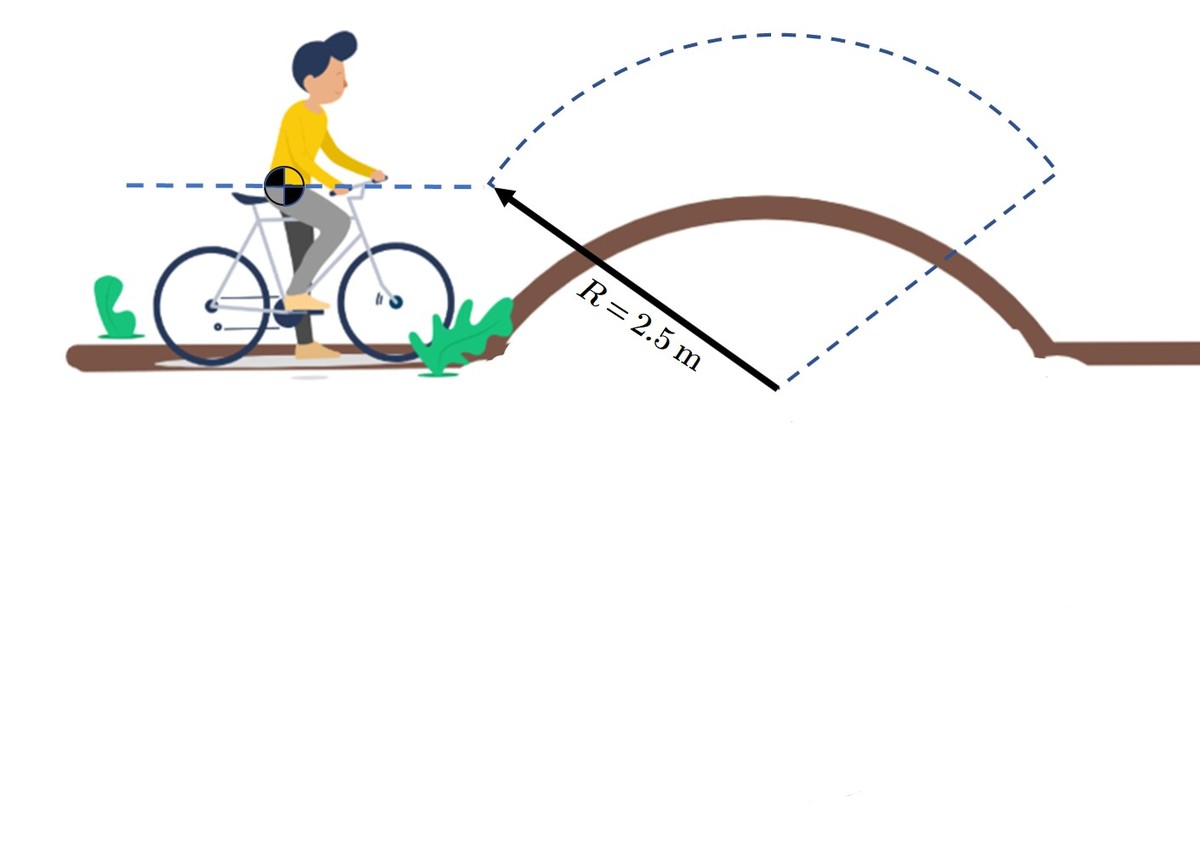
In order for the bike to become airborne, the normal force will have to equal 0.

$a_c = \dfrac{\sum F}{m}$ $\displaystyle{\dfrac{v^{2}}{r} = \dfrac{mg - F_n}{m}}$ $\displaystyle{\dfrac{v^{2}}{r} = \dfrac{mg - 0}{m}}$ $\displaystyle{\dfrac{v^{2}}{2.5} = \dfrac{1000}{100}}$ $\displaystyle{\dfrac{v^{2}}{2.5} = 10}$

$v = \sqrt{(2.5)(10)}$ $v = \boxed{5 \text{m/s}}$

Hey Shane, Good problem. Do you mind if I borrow your picture, and ask a follow up with this linked as the inspiration?

Eric Roberts - 1 month ago

Sure, go ahead!

Shane Sarosh - 1 month ago

Thanks Shane, In writing up my question I think I have come across a bit of an inaccuracy that applies to this question as well that I hadn't given consideration when solving it. The centripetal acceleration acts on the CoM of the Rider+ Bike, which means that 2.5 m radius of the bump can be used only if we consider the Bike and Rider to be a point mass with no dimension exactly following the brown path or a center of mass path offset from it. Either way, I think that diagram/wording might need to be adjusted to reflect this.

Eric Roberts - 1 month ago

If you update the picture to this it should be consistent.

Eric Roberts - 1 month ago

Airborne Biker

The answer is 5.

1 solution

0 pending reports