Perfect train. Perfect speed. Perfect Tunnel.
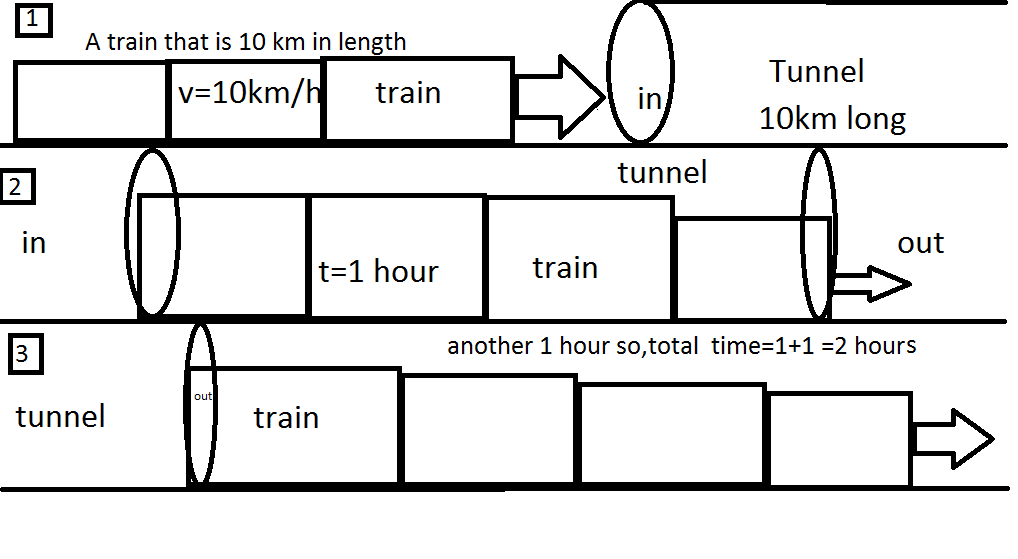
A train that is 10 km in length traveling at 10 km/hour is about to enter a tunnel that is 10 km long.
How long will it take for the entire train to get through the tunnel, from when the front of the train enters the tunnel to when the end of the train exits the tunnel?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
12 solutions
I totally get it. Thanks!!!
That is the simplest way of looking at it.
Correct. Nothing can be simpler
Relevant wiki: 1D Kinematics Problem Solving
Given that,
-
Tunnel length = 10 km
-
Speed of the train = 10 km/h
The time will take for the front and back of train to get through the tunnel= 1 hour.
The time will take for the back of the train to reach the tunnel = 1 hour
So the entire train will take 1 + 1 = 2 hours to get through the tunnel,
I thought that since the train is 10 km long and travels at 10 km per hour it would take it 1 hour to get thought the tunnel. But then I saw Arjen Vreugdenhil's reasoning and it made more sense to me. I understood that the train needs to rake one hour to get in and one hour to get out.
Log in to reply
The trick is that we compare times for the front and for the back of the train. Because you are looking at two different entities, the simple reasoning (time = distance / speed) does not work.
It says A train that is 1 0 km in length traveling at 1 0 km/hour is about to enter a tunnel that is 1 0 km long. because the tunnel is 1 0 km long the train speeds is 1 0 km per hour means the velocity of it is 1 0 km in 1 hour, so,
After 1 hour we will see its head( the front of the train ) on the exit point of the tunnel but the tail of that train (the back of the train) will remain on the entry point. So It will take another 1 hour to see its tail on the exit point. So It will take 2 hours to see the train totally out of that tunnel .
This is nice. I like your illustration.
It takes one hour to get the train in and one hour to get the train out.
if the train exits the tunnel, then it has to pass through the tunnel with its whole body.
so, after passing 10 km, the exit pole will be seen,but the whole train is still under the tunnel.so,the train has to move another 10 km to remove its body from the tunnel.
now, total path= 1 0 + 1 0 = 2 0 km, the train goes 10 km in 1 hour.so, it will take 2 hours to exist the tunnel fully.
nicely explained!
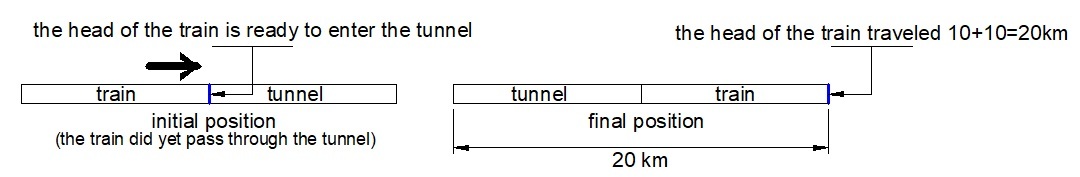
V = s p e e d ; d = d i s t a n c e ; t = t i m e
t = V d
d = length of the train plus length of the tunnel = 1 0 + 1 0 = 2 0 k m
Substituting, we get
t = 1 0 2 0 = 2 h o u r s
but why should we add the lengths?
Log in to reply
because if we do not add the lengths, the train is still inside the tunnel because they have the same lengths. the train must pass through the tunnel, it means that the end of the train must be exact the end of the tunnel. see my diagram.
T=D/S, T=(10+10)/10=2
I think it'd be helpful if you explained your notation a bit.
Step 1. The train is 10km long therefore it takes one hour to get to the end of the tunnel. Step 2. For the train to fully exit it needs another hour because it is 10km long. Time taken: 2 hours
start from the distance between the back of the train and the end of the tunnel (20km), so as the train moves at the same speed at the front and the back, how long does it take to run 20km at 10km/h?... thats 20/10=2
I think the most simplest solution is to make the train a simple near lengthless line perpendicular to the track(lengthless horizontally) by pulling it back from its nose to the end part. Now this simple object has to travel a distance of- 10+10=20. Now using same old equations in physics we get t= 2hrs.
The front will exit in one hour; the back end enters at the same time and exits one hour later. 1+1=2
It takes the train 1 hour to get through the tunnel but the question is asking for the entire train to get through the tunnel hence the answer is 2 hours
But isn't d = 10 km?
It takes 1 hour for the back of the train to reach the tunnel, and 1 hour for it to go through the tunnel.