All About Geometry

solution

In triangle PBC.
Using pythagoras theorem, we get $PC=5$

$PQ=BC=AD=4$

In triangle $PQC$ .

Using similarity.

$\dfrac{AE}{AB}=\dfrac{EB}{BC}=\dfrac{AB}{AC}$

$\dfrac{AE}{4}=\dfrac{EB}{3}=\dfrac{4}{5}$

We get, $AE=\dfrac{16}{5}$ and $EB=\dfrac{12}{5}$

Therefore, area of shaded part= $\dfrac{16}{5}×\dfrac{12}{5}=\boxed{7.68}$

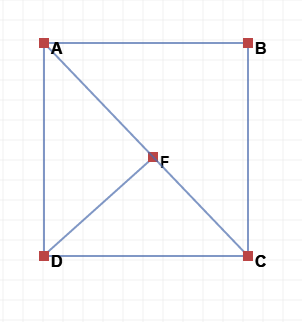The Main Objective of the Question is to find the Area of smaller Triangles.I am concentrating on the second half of the Figure , we need to find Area of $\triangle DFC$ .Now Observe that $\angle CAB$ and $\angle ACD$ are equal. Let $\angle CAB=\angle ACD=\theta$ Using Trigonometry

$\cos{\theta}=\dfrac{AB}{AC}=\dfrac{3}{5}=\dfrac{FC}{DC} \implies FC=\dfrac{9}{5} \\ \sin{\theta}=\dfrac{BC}{AC}=\dfrac{4}{5}=\dfrac{DF}{DC} \implies DF=\dfrac{12}{5} \\ \text{Area of } \triangle DFC =\dfrac12\times DF\times FC = \dfrac{108}{50} \\ \text{Shaded Area }=\text{Total Area}-2\times(Ar.\triangle ABC+Ar.\triangle DFC) \\ \implies Area = 24-2\times(6+\dfrac{108}{50})\implies 12-4.32=\boxed{7.68}$

NOTE: Due to symmetry i was concentrating on the 2nd half of the figure , because the same process can be done on the first part